Newton's Second Law

Newton's second law of motion states:

The acceleration of an object is proportional to the resultant force acting on it and inversely proportional to the object's mass

Newton's second law explains the following important principles:
- An object will accelerate (change its velocity) in response to a resultant force
- The bigger this resultant force, the larger the acceleration
- For a given force, the greater the object's mass, the smaller the acceleration experienced

Examples of Newton's Second Law

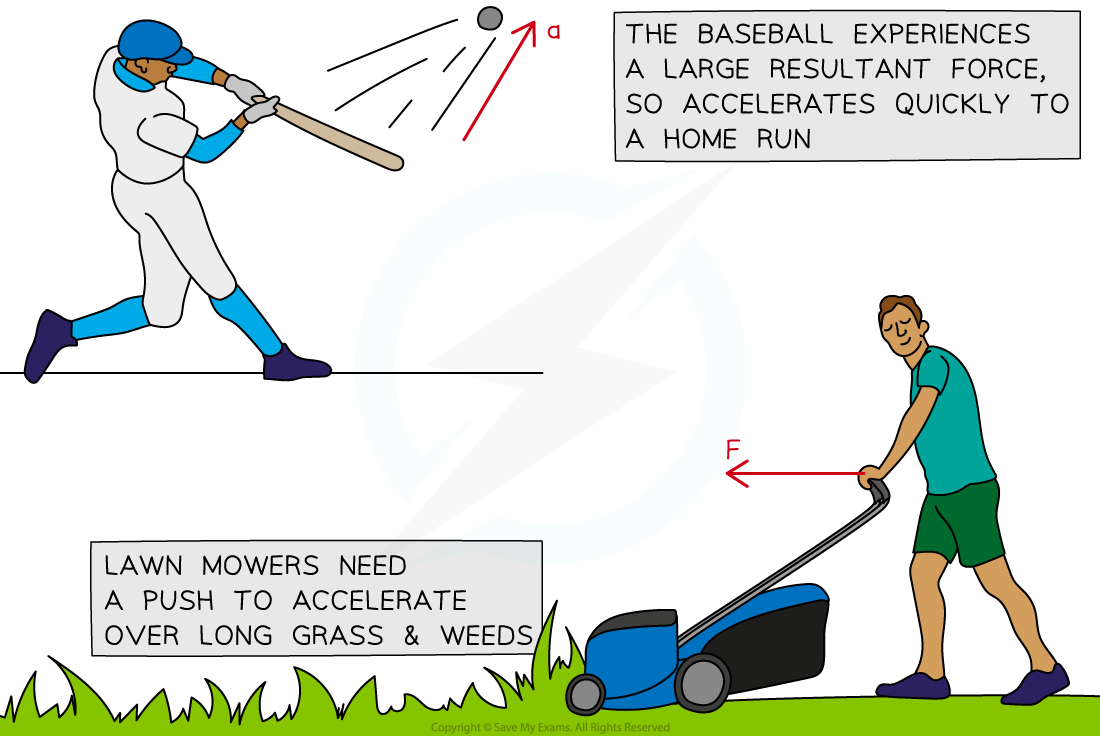
Newton second law in action, downloadable IGCSE & GCSE Physics revision notes

Objects like baseballs and lawnmowers accelerate when a resultant force is applied on them. The size of the acceleration is proportional to the size of the resultant force

Calculations Using Newton's Second Law

Newton's second law can be expressed as an equation:

F = ma

Where:
- F = resultant force on the object in Newtons (N)
- m = mass of the object in kilograms (kg)
- a = acceleration of the object in metres per second squared (m/s²)

The force and the acceleration act in the same direction

This equation can be rearranged with the help of a formula triangle:

Equation Triangle for Newton's Second Law

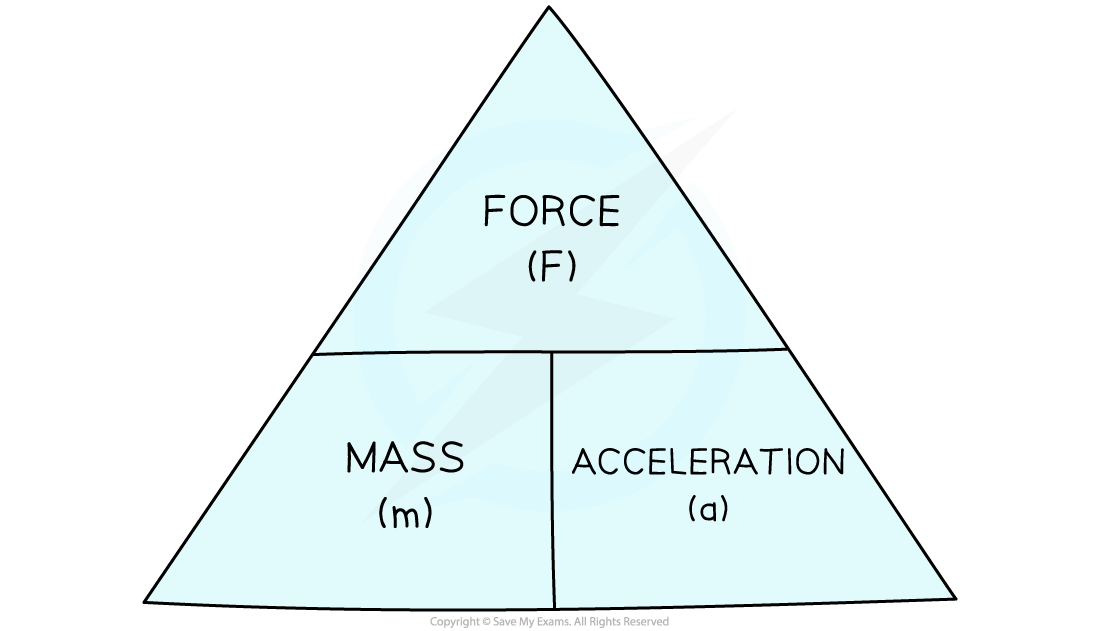
Fma Formula Triangle, downloadable IGCSE & GCSE Physics revision notes

Force, mass, acceleration formula triangle; you can use this if you need support with the rearrangement until you feel able to do it on your own

Worked example

A car salesman says that his best car has a mass of 900 kg and can accelerate from 0 to 27 m/s in 3 seconds.

Calculate:

a) The acceleration of the car in the first 3 seconds.

b) The force required to produce this acceleration.

Answer:

(a)

Step 1: List the known quantities

Initial velocity = 0 m/s
Final velocity = 27 m/s
Time, t = 3 s

Step 2: Calculate the change in velocity

change in velocity = Δv = final velocity − initial velocity

Δv = 27 − 0 = 27 m/s

Step 3: State the equation for acceleration

$a = \frac{∆ v}{t}$

Step 4: Calculate the acceleration

$a = \frac{27}{3} = 9 m / s^{2}$

(b)

Step 1: List the known quantities

Mass of the car, m = 900 kg
Acceleration, a = 9 m/s²

Step 2: Identify which law of motion to apply

The question involves quantities of force, mass and acceleration, so Newton's second law is required:

F = ma

Step 3: Calculate the force required to accelerate the car

F = 900 × 9 = 8100 N

Worked example

Three shopping trolleys, A, B and C, are being pushed using the same force. This force causes each trolley to accelerate.

WE Newton second law, downloadable IGCSE & GCSE Physics revision notes

Which trolley will have the smallest acceleration? Explain your answer.

Answer:

Step 1: Identify which law of motion to apply

The question involves quantities of force and acceleration, and the image shows trolleys of different masses, so Newton's second law is required:

F = ma

Step 2: Re-arrange the equation to make acceleration the subject

$a = \frac{F}{m}$

Step 3: Explain the inverse proportionality between acceleration and mass

Acceleration is inversely proportional to mass
This means for the same amount of force, a large mass will experience a small acceleration
Therefore, trolley C will have the smallest acceleration because it has the largest mass

Newton's Second Law (Cambridge O Level Physics)

Revision Note