Speed & Velocity (Cambridge (CIE) O Level Physics) : Revision Note

Speed

• The speed of an object is the distance it travels per unit time

• Speed is a scalar quantity

  • This is because it only contains a magnitude (without a direction)

• For objects that are moving with a constant speed, use the equation below to calculate the speed:

  

• Where:

  • Speed is measured in metres per second (m/s)

  • Distance travelled is measured in metres (m)

  • Time taken is measured in seconds (s)

Different speeds

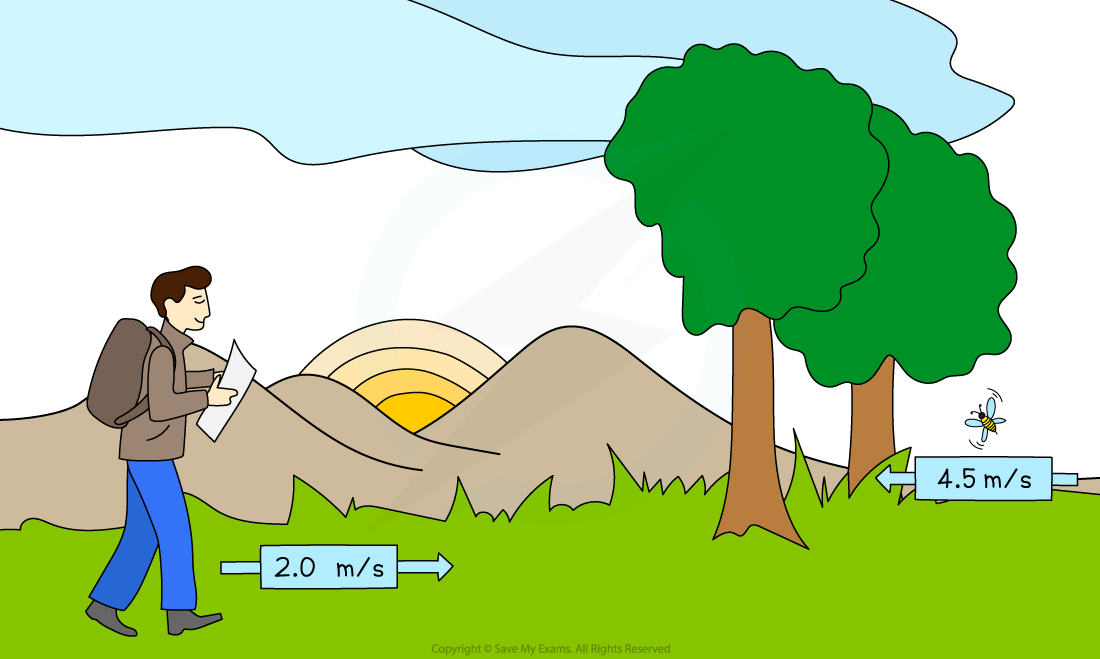
A hiker might have an average speed of 2.0 m/s, whereas a particularly excited bumble bee can have average speeds of up to 4.5 m/s

Average Speed

• In some cases, the speed of a moving object is not constant

  • For example, the object might be moving faster or slower at certain moments in time (accelerating and decelerating)

• The equation for calculating the average speed of an object is:

• The formula for average speed (and the formula for speed) can be rearranged with the help of the formula triangle below:

Average speed equation triangle

Average speed, total distance and time taken equation triangle

How to Use Formula Triangles

• Formula triangles are really useful for knowing how to rearrange physics equations

• To use them:

1. Cover up the quantity to be calculated, this is known as the 'subject' of the equation

2. Look at the position of the other two quantities

   • If they are on the same line, this means they are multiplied

   • If one quantity is above the other, this means they are divided - make sure to keep the order of which is on the top and bottom of the fraction!

• In the example below, to calculate speed, cover-up 'speed' and only distance and time are left

  • This means it is equal to distance (on the top) ÷ time (on the bottom)

Formula triangle example

How to use formula triangles

Velocity

• The velocity of a moving object is similar to its speed, except it also describes the object’s direction

  • The speed of an object only contains a magnitude - it’s a scalar quantity

• Velocity is therefore a vector quantity because it describes both magnitude and direction

  • e.g. ‘15 m/s south’ or ‘250 mph on a bearing of 030°’

Speed vs. velocity

Two objects can have the same speed but a different velocity

• This means velocity can also have a negative value

  • E.g. a ball thrown upwards at a velocity of 3 m/s comes down at a velocity –5 m/s, if upwards is considered positive

  • However, their speeds are still 3 m/s and 5 m/s respectively

• The equation for velocity is very similar to the equation for speed:

• Where:

  • v = velocity in metres per second (m/s)

  • s = displacement, measured in metres (m)

  • t = time, measured in seconds (s)

• Velocity is a vector quantity, so it uses displacement, s, rather than distance which is scalar.