Simplifying Surds (Cambridge (CIE) O Level Maths): Revision Note
Exam code: 4024
Did this video help you?
Surds & exact values
What is a surd?
A surd is the square root of a non-square integer
Using surds lets you leave answers in exact form
e.g.
rather than

How do I do calculations with surds?
Multiplying surds
You can multiply numbers under square roots together
Dividing surds
You can divide numbers under square roots
Factorising surds
You can factorise numbers under square roots
Adding or subtracting surds
You can only add or subtract multiples of “like” surds
This is similar to collecting like terms when simplifying algebra
However
cannot be simplified
You cannot add or subtract numbers under square roots
Consider
This is not equal to
Examiner Tips and Tricks
If your calculator gives an answer as a surd, leave the value as a surd throughout the rest of your working.
This will ensure you do not lose accuracy throughout your working.
Simplifying surds
How do I simplify surds?
To simplify a surd, factorise the number using a square number, if possible
If multiple square numbers are a factor, use the largest
Use the fact that
and then work out any square roots of square numbers
E.g.
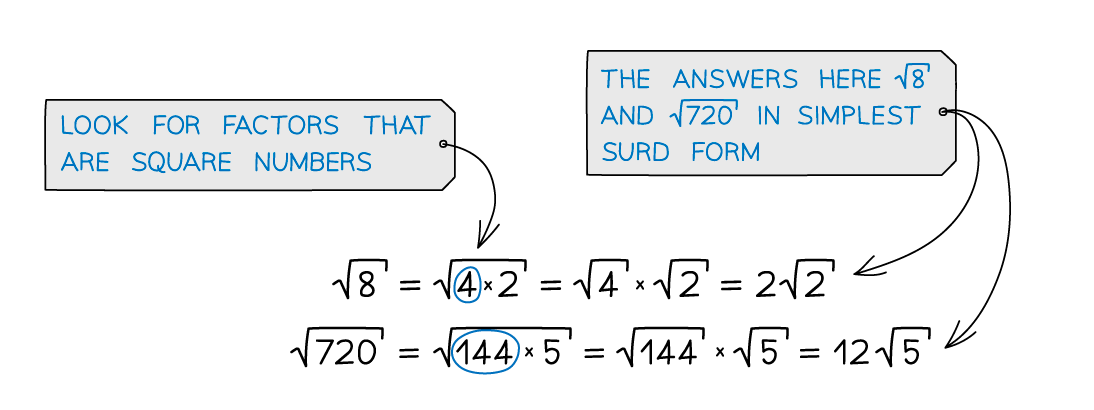
When simplifying multiple surds, simplify each separately
This may produce surds which can then be collected together
E.g.
can be rewritten as
This simplifies to
These surds can then be collected together
You may have to expand double brackets containing surds
This can be done in the same way as multiplying out double brackets algebraically, and then simplifying
The property
can be used to simplify the expression, once expanded
E.g.
expands to
This simplifies to
which gives
Worked Example
Write in the form
where
is a positive integer.
Simplify both surds separately by finding the highest square number that is a factor of each of them
9 is a factor of 54, so
4 is a factor of 24, so
Simplify the whole expression by collecting the like terms

You've read 0 of your 5 free revision notes this week
Unlock more, it's free!
Did this page help you?