Revision NotesExam QuestionsPast Papers
O LevelFurther MathsCambridge (CIE)Additional MathsRevision NotesAlgebra & FunctionsLogarithmic & Exponential FunctionsLaws of Logarithms

Laws of Logarithms (Cambridge (CIE) O Level Additional Maths): Revision Note

Amber

Author

Amber

Last updated

Did this video help you?

Laws of Logarithms

What are the laws of logarithms?

  • Laws of logarithms allow you to simplify and manipulate expressions involving logarithms

    • The laws of logarithms are equivalent to the laws of indices

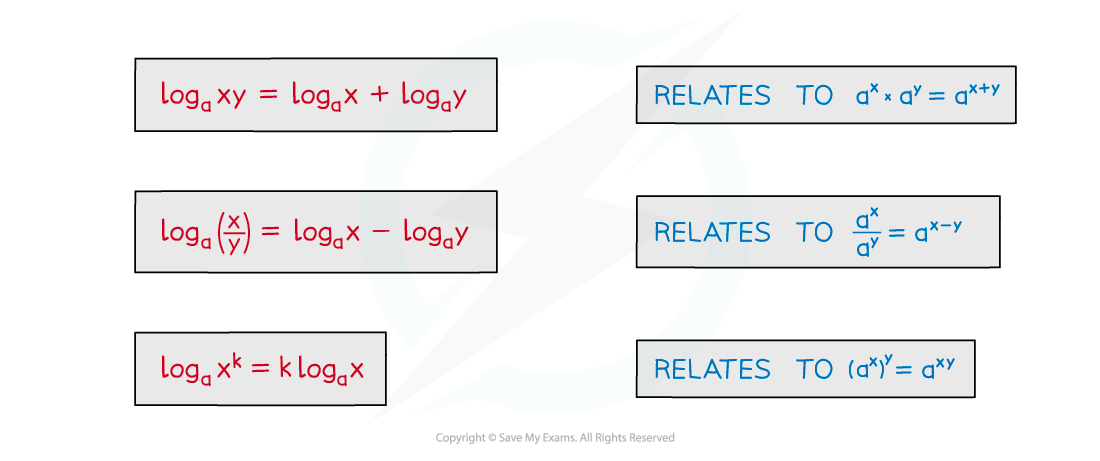
  • The laws you need to know are, given a comma space x comma space y space greater than space 0:

    • log subscript a x y equals blank log subscript a x plus blank log subscript a y

      • This relates to a to the power of x cross times blank a to the power of y equals a to the power of x plus y end exponent

    • log subscript a x over y equals blank log subscript a x blank negative space log subscript a y 

      • This relates to a to the power of x divided by blank a to the power of y equals a to the power of x minus y end exponent

    • log subscript a x to the power of m equals blank m log subscript a x 

      • This relates to left parenthesis a to the power of x right parenthesis to the power of y equals a to the power of x y end exponent

The laws of logarithms

  • There are also some particular results these lead to

    • log subscript a a equals 1

    • log subscript a a to the power of x equals x

    • a to the power of log subscript a x end exponent equals x

    • log subscript a 1 equals 0

    • log subscript a stretchy left parenthesis 1 over x stretchy right parenthesis equals negative log subscript a x

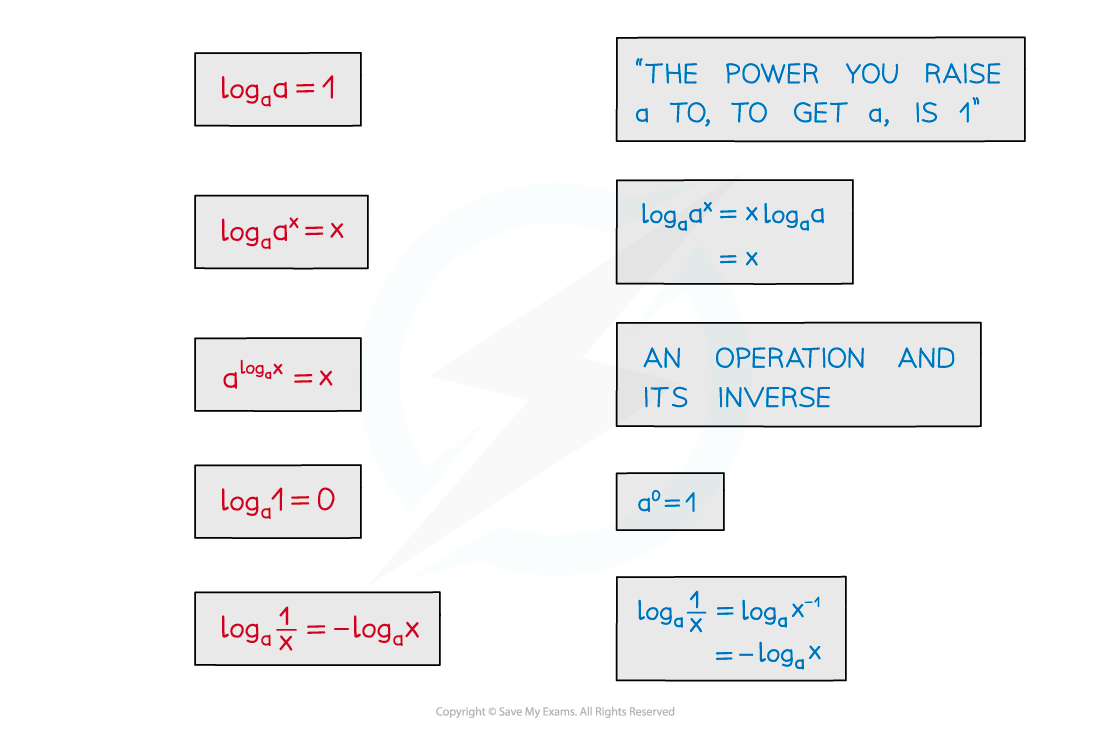
Properties of logarithms

  • Beware…

    • …log subscript a open parentheses x plus y close parentheses space not equal to space log subscript a x plus log subscript a y

  • These results apply to ln space x space left parenthesis log subscript e x right parenthesis too

    • Two particularly useful results are

      • ln space e to the power of x space equals space x

      • e to the power of ln x end exponent space equals space x

How do I use the laws of logarithms?

  • Laws of logarithms can be used to …

    • … simplify expressions

    • … solve logarithmic equations

    • … solve exponential equations

Simplifying an expression using the laws of logarithms

Examiner Tips and Tricks

  • Remember to check whether your solutions are valid

    • log (x+k) is only defined if x > -k

    • You will lose marks if you forget to reject invalid solutions

Worked Example

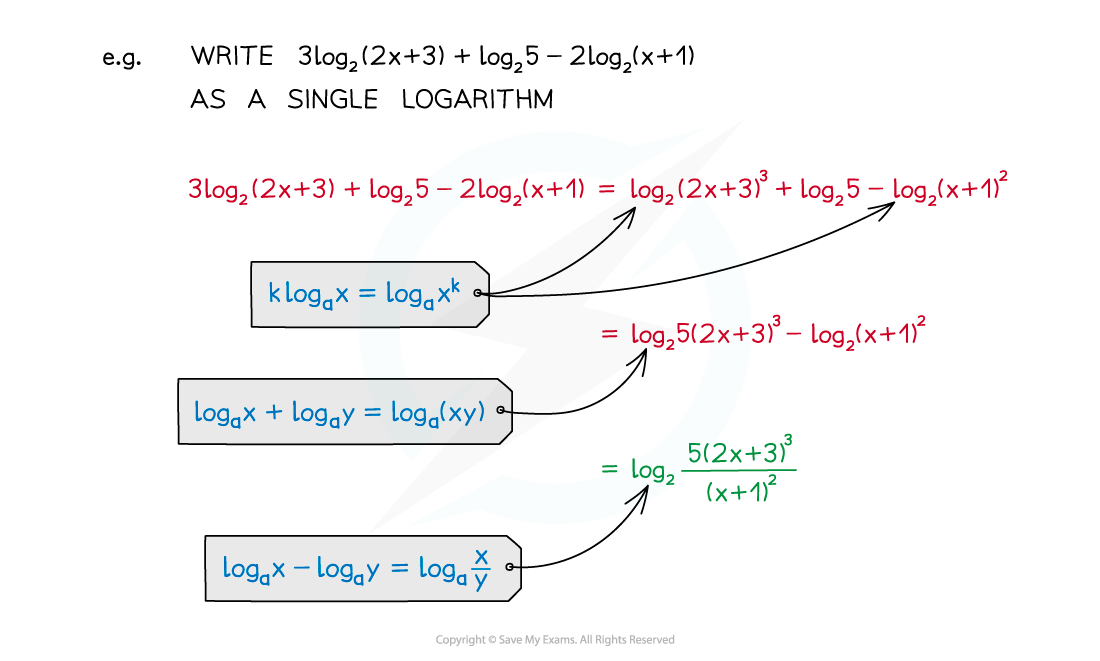
a) Write the expression 2 space log space 4 space minus space log space 2 in the form log space k, where k space element of space straight integer numbers.

  

Using the law log subscript a x to the power of m space equals space m log subscript a x we can rewrite 2 space log space 4 as log space 4 squared space equals space log space 16.

  

2 space log space 4 space minus space log space 2 space equals space log space 16 space minus space log space 2

Using the law log subscript a space x over y space equals space log subscript a space x space minus space space log subscript a space y:

 

 log space 16 space minus space log space 2 space equals space log space 16 over 2 space equals space log space 8

bold 2 bold log bold space bold 4 bold space bold minus bold space bold log bold space bold 2 bold space bold equals bold space bold log bold space bold 8

b)   Hence, or otherwise, solve 2 space log space 4 minus log space 2 equals negative log blank 1 over x.

  

   

Rewrite the equation using the expression found in part (a).

2 space log space 4 space minus space log space 2 space equals space log space 8: 

  

log space 8 space equals space minus space log space 1 over x

Using the index law 1 over x space equals space x to the power of negative 1 end exponent::

 

 log space 8 space equals space minus space log space x to the power of negative 1 end exponent

Using the law log subscript a x to the power of m space equals space m log subscript a x:

  

log space 8 equals space space log space x to the power of negative open parentheses negative 1 close parentheses end exponent space equals space log space x

 

Compare the two sides.

 log space 8 equals space log space x

bold italic x bold space bold equals bold space bold 8

Change of Base

How do I change the base of a logarithm?

  • The formula for changing the base of a logarithm is

log subscript a x equals blank fraction numerator log subscript b x over denominator log subscript b a end fraction

  • The value you choose for b does not matter, however if you do not have a calculator, you can choose b such that the problem will be possible to solve

Why change the base of a logarithm?

  • The laws of logarithms can only be used if the logs have the same base

    • If a problem involves logarithms with different bases, you can change the base of the logarithm and then apply the laws of logarithms

  • Changing the base of a logarithm can be particularly useful if you need to evaluate a log problem without a calculator

    • Choose the base such that you would know how to solve the problem from the equivalent exponent

  • This formula had more use when calculators were less advanced

    • Some old calculators only had a button for logarithm of base 10

    • To calculate log subscript 5 7on these calculators you would have to enter

      • fraction numerator log subscript 10 invisible function application 7 over denominator log subscript 10 invisible function application 5 end fraction

  • The formula can be useful when evaluating a logarithm where the two numbers are powers of a common number

    • log subscript 4 8 equals fraction numerator log subscript 2 8 over denominator log subscript 2 4 end fraction equals 3 over 2

  • The formula can be useful when you are solving equations and two logarithms have different bases

    • For example, if you have log subscript 3 k and log subscript 9 n within the same equation

      • You can rewrite log subscript 9 n as fraction numerator log subscript 3 invisible function application n over denominator log subscript 3 invisible function application 9 end fraction blank which simplifies to 1 half log subscript 3 n

      • Or you can rewrite log subscript 3 k as fraction numerator log subscript 9 invisible function application k over denominator log subscript 9 invisible function application 3 end fraction blank which simplifies to 2 log subscript 9 k

  • The formula also allows you to derive and use a formula for switching the numbers:

log subscript a invisible function application x equals fraction numerator 1 over denominator log subscript x invisible function application a end fraction

  • Using the fact that log subscript x x equals 1

Examiner Tips and Tricks

  • It is very rare that you will need to use the change of base formula

  • Only use it when the bases of the logarithms are different

Worked Example

By choosing a suitable value for b, use the change of base law to find the value of  log subscript 8 space end subscript 32 without using a calculator.

Note that 8 and 32 are both powers of 2, where 8 = 23  and 32 = 25.

Therefore we can choose b = 2 in the change of base formula. 

log subscript a space x space equals space fraction numerator log subscript b space x over denominator log subscript b space a end fraction: 

  

log subscript 8 32 space equals space fraction numerator log subscript 2 32 over denominator log subscript 2 8 end fraction

If  23 = 8 then log2 8 = 3 and if  25 = 32 then log2 32 = 5.

 

 fraction numerator log subscript 2 32 over denominator log subscript 2 8 end fraction equals 5 over 3

bold log subscript bold 8 bold 32 bold space bold equals bold space bold 5 over bold 3 bold equals bold space bold 1 bold 2 over bold 3

You've read 0 of your 5 free revision notes this week

Sign up now. It’s free!

Join the 100,000+ Students that ❤️ Save My Exams

the (exam) results speak for themselves:

    Test yourself

    Did this page help you?

    Previous:Logarithmic FunctionsNext:Exponential Equations
    Amber

    Author: Amber

    Expertise: Maths

    Amber gained a first class degree in Mathematics & Meteorology from the University of Reading before training to become a teacher. She is passionate about teaching, having spent 8 years teaching GCSE and A Level Mathematics both in the UK and internationally. Amber loves creating bright and informative resources to help students reach their potential.