Polynomial Division (Cambridge (CIE) O Level Additional Maths) : Revision Note
Did this video help you?
Polynomial Division
What is polynomial division?
Polynomial division is the process of dividing two polynomials
This is usually only useful when the degree of the denominator is less than or equal to the degree of the numerator
Polynomial division is a method for splitting polynomials into factor pairs
(with or without a remainder term)
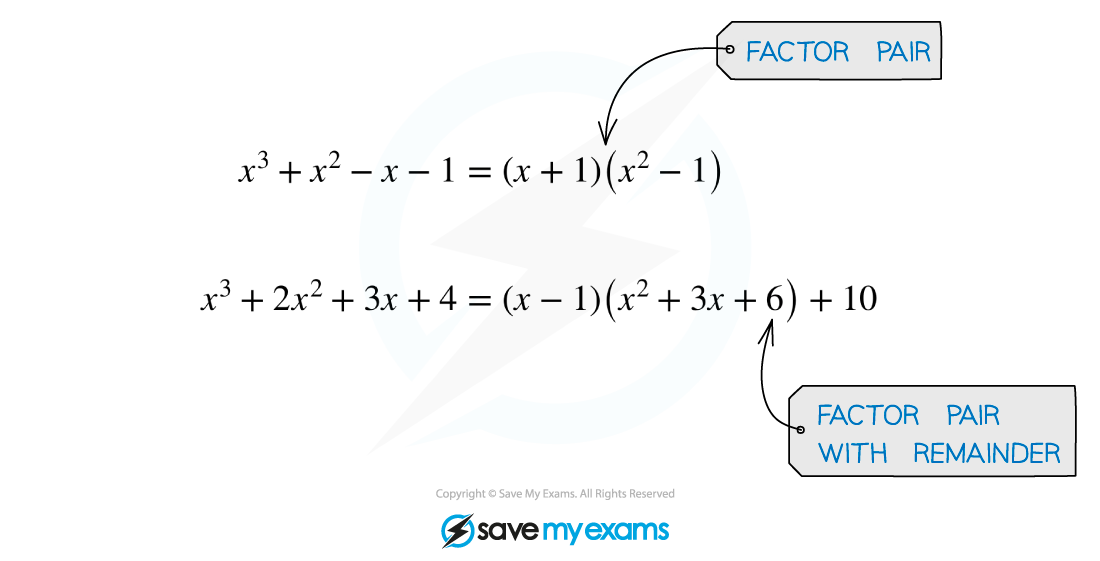
The main uses of polynomial division are
factorising polynomials
simplifying 'top-heavy' algebraic fractions
How do I divide polynomials?
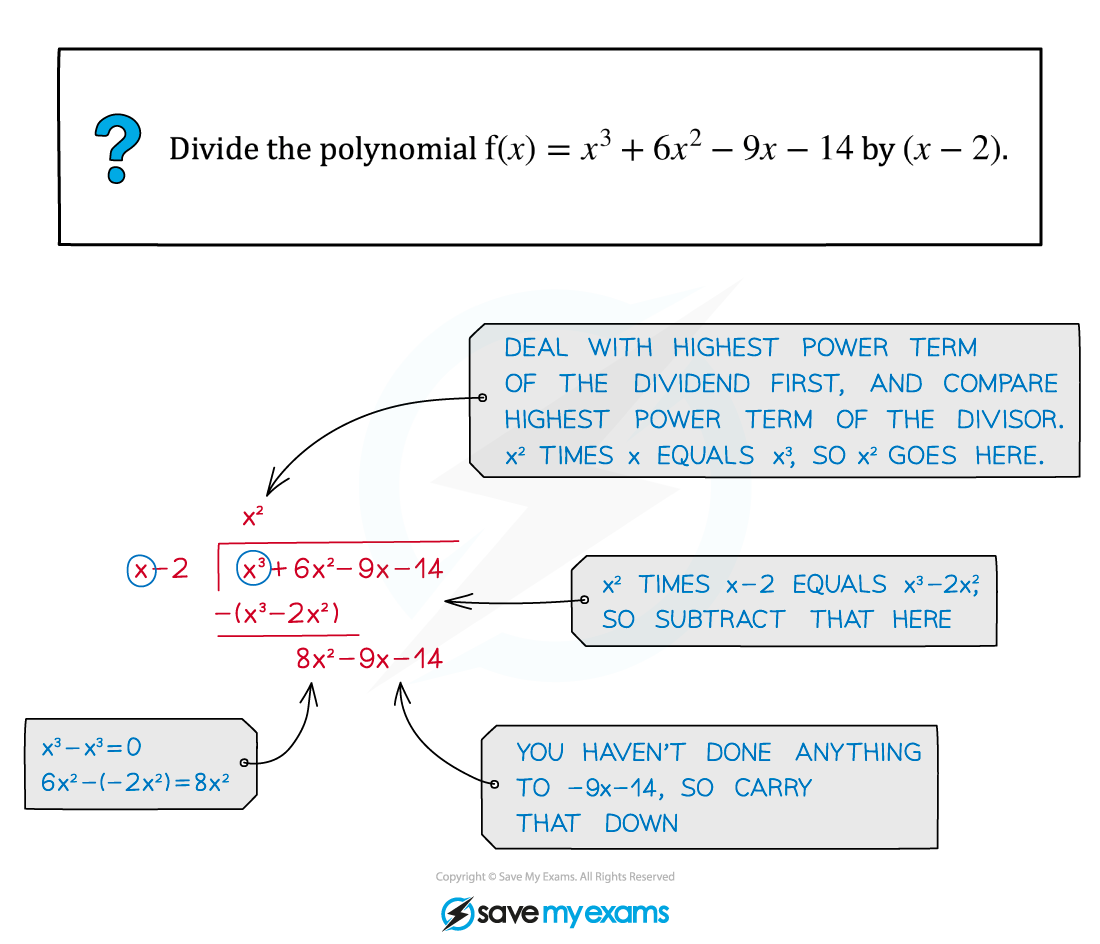
The method used for polynomial division is just like the long division method for numbers
sometimes called the 'bus stop method'

The answer to a polynomial division question is built up term by term
Start by dividing by the highest power term
Write out this multiplied by the divisor and subtract
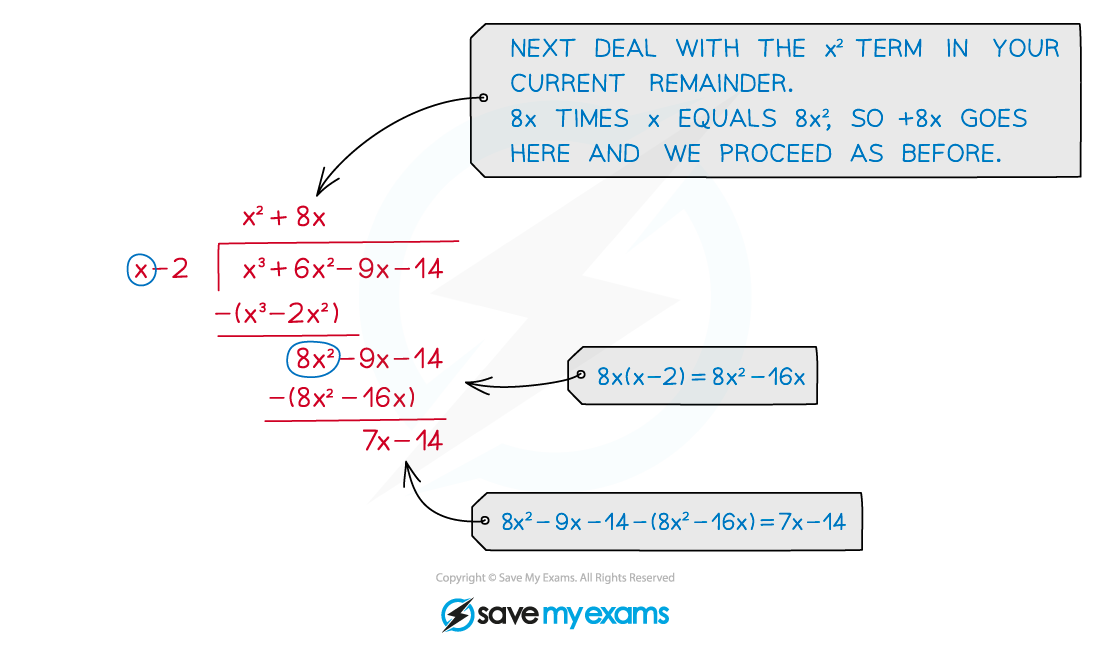
Continue to divide by each reducing power term and subtracting your answer each time
Continue until you are left with zero
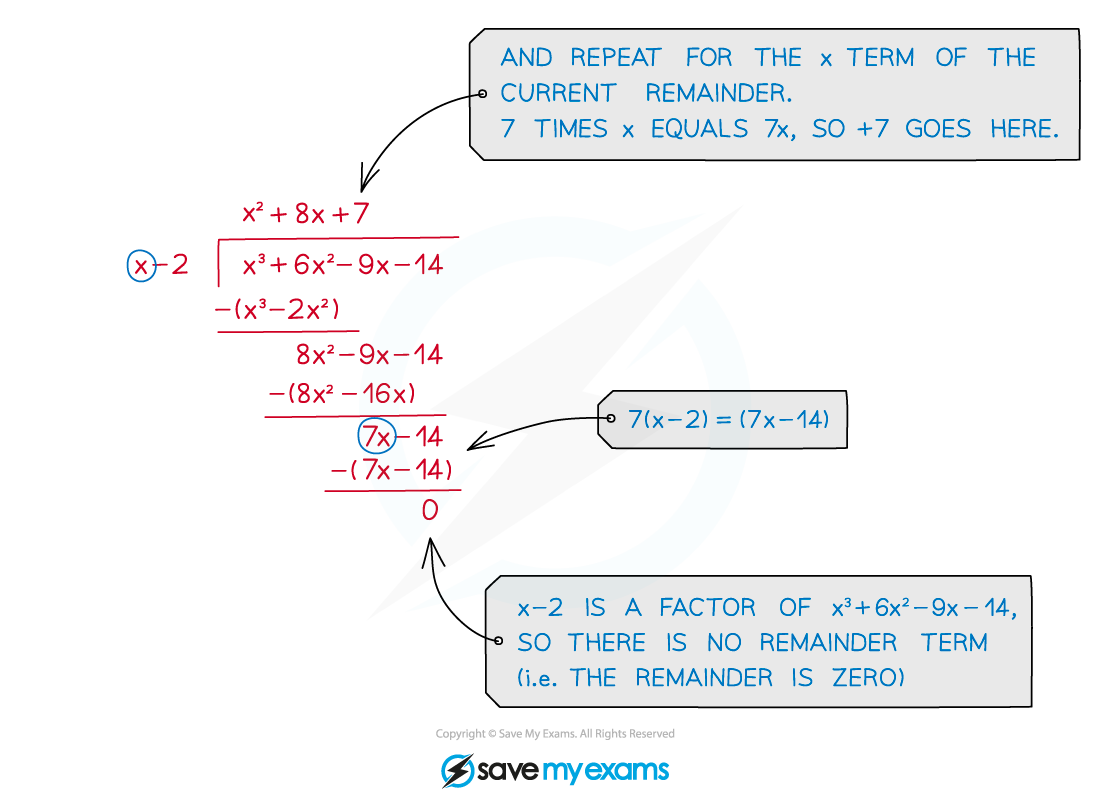
If the divisor is not a factor of the polynomial then there will be a remainder term left at the end of the division
Worked Example
For the polynomial divide
by
and write the remainder.
Set up the polynomial division ('bus stop')
There is no term so write this as
in the method. There is no
term so write this as
in the method.
The first division step to consider is .
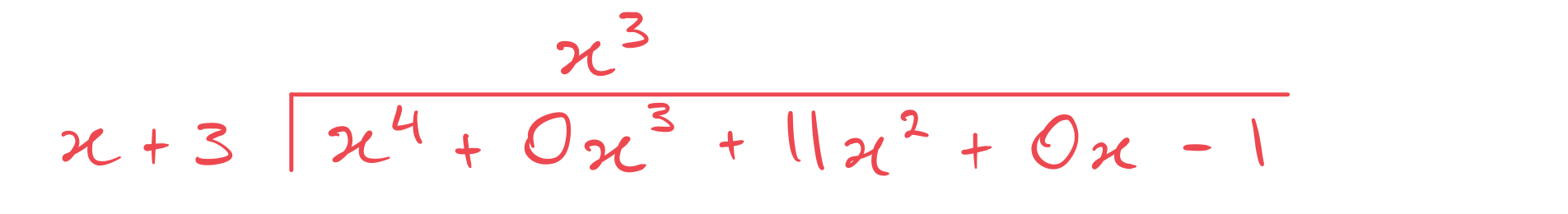
.Multiply by
and subtract from
.
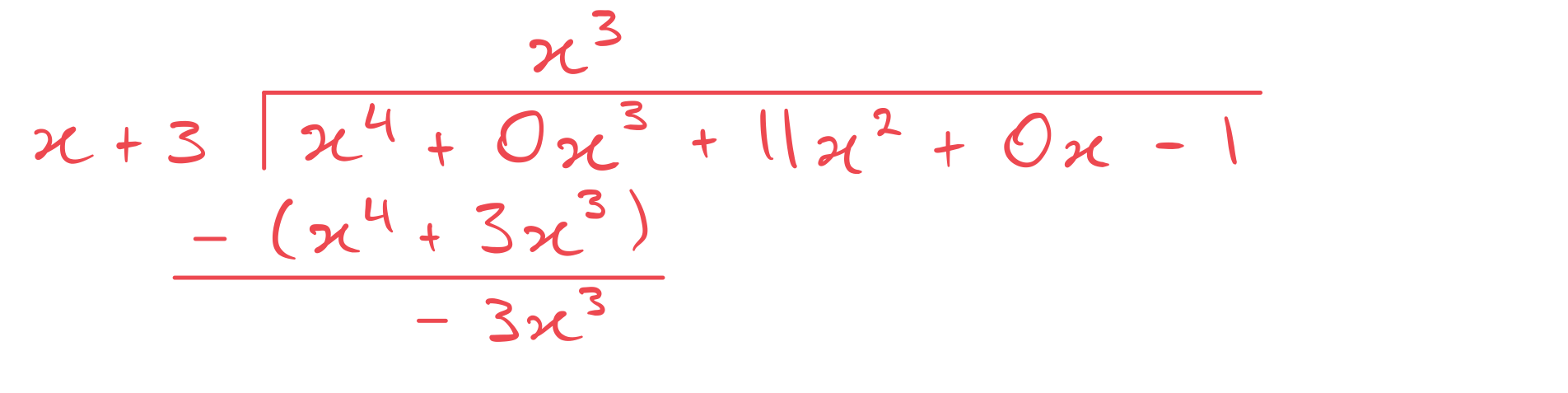
Bring the down and divide
by
. Continue with each step until you are finished.
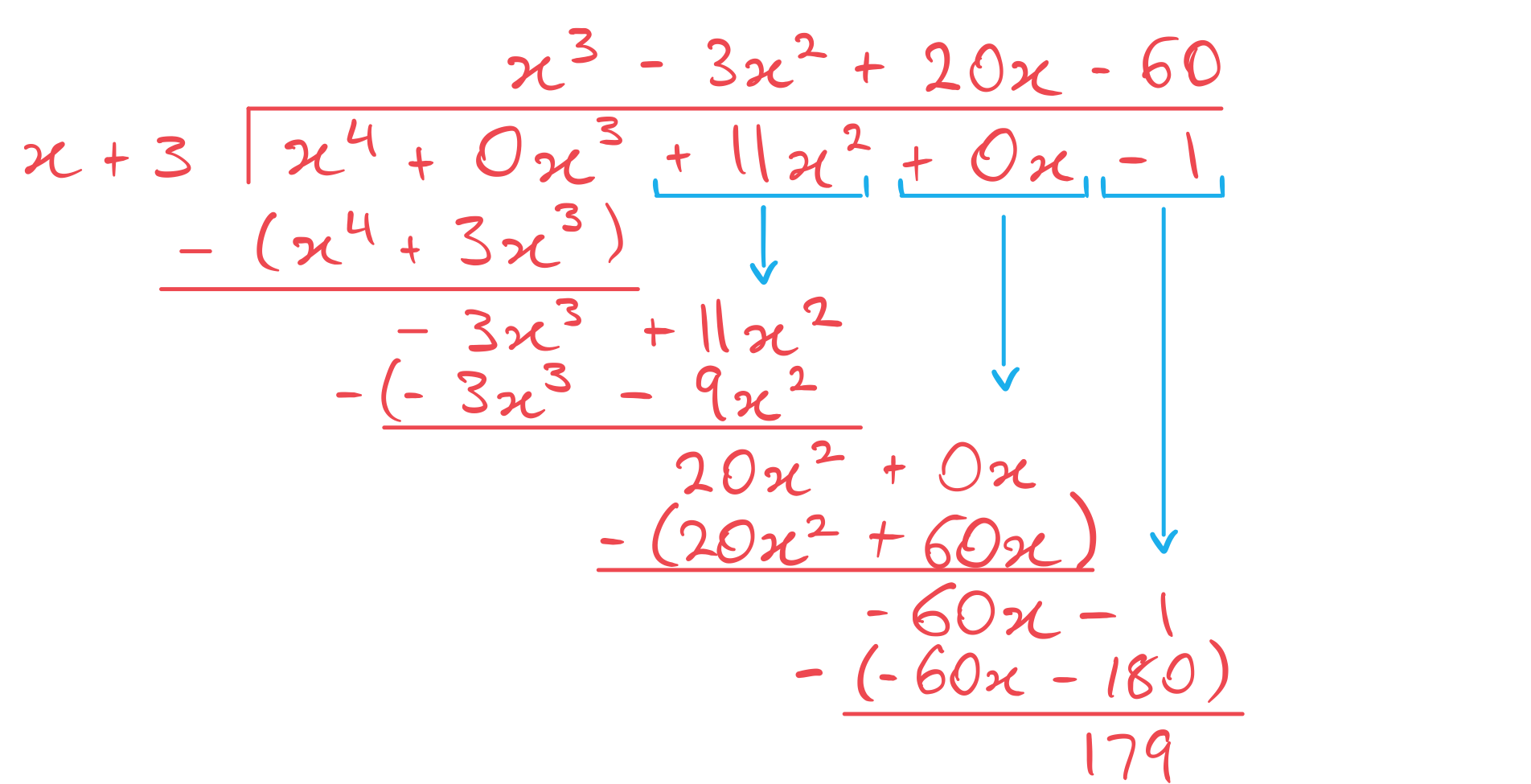
The remainder is 179.
Quadratic Divisor
What is meant by a quadratic divisor?
Polynomial division usually involves dividing by a linear term
a term of the form
where
is a constant and usually an integer
It is possible to divide a polynomial by a quadratic term (and cubic, etc)
this would be a term of the form
where
and
are constants
this is what is meant by a quadratic divisor
How do I divide by a quadratic divisor?
The process is the same as for a linear divisor
However, as
will not divide into
(in the polynomial division sense at least) the remainder, if there is one, could be of linear form, i.e.
where
and
are constants
It is possible that
and so the remainder is still a constant
Examiner Tips and Tricks
Give yourself plenty of room to do polynomial division
Not only will this help avoid errors, it will make your working clear
If you make a mistake and change something, fine, but if your method starts to get too messy it is best to restart
Worked Example
Find the remainder when is divided by
.
Set up the polynomial division ('bus stop') - there is no term so write this as
in the method.
The first division step to consider is .
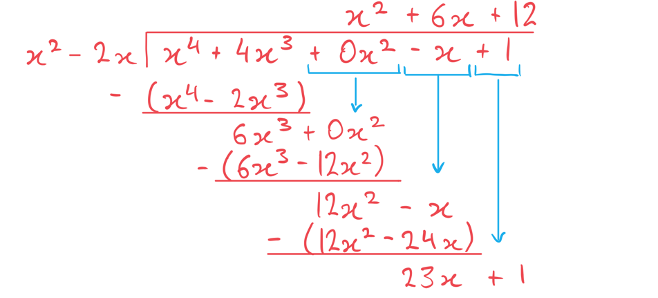
The remainder is .

You've read 0 of your 5 free revision notes this week
Sign up now. It’s free!
Did this page help you?