Problem Solving using Vectors

Showing that two lines or vectors are parallel
- Two vectors are parallel if they are scalar multiples of each other
- i.e. $a = k b$ where $k$ is a constant
  - See Vector Addition
Finding the midpoint of two (position) vectors
Showing that three points are collinear
- Collinear describes points that lie on the same straight line
  - e.g. The points $(- 2, - 2), (3, 3)$ and $(8, 8)$ all lie on the line with equation $y = x$
  - Vectors can be used to show this, and similar, results
Results concerned with geometric shapes
- Shapes with parallel lines are often involved
  - e.g. parallelogram, rhombus
- These often include lines or vectors being split into ratios
  - e.g. The point $Q$ lies on the line $P R$ such that $\vec{P Q} : \vec{Q R} = 3 : 1$

If the point $A$ has position vector $a$ and the point $B$ has position vector $b$
- the position vector of the midpoint of $A B$ is $\frac{1}{2} (a + b)$
This can be derived by considering
- $\vec{A B} = b - a$
  - using the result from Vector Addition
- If $M$ is the midpoint of $A B$ then
  - $\vec{A M} = \frac{1}{2} \vec{A B}$
- Therefore, the position vector of the midpoint, $\vec{O M}$ is
  - $\vec{O M} = \vec{O A} + \vec{A M} = a + \frac{1}{2} (b - a)$
    $\vec{O M} = \frac{1}{2} (a + b)$

Three points are collinear if they all lie on the same straight line
There are two ways to show this for three points, $A, B$ and $C$ say
- Method 1
  Show that $\vec{A B} = k \vec{A C}$ where $k$ is a constant
  i.e. show that $\vec{A B}$ and $\vec{A C}$ are scalar multiples of each other
  - As the vectors are scalar multiples they will have the same direction (and so be parallel)
  - So as both vectors start at point $A$ , they must be collinear
- Method 2
  Show that $\vec{A B} = k \vec{B C}$ AND that point $B$ lies on both the vectors $\vec{A B}$ and $\vec{B C}$
Which method you should use will depend on the information given and how you happen to see the question

Problems involving geometric shapes involve finding paths around the shape using known vectors
- there will be many other vectors in the shape that are equal and/or parallel to the known vectors
The following grid is made up entirely of parallelograms, with the vectors $a$ and $b$ defined as marked in the diagram:

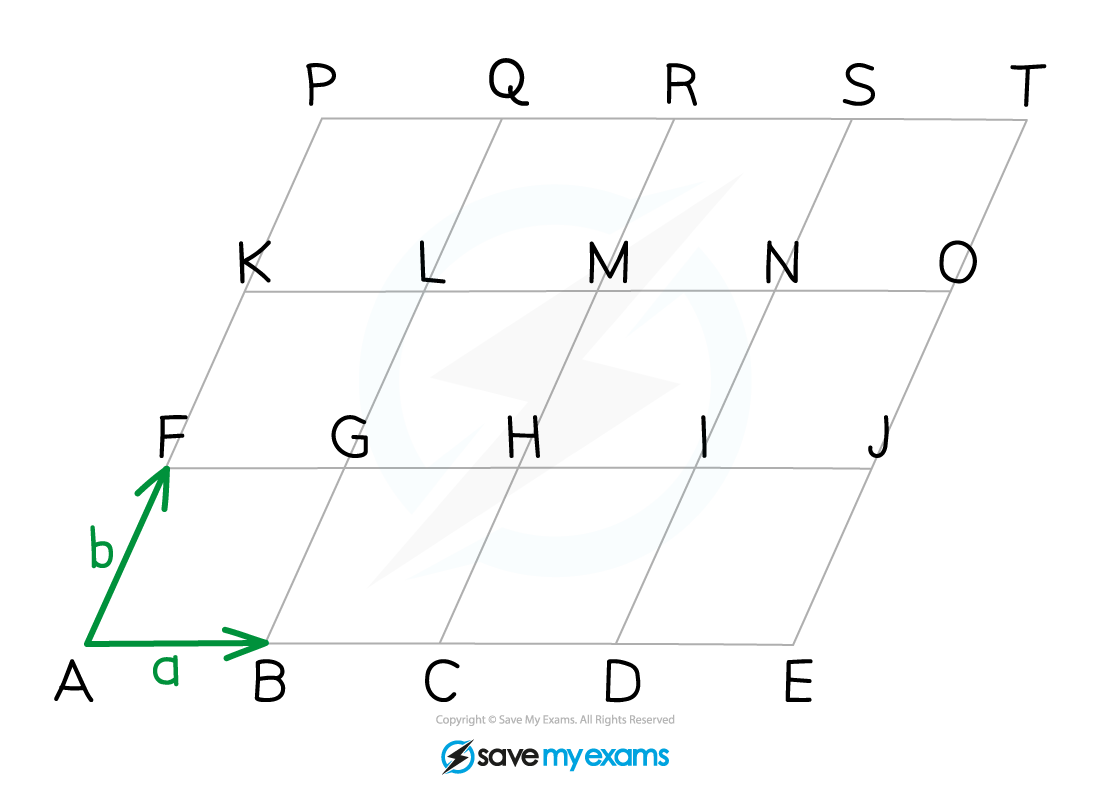
Vector parallelogram grid, Maths revision notes

Note the difference between "specific" and "general" vectors
- The vector $\vec{A B}$ in the diagram is specific and refers only to the vector starting at $A$ and ending at $B$
  - However, the vector $a$ is a general vector
    - any vector the same length as $\vec{A B}$ and parallel to it is equal to $a$
    - e.g. $\vec{R S} = a$
  - Vector $b$ is also a general vector
    - e.g. $\vec{G L} = b$
- There will also be vectors in the diagram that are the same magnitude but have the opposite direction to $a$ or $b$
  - e.g. $\vec{O N} = - a, \vec{J E} = - b$
There are also many instances of the vector addition result $\vec{F B} = b - a$
- e.g. $\vec{P L} = b - a$
There are many scalar multiples of the vectors $a$ or $b$
- e.g. $\vec{F I} = 3 a, \vec{I S} = 2 b, \vec{Q E} = 3 (b - a)$
Using a combination of these it is possible to describe a vector between any two points in terms of $a$ and $b$

Diagrams are helpful in vector questions
- If a diagram has been given, label it and add to it as you progress through a question
- If a diagram has not been given, draw one, it does not need to be accurate!

The following diagram consists of a grid of identical parallelograms.

Vectors $a$ and $b$ are defined by $a = \vec{A B}$ and $b = \vec{A F}$ .

Vector parallelogram grid, Maths revision notes

Write the following vectors in terms of $a$ and $b$ .

\vec{A E}

To get from A to E follow vector $a$ four times (to the right).

\begin{array}{rcl} \vec{A E} & = & \vec{A B} + \vec{B C} + \vec{C D} + \vec{D E} \\ = & a + a + a + a \end{array}

\vec{A E} = 4 a

\vec{G T}

There are many ways to get from G to T.
One option is to go from G to Q (

b

twice), and then from Q to T ( $a$ three times).

\begin{array}{rcl} \vec{G T} & = & \vec{G L} + \vec{L Q} + \vec{Q R} + \vec{R S} + \vec{S T} \\ = & b + b + a + a + a \end{array}

\vec{G T} = 3 a + 2 b

Point

Z

is such that it is midpoint of

H M

.
Find the vector

\vec{P Z}

There are many ways to get from P to Z.
One option is to go from P to R (

a

twice), and then from R to Z (

- b

one-and-a-half times).

\begin{array}{rcl} \vec{P Z} & = & \vec{P R} + \vec{R Z} \\ = & a + a - b - \frac{1}{2} b \end{array}

\vec{P Z} = 2 a - \frac{3}{2} b

Problem Solving using Vectors (Cambridge O Level Additional Maths)