Language of Sequences & Series (Cambridge O Level Additional Maths)

What is a progression?

• A progression (also called a sequence) is an ordered set of numbers with a rule for finding all of the numbers in the sequence
  • For example 1, 3, 5, 7, 9, … is a sequence with the rule ‘start at one and add two to each number’
• The numbers in a progression are often called terms
• The terms of a progression are often referred to by letters with a subscript
  • This will often be the letter u
  • So in the progression above, u₁ = 1, u₂ = 3, u₃ = 5 and so on
• Each term in a progression can be found by substituting the term number into formula for the n^th term

What is a series?

• You get a series by summing up the terms in a progression
  • E.g. For the sequence 1, 3, 5, 7, … the associated series is 1 + 3 + 5 + 7 +  …
• We use the notation S_n to refer to the sum of the first n terms in the series
  • S_n = u₁ + u₂ + u₃ + … + u_n
  • So for the series above S₅ = 1 + 3 + 5 + 7 + 9 = 25

What is sigma notation?

• Sigma notation is used to show the sum of a certain number of terms in a sequence
• The symbol Σ is the capital Greek letter sigma
• Σ stands for ‘sum’
  • The expression to the right of the Σ tells you what is being summed, and the limits above and below tell you which terms you are summing

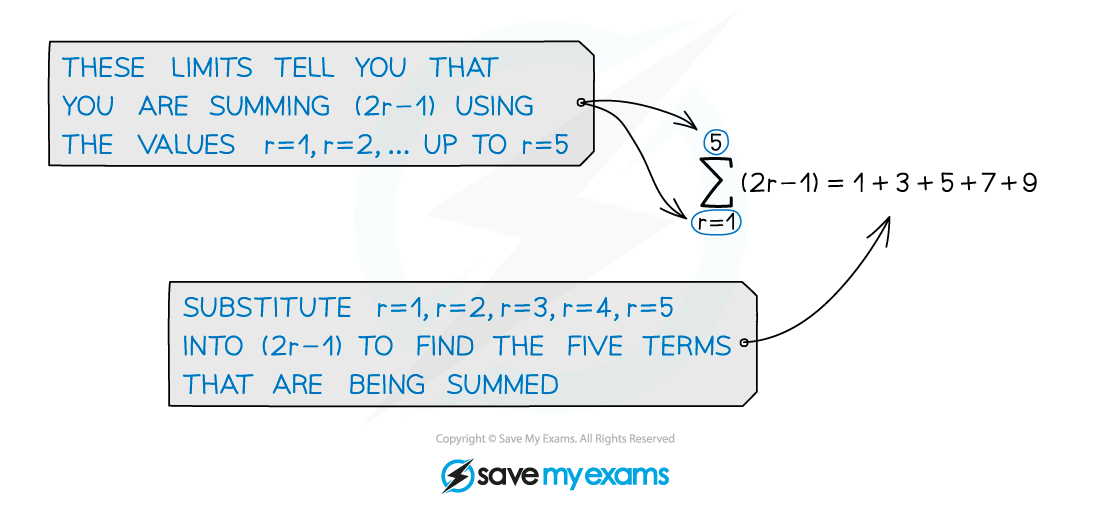
• Be careful, the limits don’t have to start with 1
  • For example   or 
  • r and k are commonly used variables within sigma notation