Quotient Rule (Cambridge (CIE) O Level Additional Maths): Revision Note
Exam code: 4037
Did this video help you?
Quotient rule
What is the quotient rule?
The quotient rule is a formula that allows you to differentiate a quotient of two functions
i.e. one function divided by another
If
where u and v are functions of x then the quotient rule is:
In function notation, if
then the quotient rule can be written as:
As with the product rule, ‘dash notation’ may be used to make remembering it easier
Final answers should match the notation used throughout the question
How do I know when to use the quotient rule?
The quotient rule is used when trying to differentiate a fraction where both the numerator and denominator are functions of
if the numerator is a constant, negative powers can be used
if the denominator is a constant, treat it as a factor of the expression\
How do I use the quotient rule?
Make it clear what
and
are
arranging them in a square can help
opposite diagonals match up (like they do for product rule)
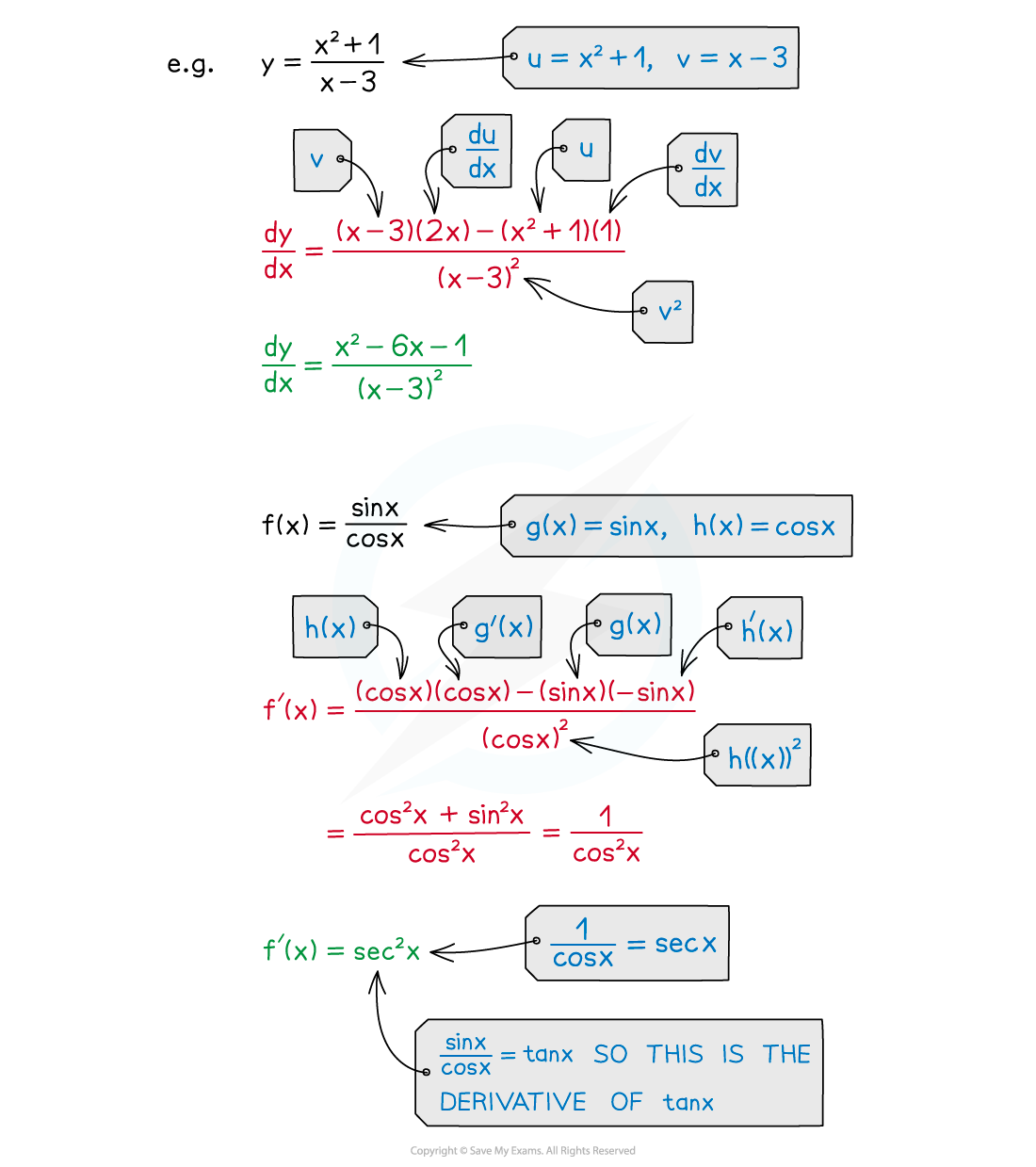
STEP 1
Identify the two functions, and
Differentiate both and
with respect to
to find
and
STEP 2
Obtain by applying the quotient rule formula
Be careful using the formula – because of the minus sign in the numerator, the order of the functions is important Simplify the answer if straightforward or if the question requires a particular form
Examiner Tips and Tricks
The quotient rule formula is not on the list of formulas page – you have to memorise it
however if you do forget it in an exam, you could rewrite
as
and then use the product rule
(the quotient rule is really doing exactly this)
Be careful using the formula – because of the minus sign in the numerator the order of the functions is important!
Worked Example


Unlock more, it's free!
Did this page help you?