Venn Diagrams

What is a Venn diagram?

A Venn diagram is a way to illustrate events from an experiment and are particularly useful when there is an overlap (or lack of) between possible outcomes
A Venn diagram consists of
- a rectangle representing the sample space
- a bubble (usually drawn as a circle/ellipse/oval) for each event
  - Bubbles may or may not overlap depending on which outcomes are shared between events
  - Bubble(s) is not a technical term but we like it!

How are Venn diagrams labelled and what do the numbers inside mean?

The rectangle is usually labelled with an $S$ – as it represents the sample space (all possible outcomes from the experiment)
- It is often referred to as the Universal Set and is commonly labelled with $S, U, ξ$ (the Greek lower case letter Xi) or $E$ (Kunstler script font)
  There is no standardised symbol used for this purpose
Bubbles are labelled with their event name (A, B, etc)
The numbers inside a Venn diagram (there should be one in each region) will represent either a frequency or a probability
- In the case of probabilities being shown, all values should total 1

3-1-2-fig1-basic-venn

What do the different regions and bubbles overlapping mean on a Venn diagram?

This will depend on how many events there are and how the outcomes overlap
Venn diagrams show ‘AND’ and ‘OR’ statements easily
Venn diagrams also instantly show mutually exclusive events
- Independence can be deduced from the probabilities involved

3-1-2-fig2-various-venns-part-1

3-1-2-fig2-various-venns-part-2

How do I solve probability problems involving Venn diagrams?

Draw, or add to a given Venn diagram, filling in as many values as possible from the information provided in the question
It is usually helpful to work from the centre outwards
- i.e. fill in intersections (overlaps) first
- This is particularly crucial with Venn diagrams with three events
  - the intersection of events $A$ and $B$ will include the intersection of events A, B and C
  - a question would make it clear if a given frequency or probability is only for events A and B , and not C
Any frequencies or probabilities not given may be able to be calculated from those that are
- Use the results from Basic Probability to deduce missing frequencies or probabilities and answer questions
  - - $P (not A) = 1 - P (A)$
    - For independent events, $P (A and B) = P (A) \times P (B)$
    - For mutually exclusive events, $P (A or B) = P (A) + P (B)$
Check a completed Venn diagram that the frequencies sum to the total involved or that probabilities sum to 1

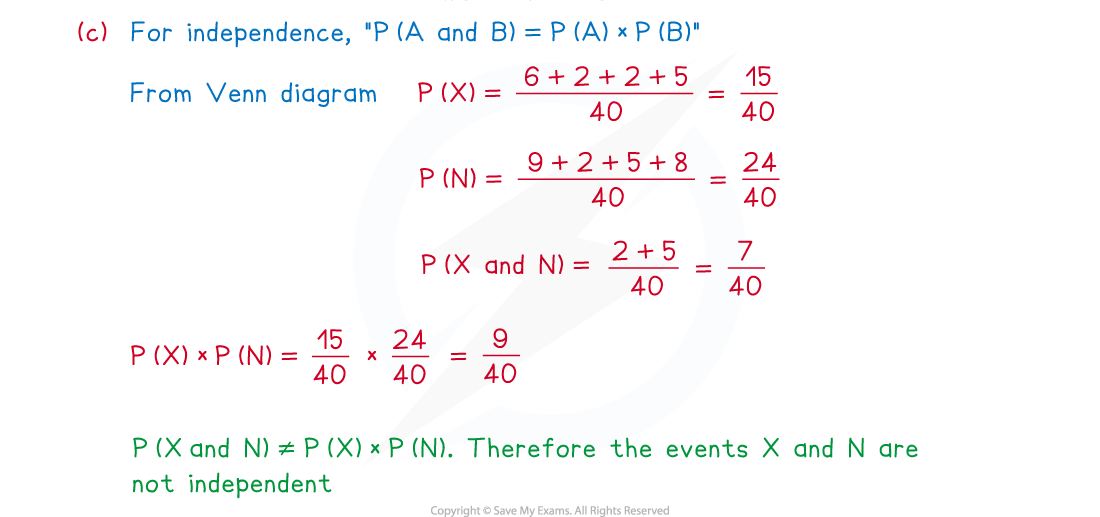
Worked example

40 people were surveyed regarding which games consoles they owned.

8 people said they owned a Playstation 5 ( $P$ ) and an Xbox Series X ( $X$ ); 11 people said they owned a Playstation 5 ( $P$ ) and a Nintendo Switch ( $N$ ); 7 people said they owned an Xbox Series X ( $X$ ) and a Nintendo Switch ( $N$ ).

4 people said they owned none of these consoles whilst 2 people said they owned all 3.

Of those people that owned only one games console, twice as many owned a Nintendo Switch as a Playstation 5 and half as many owned an Xbox Series X as a Playstation 5.

(a)

Draw a complete Venn diagram to illustrate the information given above.

(b)

One of the 40 people is chosen at random. Find the probability that this person

(i)

owns all three consoles,

(ii)

owns exactly two consoles,

(iii)

doesn’t own a Playstation 5.

(c)

Determine if the events

X

and

N

are independent.

(a)

Draw a complete Venn diagram to illustrate the information given above.

3-1-2-fig3-we-solution-part-1

(b)

One of the 40 people is chosen at random. Find the probability that this person

(i)

owns all three consoles,

(ii)

owns exactly two consoles,

(iii)

doesn’t own a Playstation 5.

3-1-2-fig3-we-solution-part-2

(c)

Determine if the events

X

and

N

are independent.

Examiner Tip

Always draw the box in a Venn diagram; it represents all possible outcomes of the experiment so is a crucial part of the diagram, the bubbles merely represent the events we are particularly interested in
In complicated problems it can be helpful to draw a “mini-Venn” diagram for part of a question and shade/label the regions of the diagram that are relevant to that part

Venn Diagrams (Edexcel International AS Maths: Statistics 1)

Revision Note

Venn Diagrams

What is a Venn diagram?

How are Venn diagrams labelled and what do the numbers inside mean?

What do the different regions and bubbles overlapping mean on a Venn diagram?

How do I solve probability problems involving Venn diagrams?

Worked example

Examiner Tip

You've read 0 of your 5 free revision notes this week

Sign up now. It’s free!

Join the 100,000+ Students that ❤️ Save My Exams

Author: Paul