Coefficient of Friction - Inclined Planes (Edexcel International AS Maths: Mechanics 1)

How is the coefficient of friction used in problems involving inclined planes?

• On an inclined plane the basic principles are the same as for coefficient of friction on horizontal surfaces
• The important directions are parallel to the plane and perpendicular to the plane (instead of horizontal and vertical)

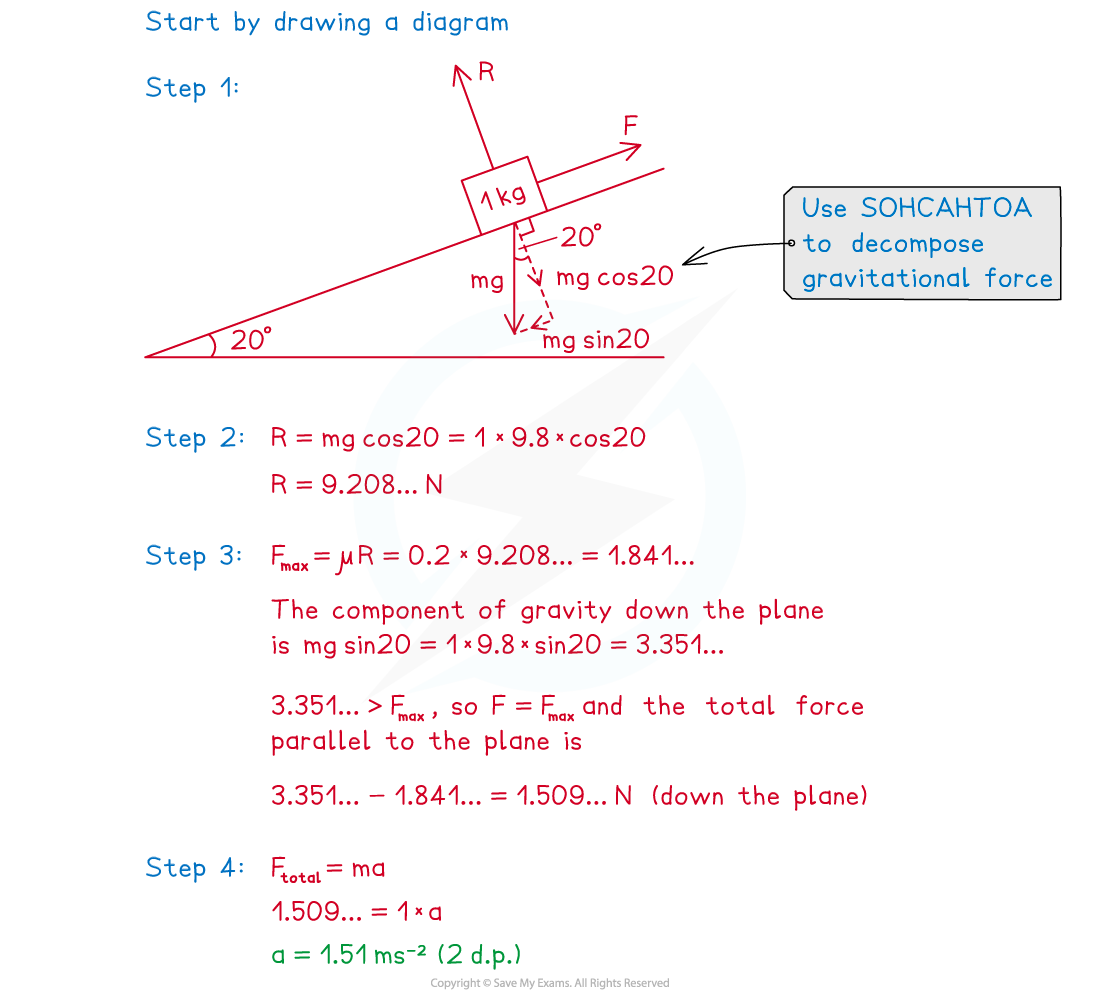
Step 1. Resolve weight (and any other forces if necessary) into components parallel and perpendicular to the plane

Step 2. Calculate the normal reaction force R

• • Be careful – R will never simply be equal to mg in an inclined plane problem!
  • R will always be directed perpendicular to and away from the plane
  • the magnitude of R will always be such as to make the total perpendicular force zero

Step 3. Calculate F_MAX= μR and find the resultant (total force) of all the forces on the object that are parallel to the plane

• • The force of friction F will always act parallel to the plane in the direction opposite to any movement (or potential movement) of the object
  • Remember – if the resultant of the other parallel forces is less than or equal to F_MAX then friction will exactly balance those forces out and the object will remain stationary

Step 4. Use F = ma to determine the acceleration of the object

A wooden block of mass 1 kg is released from rest on a rough plane that is inclined at 20°  above the horizontal. The coefficient of friction between the block and the plane is 0.2. Find the acceleration of the block.