Nuclear Binding Energy & Mass Deficit (Edexcel International A Level Physics)

Nuclear Binding Energy

• Experiments into nuclear structure have found that the total mass of a nucleus is less than the sum of the masses of its constituent nucleons

  • This difference in mass is known as the mass defect or mass deficit

  • Mass defect is defined as:

    The difference between the measured mass of a nucleus and the sum total of the masses of its constituents

• The mass defect Δm of a nucleus can be calculated using:

• Where:

  • Z = proton number

  • A = nucleon number

  • m_p = mass of a proton (kg)

  • m_n = mass of a neutron (kg)

  • m_total = measured mass of the nucleus (kg)

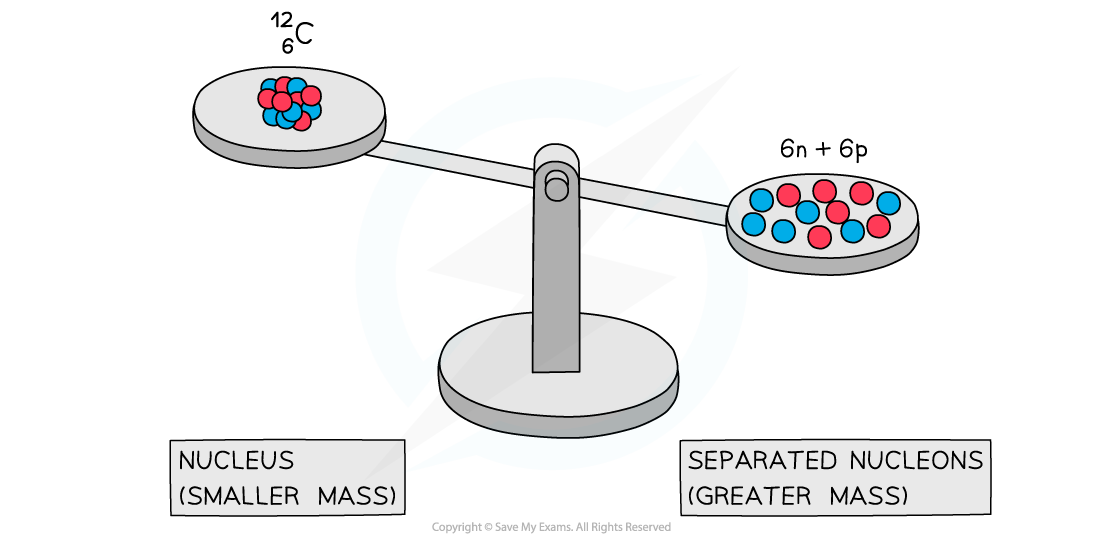
A system of separated nucleons has a greater mass than a system of bound nucleons

• Due to mass-energy equivalence, this decrease in mass implies that energy is released

• Energy and mass are proportional, so, the total energy of a nucleus is less than the sum of the energies of its constituent nucleons

• Binding energy is defined as:

  The energy required to break a nucleus into its constituent protons and neutrons

• The formation of a nucleus from a system of isolated protons and neutrons therefore releases energy, making it an exothermic reaction

  • This can be calculated using the equation:

Mass-Energy Equivalence

• Einstein showed in his Theory of Relativity that matter can be considered a form of energy and hence, he proposed:

  • Mass can be converted into energy

  • Energy can be converted into mass

• This is known as mass-energy equivalence, and can be summarised by the equation:

• Where:

  • E = energy (J)

  • m = mass (kg)

  • c = the speed of light (m s^-1)

• Some examples of mass-energy equivalence are:

  • The fusion of hydrogen into helium in the centre of the sun

  • The fission of uranium in nuclear power plants

  • Nuclear weapons

  • High-energy particle collisions in particle accelerators