Magnetic Force on a Charged Particle (Edexcel International A Level (IAL) Physics): Revision Note
Exam code: YPH11
Magnetic Force on a Charged Particle
The magnetic force on an isolated moving charged particle, such as a proton, is given by the equation:
F = BQv
Where:
F = magnetic force on the particle (N)
B = magnetic flux density (T)
Q = charge of the particle (C)
v = speed of the particle (m s-1)
This is the maximum force on the charged particle, when F, B and v are mutually perpendicular
Therefore if a particle travels parallel to a magnetic field, it will not experience a magnetic force
Current is the rate of flow of positive charge
This means that the direction of the 'current' for a flow of negative charge (e.g. an electron beam) is in the opposite direction to its motion
If the charged particle is moving at an angle θ to the magnetic field lines, then the size of the magnetic force F is given by the equation:
F = BQv sin θ
This equation shows that:
The size of the magnetic force is zero if the angle θ is zero (i.e. the particle moves parallel to the field lines)
The size of the magnetic force is maximum if the angle θ is 90° (i.e. the particle moves perpendicular to field lines)
Worked Example
A beta particle is incident at 70° to a magnetic field of flux density 0.5 mT, travelling at a speed of 1.5 × 106 m s–1.
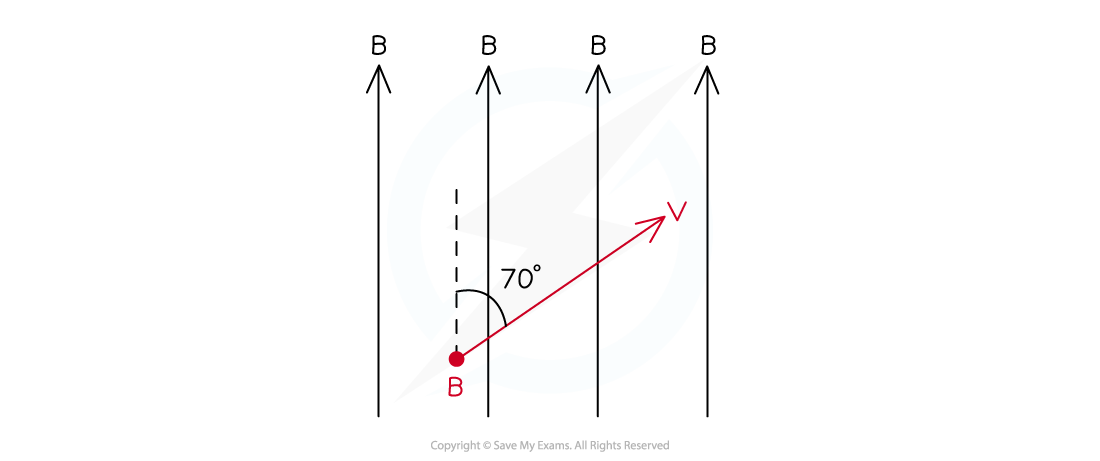
Calculate:
a) The magnitude of the magnetic force on the beta particle
b) The magnitude of the maximum possible force on a beta particle in this magnetic field, travelling with the same speed
Answer:
Part (a)
Step 1: Write out the known quantities
Magnetic flux density B = 0.5 mT = 0.5 × 10–3 T
Speed v = 1.5 × 106 m s–1
Angle θ between the flux and the velocity = 70°
Step 2: Substitute quantities into the equation for magnetic force on a charged particle
A beta particle is an electron
Therefore, the magnitude of electron charge Q = 1.6 × 10–19 C
Substituting values gives:
F = BQv sin θ
F = (0.5 × 10–3) × (1.6 × 10–19) × (1.5 × 106) × sin (70)
F = 1.1 × 10–16 N
Part (b)
Step 1: Write out the known quantities
Magnetic flux density B = 0.5 mT = 0.5 × 10–3 T
Speed v = 1.5 × 106 m s–1
Step 2: Determine the angle to the flux lines
Angle θ between the flux and the velocity = 90° if the magnetic force is a maximum
Step 3: Substitute quantities into the equation for magnetic force on a charged particle
The magnitude of electron charge Q = 1.6 × 10–19 C
Substituting values gives:
F = BQv sin θ = BQv when sin 90 = 1
F = (0.5 × 10–3) × (1.6 × 10–19) × (1.5 × 106)
F = 1.2 × 10–16 N
Examiner Tips and Tricks
Remember not to mix this up with F = BIL sin θ!
F = BIL sin θ is the force on a current-carrying conductor
F = BQv sin θ is the force on an isolated moving charged particle (which may be inside a conductor)
Another super important fact to remember for typical exam questions is that the magnetic force on a charged particle is centripetal, because it always acts at 90° to the particle's velocity. You should practise using Fleming's Left Hand Rule to determine the exact direction!
Fleming's Left Hand Rule for a Charged Particle
Fleming’s left hand rule can be used to determine the direction of the magnetic force on a moving charged particle in a magnetic field
The First Finger = direction of the magnetic field
The Second Finger = direction of conventional current (i.e. the velocity of a moving positive charge)
The Thumb = direction of the magnetic force
Fleming's Left Hand Rule is illustrated in the image below:
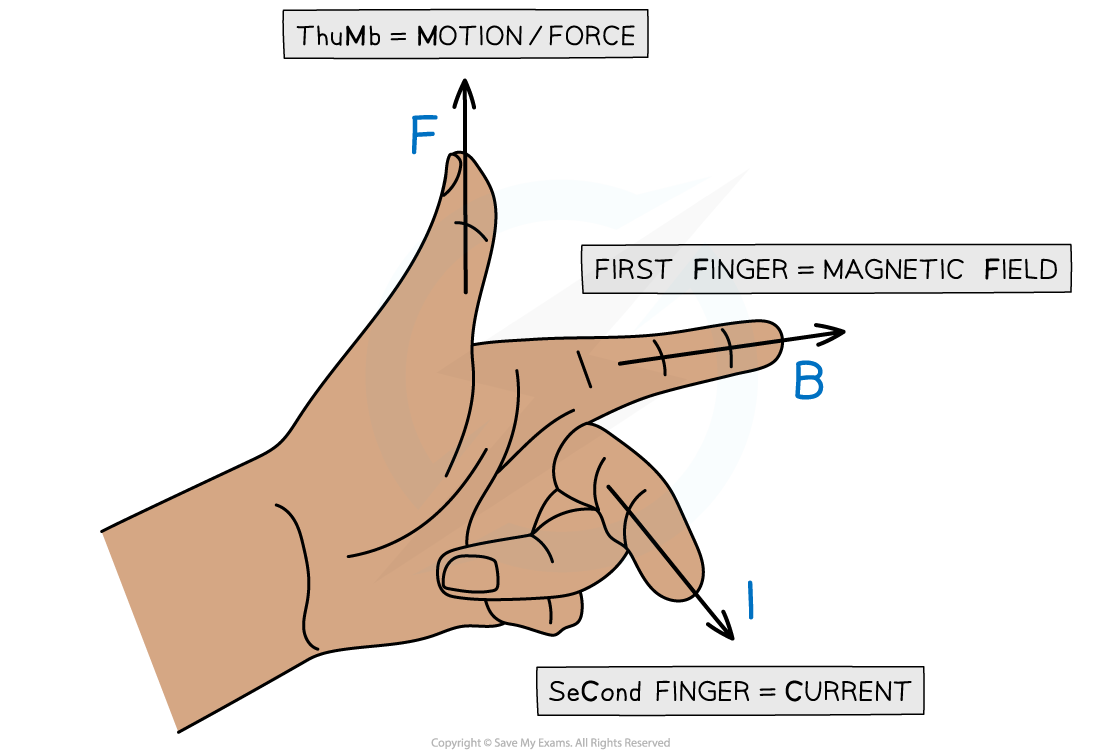
Fleming's Left Hand Rule shows the magnetic force, magnetic field and conventional current (flow of positive charge) are all perpendicular to each other
Since this is represented in 3D space, sometimes the flow of charge, magnetic force or magnetic field could be directed into or out of the page, not just left, right, up and down
The direction of the magnetic field into or out of the page in 3D is represented by the following symbols:
Dots (sometimes with a circle around them) represent the magnetic field directed out of the plane of the page
Crosses represent the magnetic field directed into the plane of the page
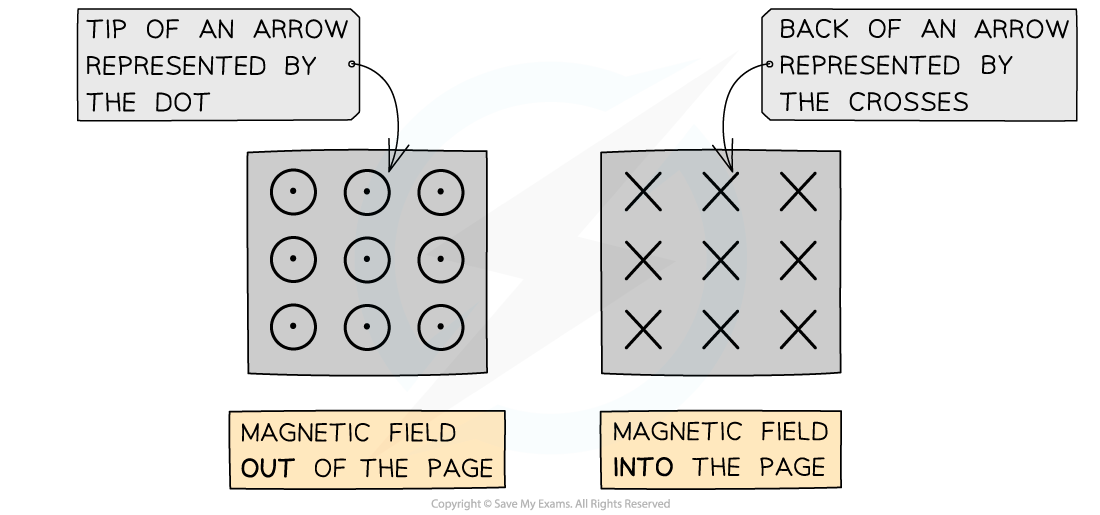
The magnetic field into or out of the page is represented by circles with dots or crosses
The way to remember this is by imagining an arrow used in archery or darts:
If the arrow is approaching head-on, such as out of a page, only the very tip of the arrow can be seen (a dot)
When the arrow is moving away, such as into a page, only the cross of the feathers at the back can be seen (a cross)
An Electron Moving in a Magnetic Field
The maximum magnetic force on a moving charged particle is always perpendicular to its velocity
This means magnetic forces cause charged particles to move in a circle
The direction of magnetic force on the charged particle can be determined using Fleming's Left Hand Rule
The image below shows an electron incident on a uniform magnetic field B directed into the page:
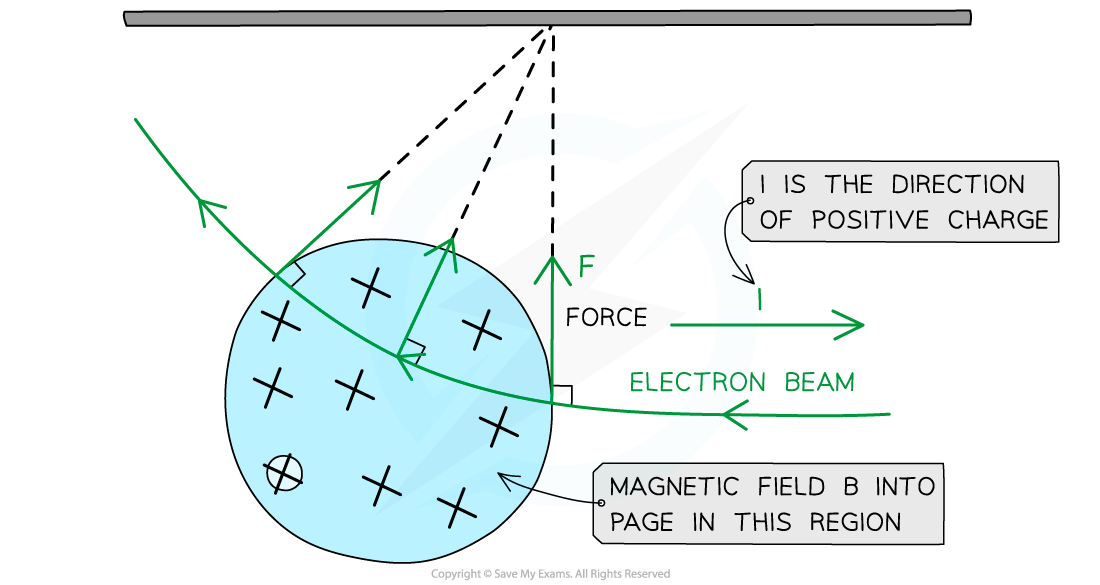
An electron moving to the left as shown is equivalent to a conventional positive charge or current moving to the right. Using Fleming's Left Hand Rule, the direction of the force can be determined
According to Fleming's Left Hand Rule:
B is directed into the page, therefore the first finger should point into the page
The conventional current (or velocity of a positive charge) is directed to the right (because an electron is moving to the left), therefore the second finger should point to the right
Therefore, the force on the electron as shown by the thumb is initially upwards as it enters the magnetic field
The force due to the magnetic field is always perpendicular to the velocity of the electron
Note: this is equivalent to circular motion
Therefore, the magnetic force on a moving charge is a centripetal force
The centripetal force is what keeps moving charges following a circular trajectory
Examples of the direction of the magnetic force on positive and negative particles are:
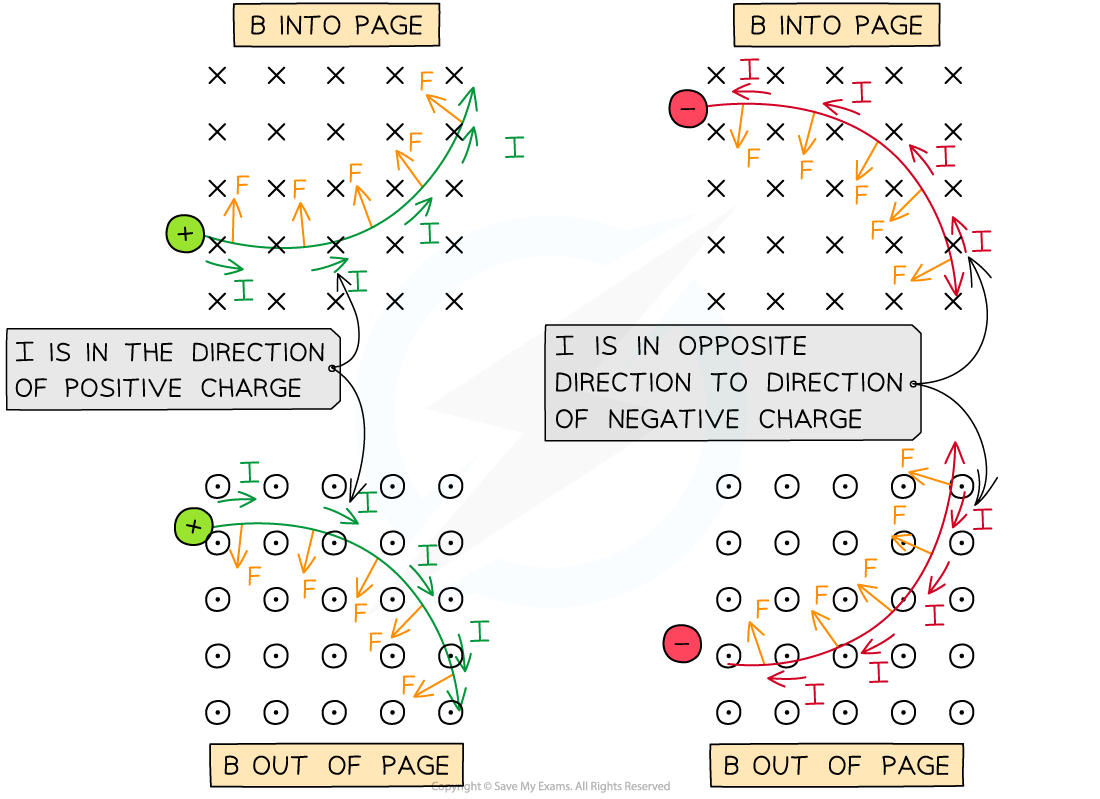
The direction of the magnetic force F on positive and negative particles in a B field in and out of the page
Fleming’s Left Hand Rule can be used again to find the direction of the force, magnetic field and velocity
The key difference is that the second finger, representing current I (direction of positive charge), can now be used as the direction of velocity v of a positive charge
Examiner Tips and Tricks
The most important point when using Fleming's left hand rule is the direction of the charge (or current flow). This is always the direction of positive charge. Therefore, for electrons, or negatively charged ions, you should point your second finger for the current in the opposite direction to its motion.

Unlock more, it's free!
Did this page help you?