Thermionic Emission (Edexcel International A Level Physics)

• When metals are heated, the conduction electrons within them gain energy
• If these electrons gain sufficient energy, they are able to leave the surface of the metal
  • This is known as thermionic emission 
  • This is similar to the photoelectric effect, but the energy absorbed by electrons in this case is due to thermal energy, rather than the energy absorbed by incident photons

• Once electrons are released from a metal surface they may be accelerated by electric or magnetic fields

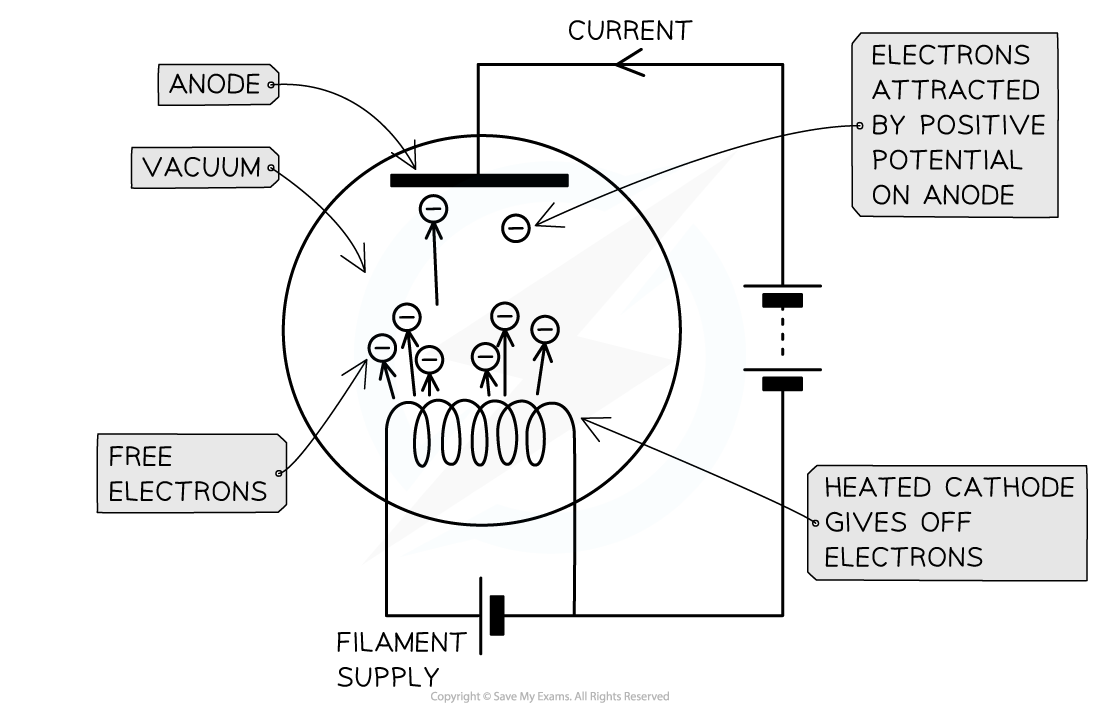
Electrons are emitted from the (negative) cathode and accelerated to the (positive) anode

Step 1: List the known quantities

• • Potential difference = 5 kV = 5000 V
  • Mass of an electron, m_e = 9.11 × 10^–31 kg
  • Electron charge e = 1.6 × 10^–19 C

Step 2: Equate the kinetic energy gained by the electron to the energy transferred across the potential difference

• • Potential difference V is the energy transferred W per unit of charge Q, or 
  • Since the charge in this case is an electron, Q = e and so W = eV
  • Therefore, the kinetic energy gained is equal to eV so we can write:

Step 3: Make speed v the subject of the equation

• • Rearranging this equation for v gives:

Step 4: Substitute quantities and calculate the speed v

• • Substituting known quantities gives:

= 41 908 313... = 4.2 × 10⁷ m s^–1