Angular Velocity (Edexcel International A Level (IAL) Physics) : Revision Note
Angular Velocity
The angular velocity ω of a body in circular motion is defined as:
The rate of change of angular displacement
In other words, angular velocity is the angle swept out by an object in circular motion, per second
Angular velocity is a vector quantity and is measured in rad s–1
Since it is a vector, it has a magnitude (angular speed) and direction
Angular velocity is calculated using:
ω =
Where:
Δθ = change in angular displacement (radians)
Δt = time interval (s)
It is related to linear speed, v by the equation
v = ωr
Where:
v = linear speed, v (m s-1)
ω = angular speed (rad s-1)
r = radius of orbit (m)
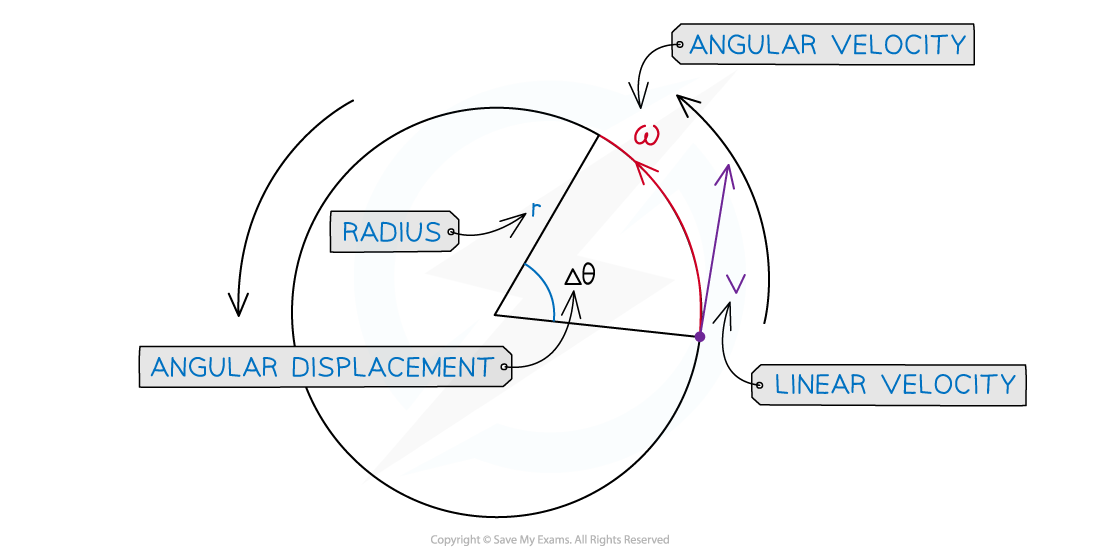
When an object is in uniform circular motion, velocity constantly changes direction, but the speed stays the same
Taking the angular displacement of a complete orbit or revolution as 2π radians, the angular velocity ω an be calculated using the equation:
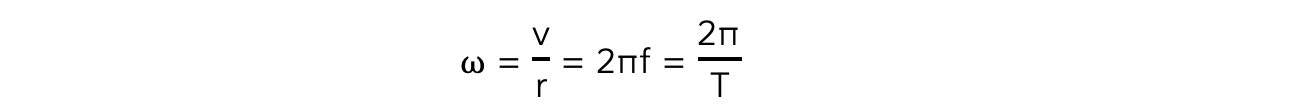
Where:
T = the time period (s)
f = frequency (Hz)
This equation shows that:
The greater the rotation angle θ in a given amount of time T, the greater the angular velocity ω
An object travelling with the same linear velocity, but further from the centre of orbit (larger r) moves with a smaller angular velocity (smaller ω)
Worked Example
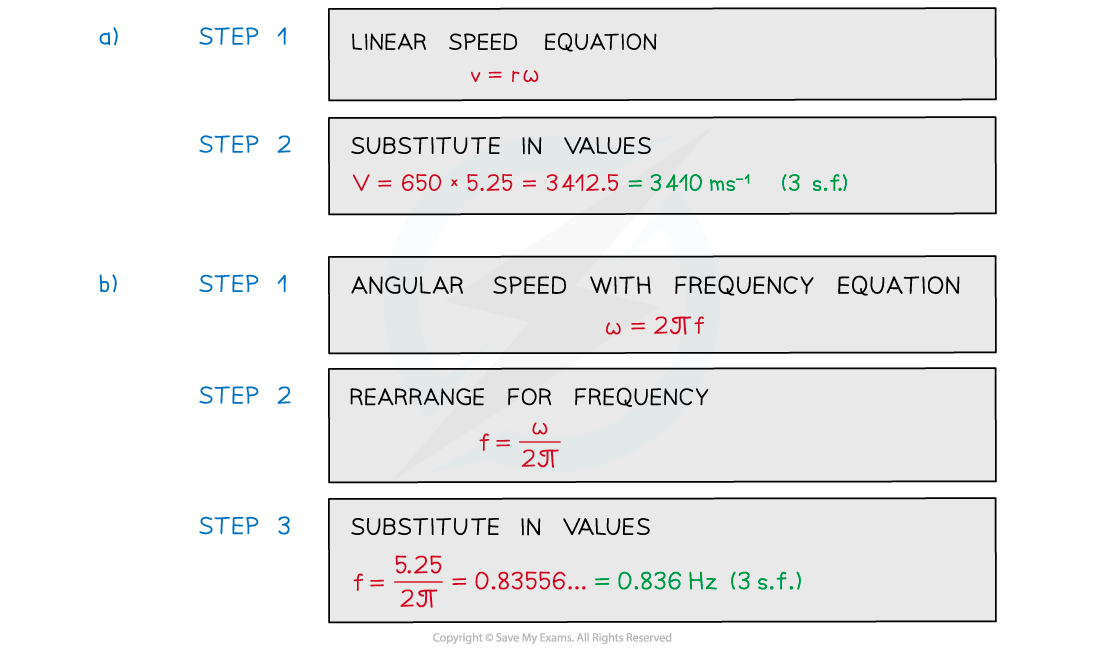
A bird flies in a horizontal circle with an angular speed of 5.25 rad s−1 of radius 650 m.
Calculate:
a) The linear speed of the bird
b) The angular frequency of the bird
Answer:
Examiner Tips and Tricks
Try not to be confused by similar sounding terms like "angular velocity" and "angular speed". Just like in regular linear motion, you have linear velocity and linear speed: one is a scalar (speed) and the other is a vector (velocity).
In this worked example, the equation v = rω is used to calculate the linear speed. This is fine, because v in this context is just the magnitude of the linear velocity (and similarly, ω is the magnitude of the angular velocity).
Finally, you may sometimes come across ω being labelled as 'angular frequency', because of its relationship to linear frequency f as given by the alternative equation ω = 2πf. Remember, the units of ω are rad s–1, whereas the units of f are Hz.

You've read 0 of your 5 free revision notes this week
Sign up now. It’s free!
Did this page help you?
