Phase & Path Difference (Edexcel International A Level (IAL) Physics) : Revision Note
Phase & Path Difference
Waves are said to be coherent if they have:
The same frequency
A constant phase difference
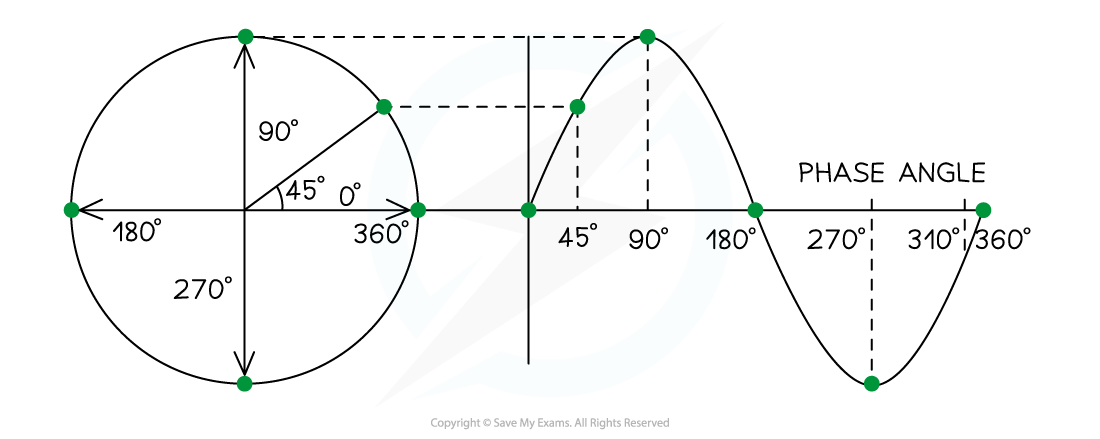
Phase Difference
Two points on a wave, or on different waves, are in phase when they are the same point in their wave cycle
The angle between their wave cycles is the phase difference
Path Difference
The type of interference occurring at a given point (i.e. constructive or destructive) depends on the path difference of the overlapping waves
Path difference is defined as:
The difference in distance travelled by two waves from their sources to the point where they meet
Path difference is generally expressed in multiples of wavelength
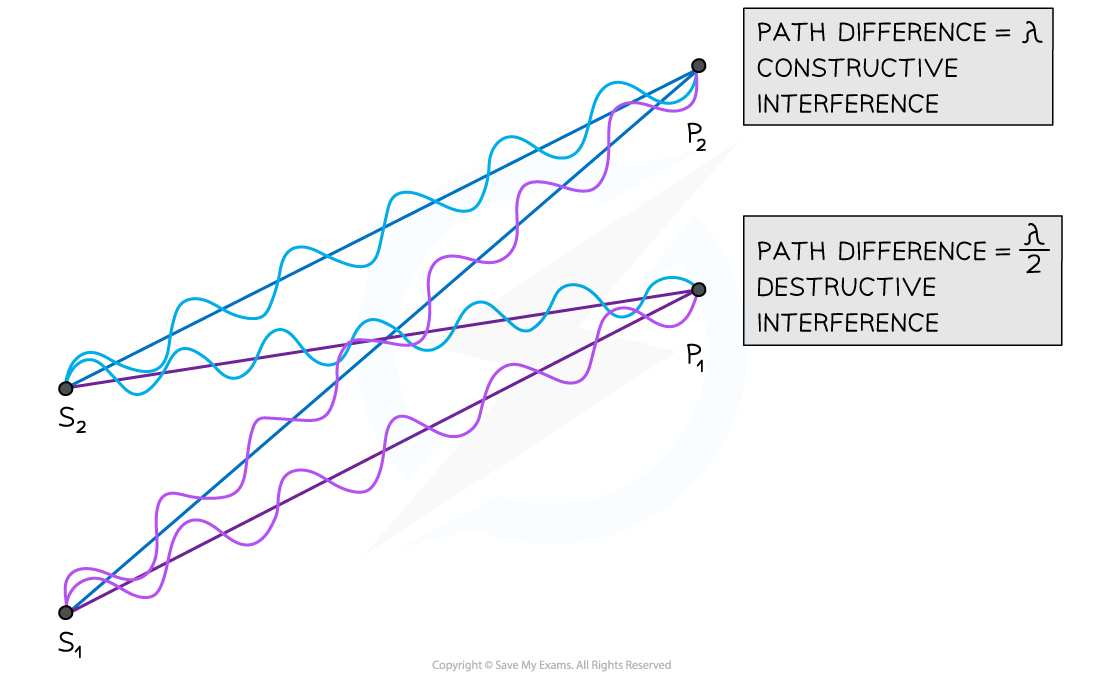
At point P2 the waves have a path difference of a whole number of wavelengths resulting in constructive interference. At point P1 the waves have a path difference of an odd number of half wavelengths resulting in destructive interference
In the diagram above, the number of wavelengths between:
S1 ➜ P1 = 6λ
S2 ➜ P1 = 6.5λ
S1 ➜ P2 = 7λ
S2 ➜ P2 = 6λ
The path difference at point P1 is 6.5λ – 6λ = λ / 2
The path difference at point P2 is 7λ – 6λ = λ
In general:
The condition for constructive interference is a path difference of nλ
The condition for destructive interference is a path difference of (n + ½)λ
In this case, n is an integer i.e. 0, 1, 2, 3...
Hence:
Destructive interference occurs at point P1
Constructive interference occurs at point P2
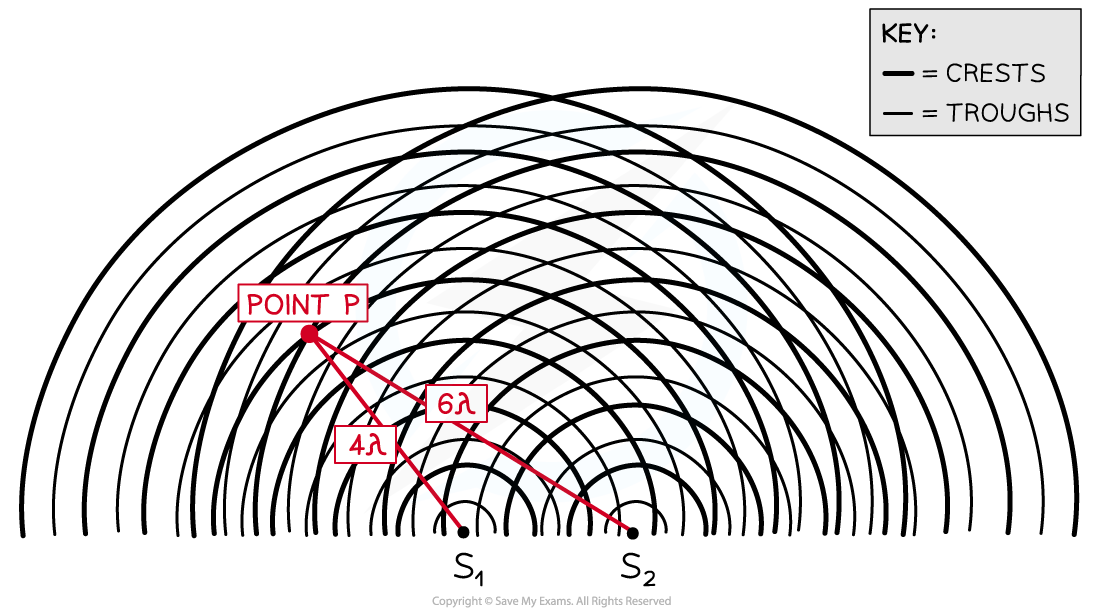
At point P the waves have a path difference of a whole number of wavelengths resulting in constructive interference
Another way to represent waves spreading out from two sources is shown in the diagram above
At point P, the number of crests from:
Source S1 = 4λ
Source S2 = 6λ
The path difference at P is 6λ – 4λ = 2λ
This is a whole number of wavelengths, hence constructive interference occurs at point P
Worked Example
The diagram shows the interferences of coherent waves from two point sources.
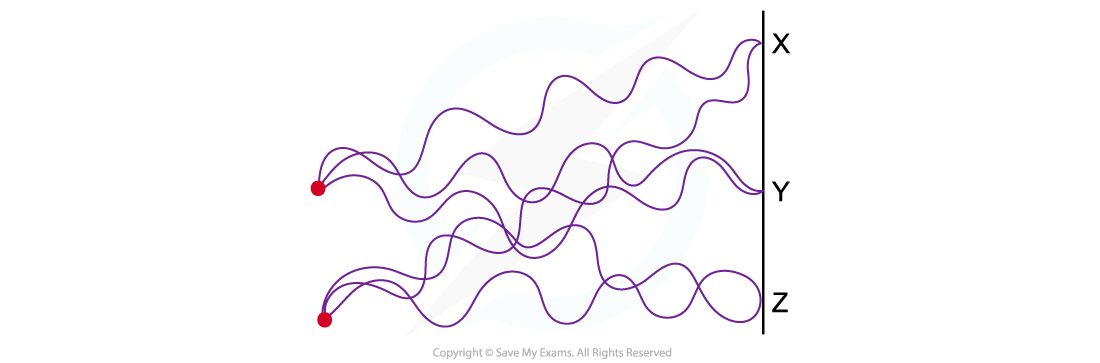
Which row in the table correctly identifies the type of interference at points X, Y and Z.
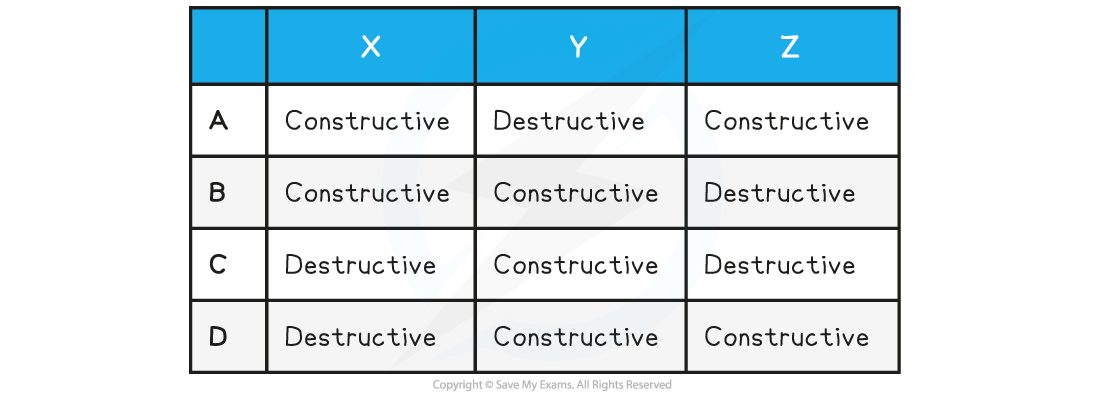
Answer: B
At point X:
Both peaks of the waves are overlapping
Path difference = 5.5λ – 4.5λ = λ
This is constructive interference and rules out options C and D
At point Y:
Both troughs are overlapping
Path difference = 3.5λ – 3.5λ = 0
Therefore constructive interference occurs
At point Z:
A peak of one of the waves meets the trough of the other
Path difference = 4λ – 3.5λ = λ / 2
This is destructive interference
Examiner Tips and Tricks
Phase difference and path difference are easy to confuse because the names sound similar. However they are very different concepts.
Phase difference tells us how far apart the waves are when comparing their phases (you can think of this as their peaks and troughs).
Path difference is how much further along one wave is than another. Think of it as how much further along the path it has travelled.

You've read 0 of your 5 free revision notes this week
Sign up now. It’s free!
Did this page help you?
