E.M.F. vs. Terminal Potential Difference (Edexcel International A Level (IAL) Physics) : Revision Note
E.M.F. vs. Terminal Potential Difference
Terminal Potential Difference
The terminal potential difference (p.d.) is the potential difference across the terminals of a cell
If there was no internal resistance, the terminal p.d. would be equal to the e.m.f.
If a cell has internal resistance, the terminal p.d. is always lower than the e.m.f.
If you have a load resistor R across the cell's terminals, then the terminal p.d. is also the potential difference across the load resistor
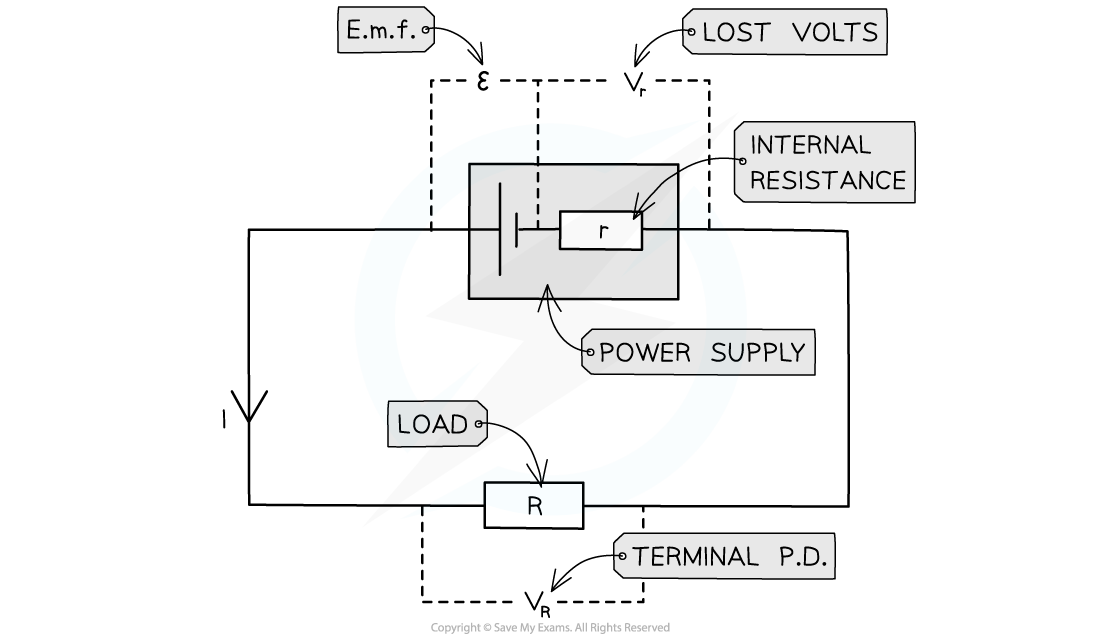
Circuit showing the e.m.f. and internal resistance of a power supply
Where:
Resistor R is the ‘load resistor’
r is the internal resistance
ε is the e.m.f.
Vr is the lost volts
VR is the p.d across the load resistor, which is the same as the terminal p.d.
Terminal potential difference is the voltage available to the rest of the circuit
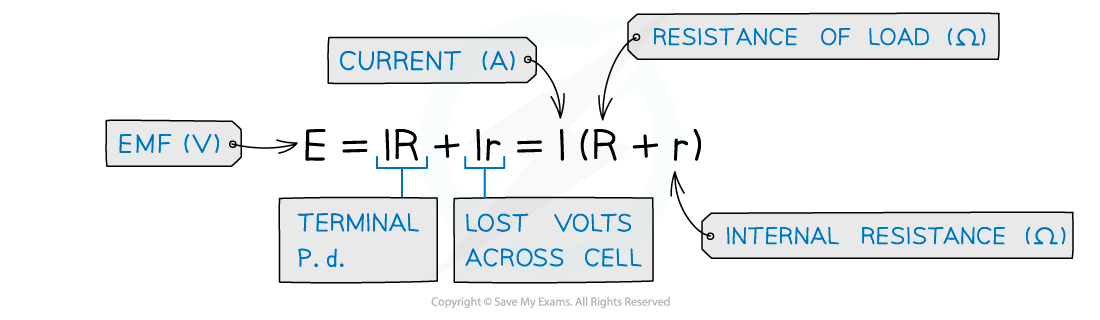
VR = I × R ( from Ohm’s law)
Where:
R = load resistance (Ω)
I = current in the circuit (A)
VR = Terminal p.d. (V)
When a load resistor is connected, current flows through the cell and a potential difference develops across the internal resistance.
This voltage is not available to the rest of the circuit so is called the ‘lost volts’
Vr is the lost volts
This is the voltage lost in the cell due to internal resistance
Vr = I × r (from Ohm’s law)
Where:
r = internal resistance (Ω)
I = current in the circuit (A)
Vr = Lost volts (V)
The e.m.f. is the sum of the terminal p.d. and the lost volts:
The Difference Between Potential Difference and E.M.F
The difference between potential difference and e.m.f is the type of energy transfer per unit charge

When charge passes through a resistor, for example, its electrical energy is converted to heat in the resistor
The resistor, therefore, has a potential difference across it
Potential difference describes the loss of energy from charges
Ie. when electrical energy is transferred to other forms of energy in a component
E.m.f. describes the transfer of energy from the power supply to electrical charges within the circuit
Worked Example
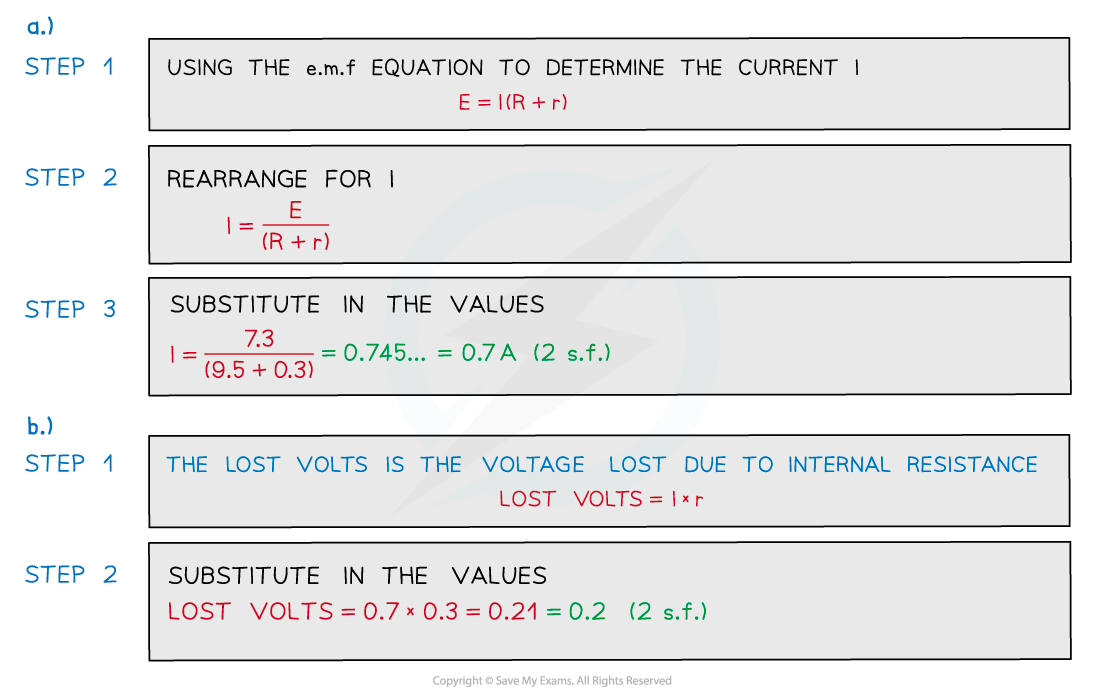
A battery of e.m.f. 7.3 V and internal resistance r of 0.3 Ω is connected in series with a resistor of resistance 9.5 Ω.

Determine:
a) The current in the circuit
b) Lost volts from the battery
Answer:
Examiner Tips and Tricks
If the exam question states 'a battery of negligible internal resistance', this assumes that e.m.f. of the battery is equal to its voltage. Internal resistance calculations will not be needed here. If the battery in the circuit diagram includes internal resistance, then the e.m.f. equations must be used.

You've read 0 of your 5 free revision notes this week
Sign up now. It’s free!
Did this page help you?
