Hooke's Law (Edexcel International A Level (IAL) Physics): Revision Note
Exam code: YPH11
Hooke's Law
When a force F is added to the bottom of a vertical metal wire of length L, the wire stretches
A material obeys Hooke’s Law if:
The extension of the material is directly proportional to the applied force (load) up to the limit of proportionality
This linear relationship is represented by the Hooke’s law equation:
ΔF = kΔx
Where:
F = applied force (N)
k = spring constant (N m–1)
Δx = extension (m)
The spring constant is a property of the material being stretched and measures the stiffness of a material
The larger the spring constant, the stiffer the material
Hooke's Law applies to both extensions and compressions:
The extension of an object is determined by how much it has increased in length
The compression of an object is determined by how much it has decreased in length
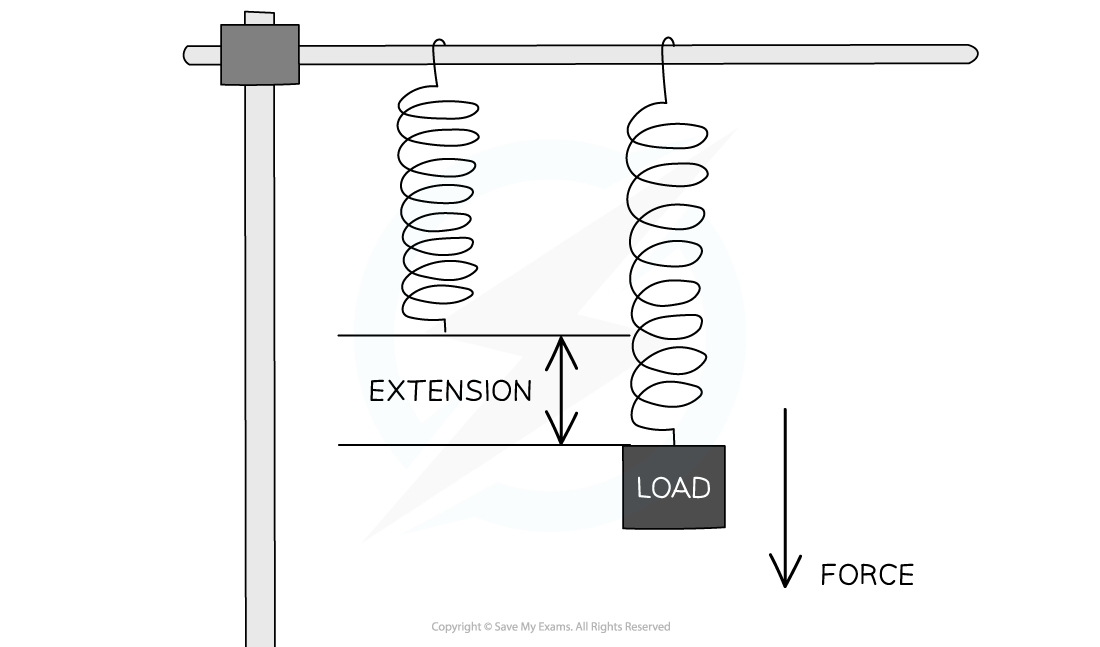
Stretching a spring with a load produces a force that leads to an extension
Force–Extension Graphs
The way a material responds to a given force can be shown on a force-extension graph
A material may obey Hooke's Law up to a point
This is shown on its force-extension graph by a straight line through the origin
As more force is added, the graph may start to curve slightly
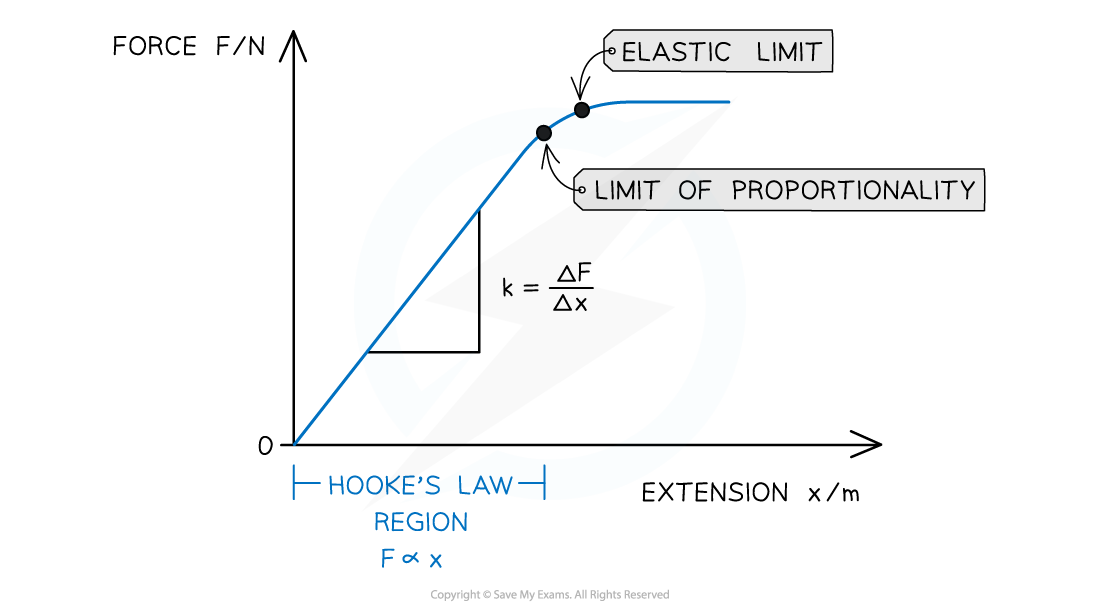
The Hooke's Law region of a force-extension graph is a straight line. The spring constant is the gradient of that region
The key features of the graph are:
The limit of proportionality: The point beyond which Hooke's law is no longer true when stretching a material i.e. the extension is no longer proportional to the applied force
The point is identified on the graph where the line starts to curve (flattens out)
Elastic limit: The maximum amount a material can be stretched and still return to its original length (above which the material will no longer be elastic). This point is always after the limit of proportionality
The gradient of this graph is equal to the spring constant k
Worked Example
A spring was stretched with increasing load.
The graph of the results is shown below.
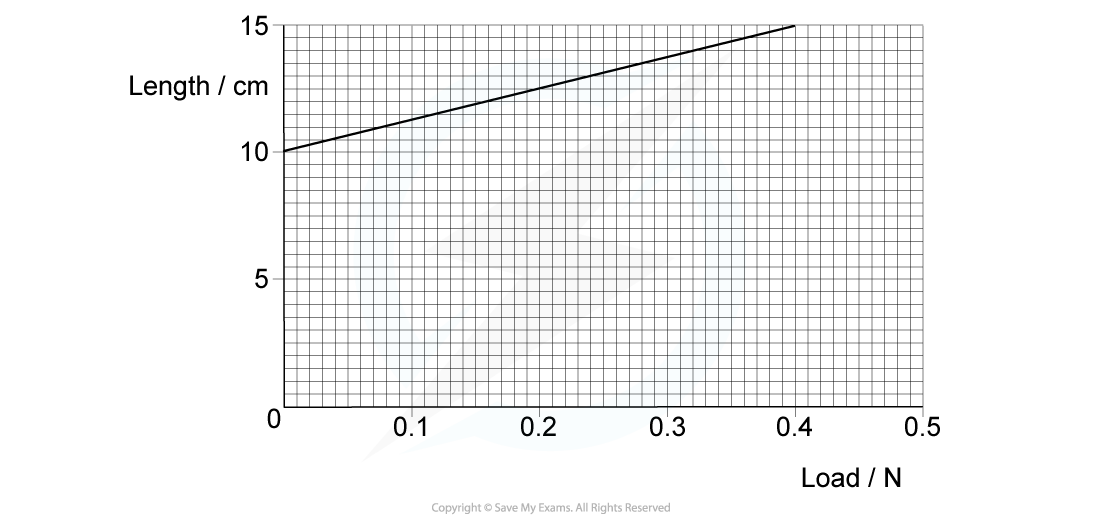
What is the spring constant?
Answer:
Step 1: Rearrange Hooke's Law to make the spring constant the subject
Step 2: Compare the gradient to the equation in Step 1
This graph is length - extension, so the gradient gives:
Therefore k is the reciprocal of the gradient
Step 3: Find the gradient
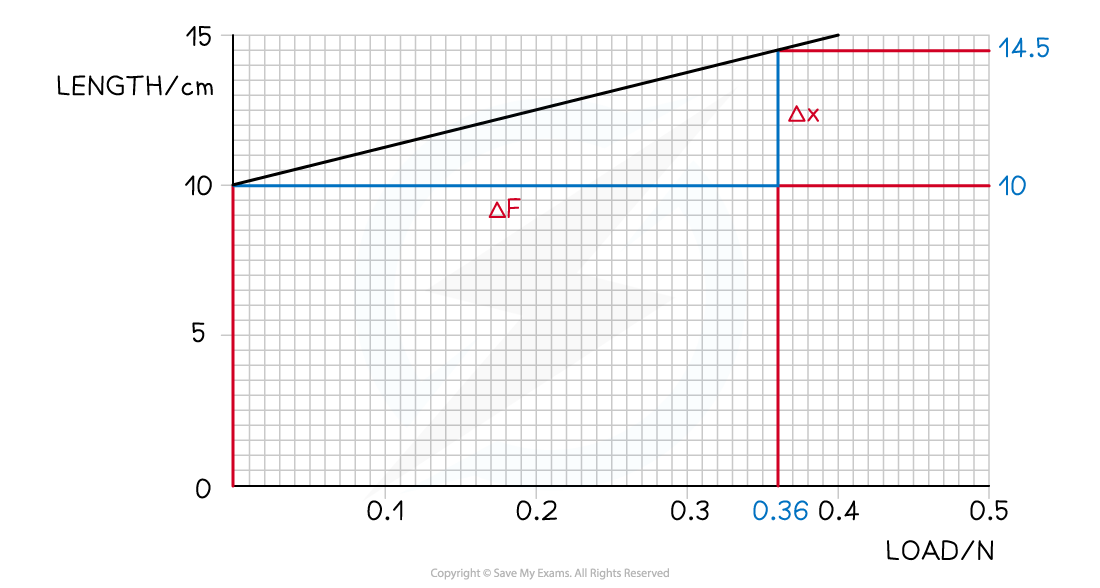
Step 4: Calculate gradient
Step 5: Calculate the spring constant by finding the reciprocal of the gradient
Step 6: Write the answer, including units
Spring constant, k = 8.0 N m−1
Examiner Tips and Tricks
Always double check the axes before finding the spring constant as the gradient of a force-extension graph. Exam questions often swap the force (or load) onto the x-axis and extension (or length) on the y-axis.
In this case, the gradient is not the spring constant, it is 1 ÷ gradient instead.

Unlock more, it's free!
Did this page help you?