Revision NotesExam QuestionsPast Papers
International A LevelPhysicsEdexcelRevision Notes1. Mechanics & MaterialsMotionMotion Graphs

Motion Graphs (Edexcel International A Level Physics)

Revision Note

Lindsay Gilmour

Last updated

Motion Graphs

  • Three types of graph that are used to represent motion are displacement-time graphs, velocity-time graphs and acceleration-time graphs

  • Graphs are named 'y-axis - x-axis', so 'displacement-time' simply means 'displacement on the y-axis and time on the x-axis'

  • Graphs of motion can be thought of as telling a story of something which has happened in the real world, such as

    • the time, direction and average speed of a journey to school

    • initial and final velocity and acceleration as a rocket takes off from the launchpad

    • movement and speed in various directions as an athlete takes part in an event

  • Interpreting graphs means using the slope of the line, or the area under the line to find information about that 'story'

  • Drawing graphs is a skill where careful planning, plotting and line drawing from some data yields further information from an investigation

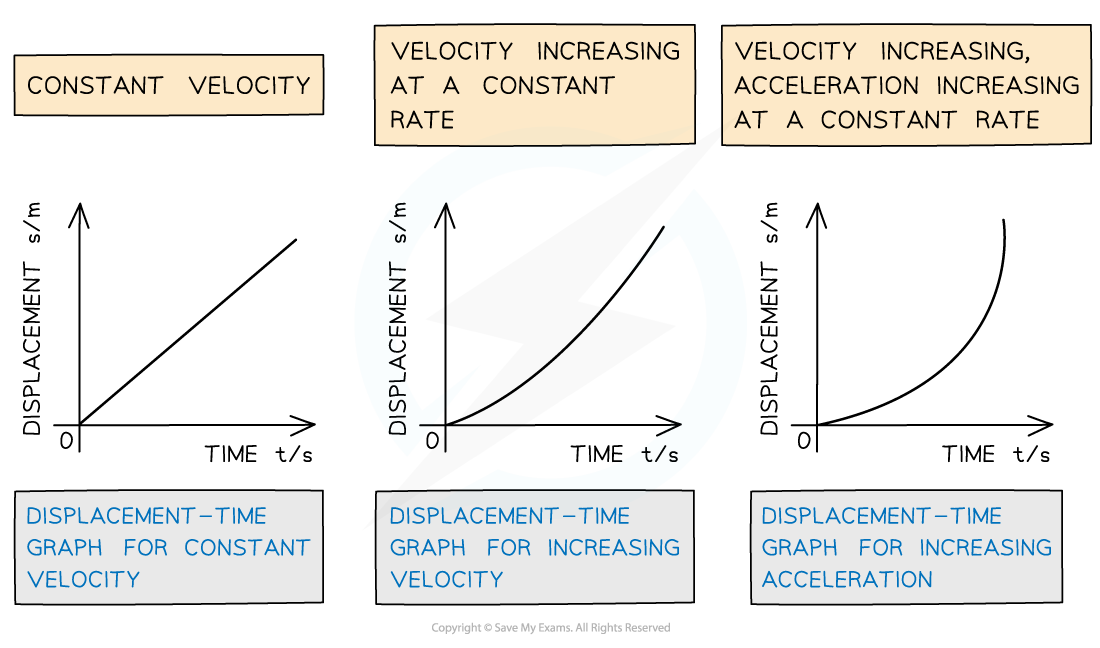
Displacement-Time

  • On a displacement-time graph;

    • Slope equals velocity

      • A straight (diagonal) slope represents a constant velocity

      • A curved slope represents an acceleration

    • A positive slope represents motion in the positive direction

    • A negative slope represents motion in the negative direction

    • A zero slope (horizontal line) represents a state of rest

    • The area under the curve is meaningless

Motion graphs (1), downloadable AS & A Level Physics revision notes

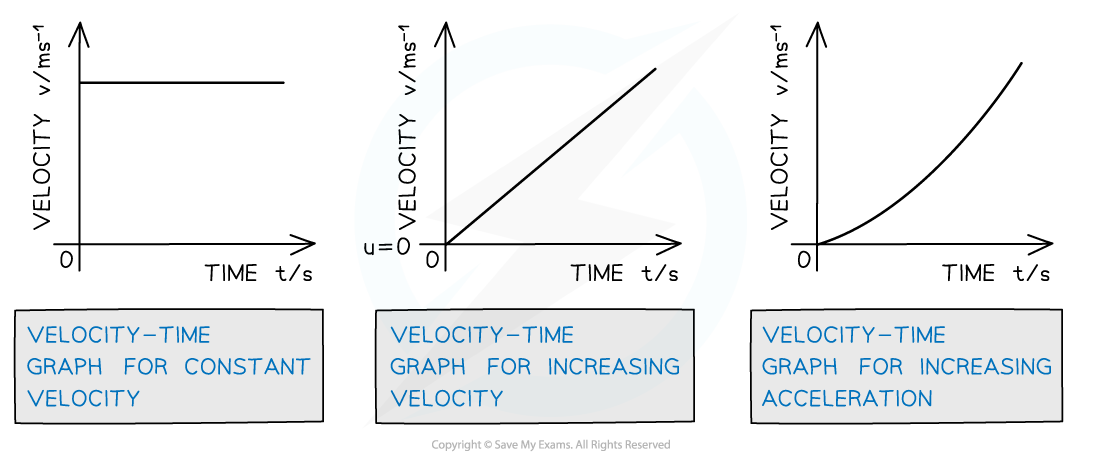
Velocity-Time

  • On a velocity-time graph…

    • Slope equals acceleration

      • A straight line represents uniform acceleration

      • A curved line represents non-uniform acceleration

    • A positive slope represents an increase in velocity in the positive direction

    • A negative slope represents an increase in velocity in the negative direction

    • a zero slope (horizontal line) represents motion with constant velocity

    • The area under the curve equals the displacement or distance travelled

Motion graphs (2), downloadable AS & A Level Physics revision notes

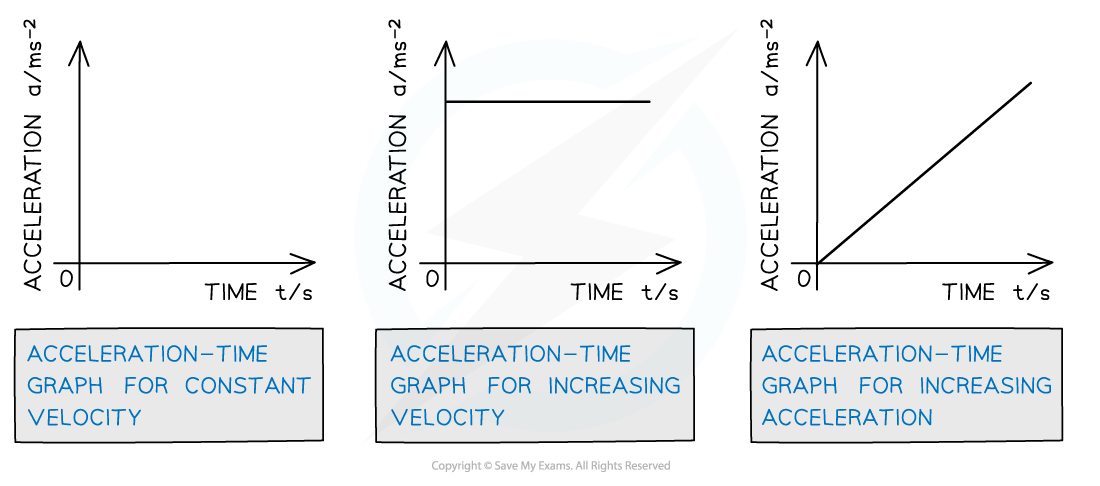
Acceleration-Time

  • On an acceleration-time graph…

  • A zero slope (horizontal line) represents an object undergoing constant acceleration

  • The area under the curve equals the change in velocity

  • The steepness of the slope is meaningless

Motion graphs (3), downloadable AS & A Level Physics revision notes

How displacement, velocity and acceleration graphs relate to each other

Drawing Graphs

  • Drawing a good graph is an important skill

  • Good graphs have the following:

    • Each axis has two labels; the name of the variable and the unit (and may include a multiplier, for  example, m × 10−9)

    • Values on the axes are evenly spaced, with the same number of divisions for each amount

      2-2-motion-graphs-drawing-graphs-1-of-3_edexcel-al-physics-rn

    • The axes start at the origin only when this fits the data or the relationship being graphed is directly proportional or when specified in the question

    • Increments on the scale are in multiples of 2, 5 and 10 but never multiples of 3.

    • The range of values being plotted takes up the length of the axis, so that the plotted line takes up most of the space on the graph

    • Plots are accurate to within half a small square

    • Plots and curves are drawn with sharp pencil so that they are narrow (don't take up more than half a small square)

      2-2-motion-graphs-drawing-graphs-2-of-3_edexcel-al-physics-rn

    • Lines of best fit match the data, so that lines go through as many of the plots as possible (ignoring outliers) with the remainder evenly spaced above and below the line

2-2-motion-graphs-drawing-graphs-3-of-3_edexcel-al-physics-rn

Worked Example

A runner is practicing short sprints between two markers. A displacement-time graph showing part of their training is shown.

2-2-motion-graphs-we1-qn_edexcel-al-physics-rn

(a)   Find the distance between the markers

(b)   Determine the speed of the runner at the fastest part of their sprint

Answer:

Part (a)

Step 1: Determine the direction of motion from the graph

  • Initially displacement increases from 0 - 50 m

  • This represents the sprinter running away from the starting position

  • Secondly displacement decreases from 50 - 0 m

  • This represents the sprinter returning towards the starting position

Step 2: Identify the sprinter's turning point on the graph 

  • The distance between the markers is from 0 → turning around point

  • This is 50 m from the start point

Step 3: Write the correct answer

Distance between the markers = 50 m

  Part (b)

Step 1: Identify the part of the graph that represents the velocity

  • The graph shows displacement against time

  • Velocity is equal to:

V e l o c i t y equals fraction numerator d i s p l a c e m e n t over denominator t i m e end fraction

  • Therefore, the slope of the displacement-time graph gives velocity

  • Displacement × time (area under the graph) is not a physical quantity

Step 2: Identify the steepest section of the graph

  • Select the section on the graph where the slope is the steepest

    • This represents the fastest velocity

    • Clearly mark two points on this line which are as far apart as possible 

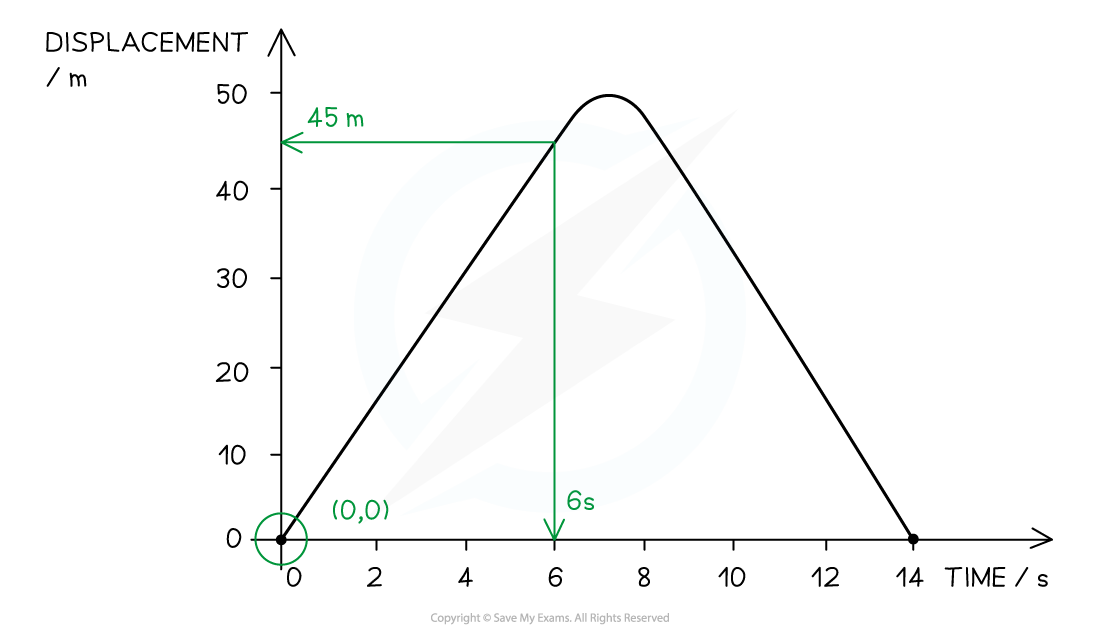
2-2-motion-graphs-we1-ans_edexcel-al-physics-rn

Step 3: Use the coordinates from the graph to calculate the slope

slope = fraction numerator i n c r e a s e space i n space y over denominator i n c r e a s e space i n space x end fraction space equals space 45 over 6 space= 7.5 m s−1

Step 4: Write down the answer to the correct number of significant figures

The sprinter's fastest speed = 7.5 m s−1

Worked Example

Motion has been plotted on a displacement-time graph.

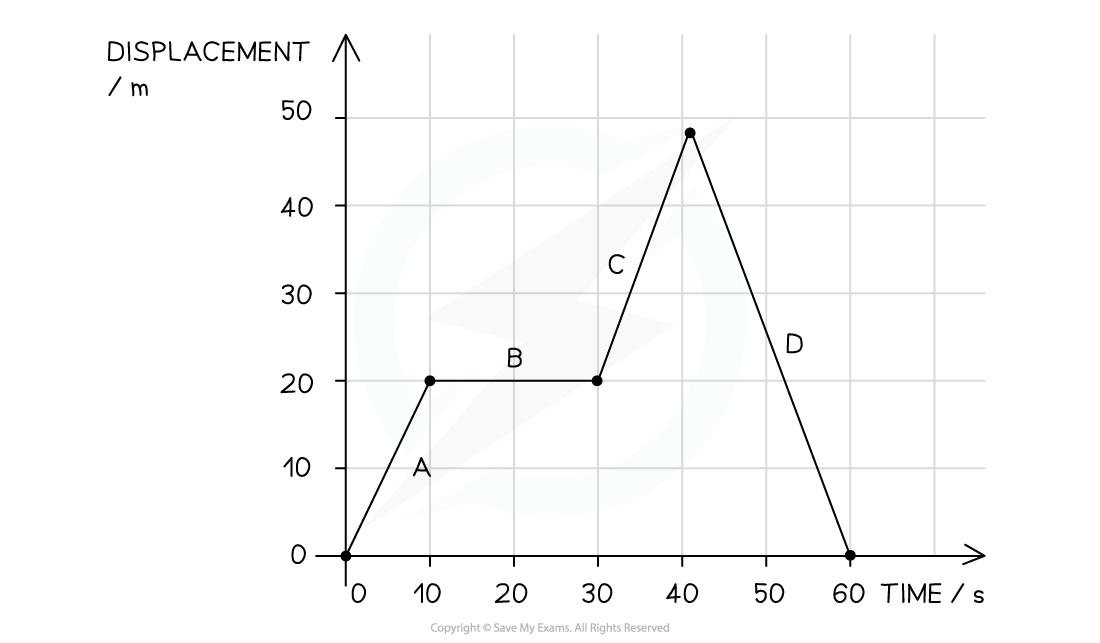
2-2-motion-graphs-we2-qn_edexcel-al-physics-rn

Sketch a velocity-time graph for this motion. Include values and show your calculations.

Answer:

Step 1: Calculate each stage:

Part A

Step 1: Determine the time interval

Time from 0 - 10 s

Step 2: Calculate the velocity by finding the gradient

Gradient = fraction numerator capital delta y over denominator capital delta x end fraction = 20 over 10 = 2 m s−1

Part B

Step 1: Determine the time interval

Time from 10 - 30 s

Step 2: Calculate the velocity by finding the gradient

Gradient = 0 (horizontal line), velocity = 0 m s−1

Part C

Step 1: Determine the time interval

Time from 30 - 40 s

Step 2: Calculate the velocity by finding the gradient

Gradient = fraction numerator capital delta y over denominator capital delta x end fraction = fraction numerator 50 space minus space 20 over denominator 40 space minus space 30 end fraction = 3 m s−1

Part D

Step 1: Determine the time interval

Time from 40 - 60 s

Step 2: Calculate the velocity by finding the gradient

Gradient = fraction numerator capital delta y over denominator capital delta x end fraction = fraction numerator 0 space minus space 50 over denominator 60 space minus space 40 end fraction = − 2.5 m s−1

Step 2: Use the calculated values to sketch a graph

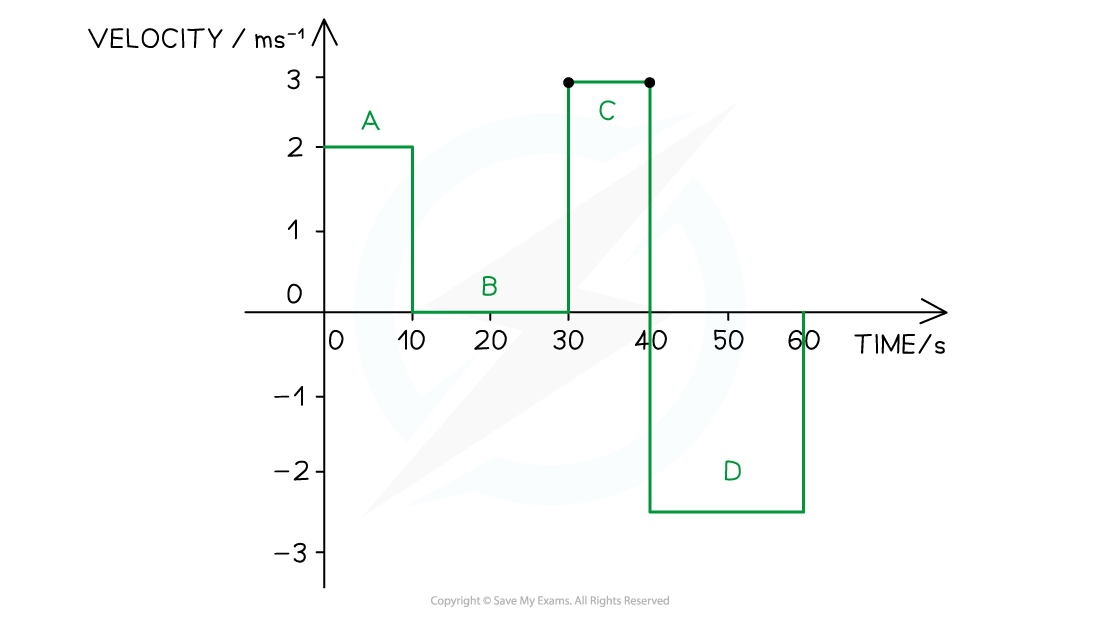
2-2-motion-graphs-we2-ans_edexcel-al-physics-rn

Examiner Tips and Tricks

Interpreting Graphs

It's common for a question to ask you to solve something 'using a graphical method'. This just means 'by finding your answer on a graph'.

In AS Physics most (but not all) graphs will be a straight line, meaning they follow the equation y = mx + c

This means that the 'graphical method' question is asking you to look at the gradient, or the area under the line. Once you are secure in finding these then graph questions will become much easier!

Drawing Graphs

A well-drawn graph will net you four or five quick and easy marks IF you have practiced the skill.

Common mistakes are starting at the origin when there is no need (so the line takes up less than half of the page) and trying to work with a scale that goes in multiples of three (making it hard to get the plotting right).

You've read 0 of your 5 free revision notes this week

Sign up now. It’s free!

Join the 100,000+ Students that ❤️ Save My Exams

the (exam) results speak for themselves:

    Test yourself

    Did this page help you?

    Previous:Equations of MotionNext:Slope & Area of Motion Graphs
    Lindsay Gilmour

    Author: Lindsay Gilmour

    Expertise: Physics

    Lindsay graduated with First Class Honours from the University of Greenwich and earned her Science Communication MSc at Imperial College London. Now with many years’ experience as a Head of Physics and Examiner for A Level and IGCSE Physics (and Biology!), her love of communicating, educating and Physics has brought her to Save My Exams where she hopes to help as many students as possible on their next steps.