Equations of Motion (Edexcel International A Level Physics)

• The kinematic equations of motion are a set of four equations which can describe any object moving with constant acceleration
• They are often referred to as the 'suvat' equations due to the variables they include
• These are:
  • s = displacement (m)
  • u = initial velocity (m s⁻¹)
  • v = final velocity (m s⁻¹)
  • a = acceleration (m s⁻²)
  • t = time interval (s)

• The four SUVAT equations are:

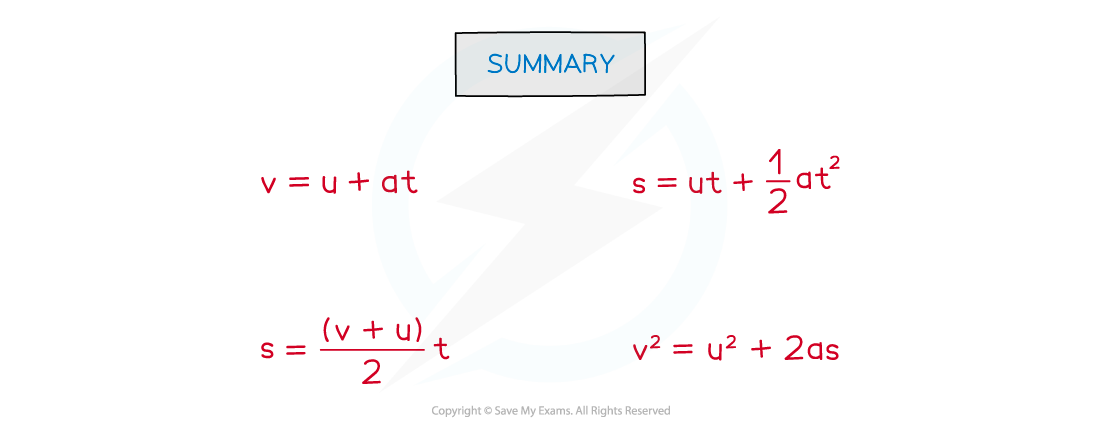
• These are all given on the data sheet
• All the variables, apart from time t, are vector quantities
  • This means they can either be positive or negative depending on their direction

Mastering the SUVAT Equations

• The SUVAT equations are always used when acceleration is constant, but not zero

• This is indicated in questions by
  • An explicit statement that an object is 'constantly accelerating' or 'constantly decelerating', or words to that effect
  • A description of an object in freefall where either 'air resistance can be ignored' or 'air resistance is negligible'
  • A statement that an accelerating or decelerating force is constant, since F = ma, constant force → constant acceleration

• Questions may appear to be missing some information, this should be checked before starting to calculate
  • Objects in motion often start or end at rest, making either u or v = zero
  • Objects in freefall have a constant acceleration of 9.81 m s⁻²
  • When an object is slowing down it has a negative value for acceleration, this must be included in the calculation
  • Objects thrown or shot upwards also have negative acceleration (since they are slowing down as they ascend) and reach a final velocity of zero at the top of their path

Step 1: List the known values

• • Length of the runway, s_max = 450 m (this is the maximum value of displacement)
  • Initial velocity, u = 40 m s⁻¹
  • Final velocity, v = 0 m s⁻¹ (since the plane will stop)
  • Acceleration, a = − 2 m s⁻² (the negative sign indicates slowing down)

Step 2: Identify the missing values (the unknown answer and the one not given)

• • Known quantities: u, v and a
  • Unknown quantity: displacement, s
  • Time, t is not required in this question

Step 3: Choose the correct SUVAT equation and rearrange it

• • The SUVAT equation which contains u, v, a and s and omits t is:

v² = u² + 2as

• • Rearrange to make s the subject:

Step 4: Substitute the values into the equation and calculate s

= 400 m

Step 5: Write the full answer to the question

• • The plane stops after 400 m, which is 50 m before the end of the runway

• • Note: In this solution, the negative value produced by v² − u² is cancelled out by the negative value for acceleration, giving a positive value for displacement

Part (a)

Step 1: List the known values

• • Initial velocity, u = 0 m s⁻¹
  • Acceleration, a = 6.5 m s⁻² (the positive sign indicates the speed is increasing due to the motor)
  • Time, t = 30 s

Step 2: Identify the missing values (the unknown answer and the one not given)

• • Known quantities: u, a, t
  • Unknown quantity: final velocity, v
  • Displacement, s, is not required in this question

Step 3: Choose the correct SUVAT equation

• • The SUVAT equation which omits displacement, s, is:

v = u + at

Step 4: Substitute the values into the equation and calculate

v = 0 + (6.5 × 30) = 195 m s⁻¹

Step 5: Write the full answer to the question, taking care to check significant figures

• • The final velocity, v, at 30 s is 200 m s⁻¹ (2 s.f.)

Part (b)

Step 1: List the known values

• • Initial velocity, u = 0 m s⁻¹
  • Acceleration, a = 6.5 m s⁻² 
  • Time, t = 30 s
  • Final velocity, v = 195 m s⁻¹

Step 2: Identify the missing values (the unknown answer and the one not given)

• • Known quantities: u, a, t, and v (from part a)
  • Unknown quantity: final displacement, s
  • All the SUVAT values are available in this case, but since v was calculated, first look for an equation that doesn't use it (in case of mistakes)

Step 3: Choose the correct SUVAT equation

• • The SUVAT equation which omits velocity is:

s = ut + at²

Step 4: Substitute the values into the equation and calculate s

s = (0 × 30) + (6.5 × 30²) = 2925 m

Step 5: Write the full answer to the question, taking care to check significant figures

• • The height, s, above the launchpad is 2900 m (2 s.f)