Conservation of Linear Momentum (Edexcel International A Level (IAL) Physics) : Revision Note
The Principle of Conservation of Linear Momentum
The principle of conservation of momentum states that in a closed system, the total momentum before an event is equal to the total momentum after the event
Momentum is always conserved in collisions where no external forces act
This is usually written as:
Total momentum before a collision = Total momentum after a collision
Since momentum is a vector quantity, a system of objects moving in opposite directions can have an overall momentum of 0
This applies to objects moving towards each other or away from each other
The diagram below shows two masses m with velocity u and M at rest (M has zero velocity)
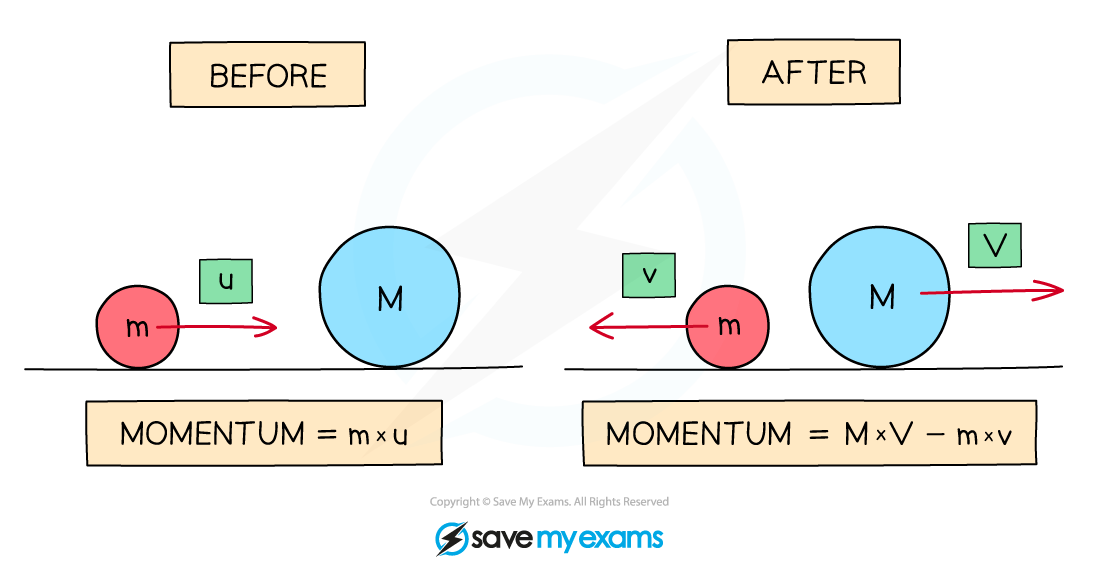
The momentum of a system before and after a collision
Before the collision:
The momentum is only of mass m which is moving
If the right is taken as the positive direction, the total momentum of the system is m × u
After the collision:
Mass M also now has momentum
The velocity of m is now -v (since it is now travelling to the left) and the velocity of M is V
The total momentum is now the momentum of M + momentum of m
This is (M × V) + (m × -v) or (M × V) – (m × v)
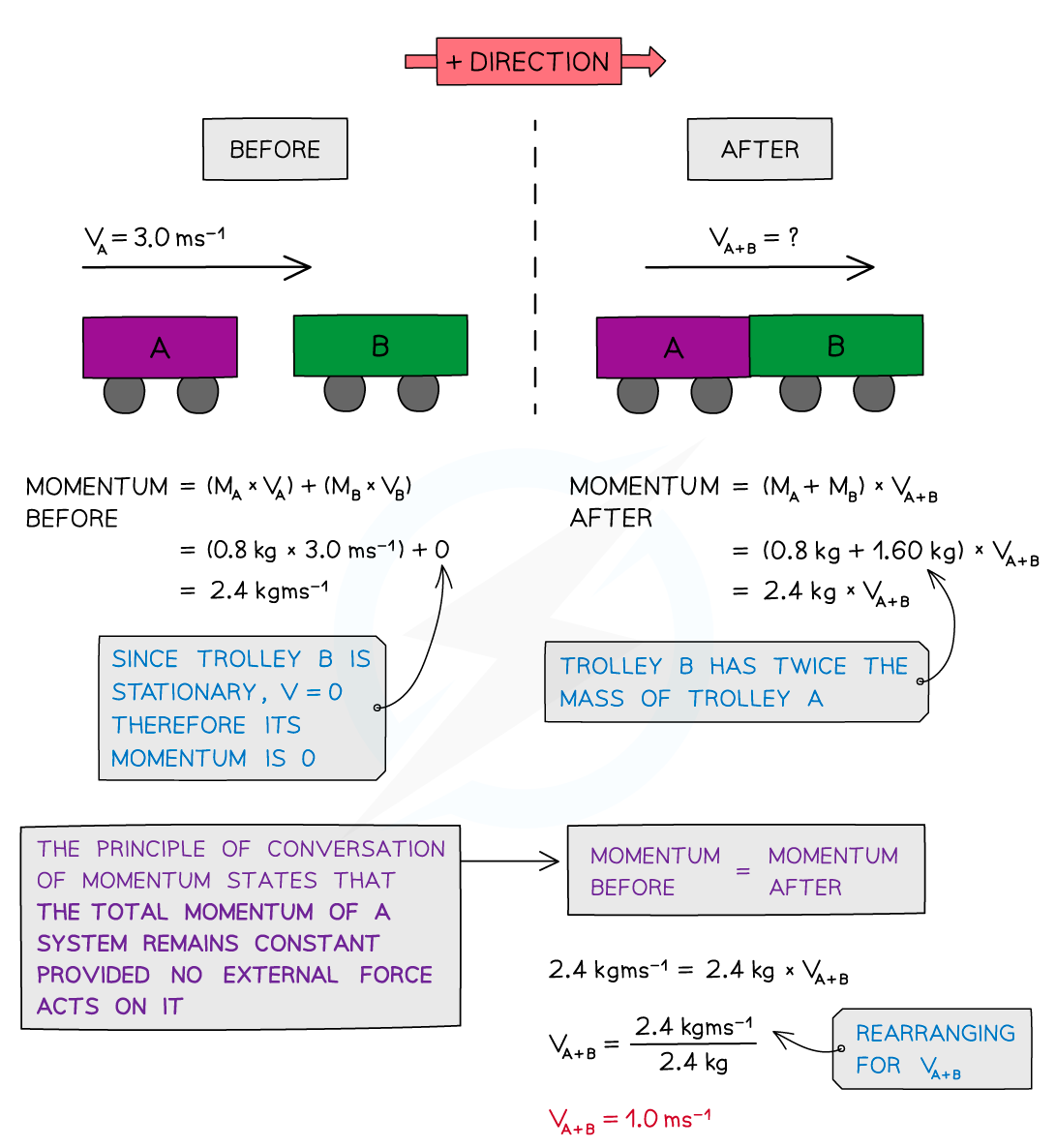
Worked Example
Trolley A of mass 0.80 kg collides head-on with stationary trolley B whilst travelling at
3.0 m s–1. Trolley B has twice the mass of trolley A. On impact, the trolleys stick together.
Using the conversation of momentum, calculate the common velocity of both trolleys after the collision.
Answer:
Conservation of Linear Momentum & Newton's Third Law
Newton’s third law of motion states:
Whenever two bodies interact, the forces they exert on each other are equal and opposite
This means:
When one object exerts a force on another object, the second object will exert an equal force on the first object in the opposite direction
When two objects collide, both objects will react, generally causing one object to speed up (gain momentum) and the other object to slow down (lose momentum)
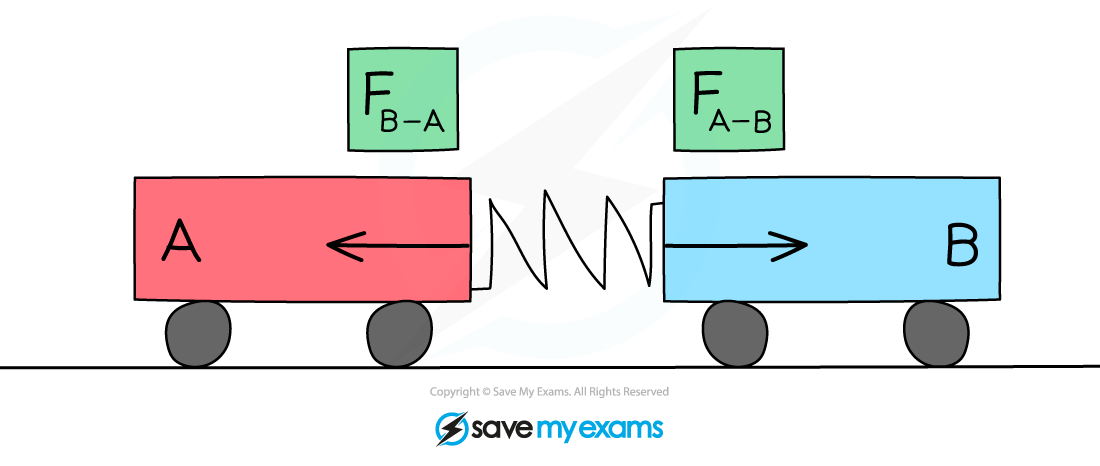
Newton's third law can be applied to collisions
Consider the collision between two trolleys, A and B:
When trolley A exerts a force on trolley B, trolley B will exert an equal force on trolley A in the opposite direction
In this case:
FB–A = –FA–B
While the forces are equal in magnitude and opposite in direction, the accelerations of the objects are not necessarily equal in magnitude
From Newton's second law, acceleration depends upon both force and mass, this means:
For objects of equal mass, they will have equal accelerations
For objects of unequal mass, they will have unequal accelerations
Examiner Tips and Tricks
Momentum questions are often very long and wordy. Even if you are given a diagram, make a quick sketch representing all the bodies as point masses. Mark the velocities with arrows and include positive and negative signs.
The Maths of momentum is straightforward, so as long as you have your vector directions clear in your mind, nothing can go wrong!

You've read 0 of your 5 free revision notes this week
Sign up now. It’s free!
Did this page help you?
