Calculating Poisson Probabilities

Throughout this section we will use the random variable $X ~ Po (λ)$ . For a Poisson distribution, the probability of a X taking a non-integer or negative value is always zero. Therefore any values mentioned in this section (other than λ) will be assumed to be non-negative integers.

Where does the formula for a Poisson distribution come from?

The formula for calculating an individual Poisson probability is
- $P (X = r) = p_{r} = e^{- λ} \times \frac{λ^{r}}{r!}$ for r = 0, 1, 2 ,...., n
The derivation of the formula comes from the binomial distribution, however it is outside the scope of this syllabus and will not be proved here
- Whilst the binomial distribution relies on knowing a fixed number of trials, the Poisson can allow for any number of trials within a time period
- Only the mean number of occurrences of an event within the given period needs to be known

How do I calculate the cumulative probabilities for a Poisson distribution?

Most of the time the value of λ will be in the table for the 'Poisson Cumulative Distribution Function' in the formula booklet
$P (X \leq x)$ is asking you to find the probabilities of all values up to and including x
- This will be the value in the row of x
$P (X < x)$ is asking you to find the probabilities of all values up to but not including x
- This means all values that are less than x
- This will be the value in the row of x - 1
  - $P (X \leq x - 1)$
$P (X \geq x)$ is asking you to find the probabilities of all values greater than and including x
- This means all values that are at least x
- As there is not a fixed number of trials this includes an infinite number of possibilities
- To calculate this, use the identity $P (X \geq x) = 1 - P (X < x)$
  - Using above we get: $P (X \geq x) = 1 - P (X \leq x - 1)$
$P (X > x)$ is asking you to find the probabilities of all values greater than but not including x
- This means all values that are more than x
- Rewrite $P (X > x) = 1 - P (X \leq x)$ as to calculate this
If calculating $P (a \leq X \leq b)$ then calculate $P (X \leq b) - P (X \leq a - 1)$
- This is the same idea as for the binomial distribution

How do I calculate the cumulative probabilities for a Poisson distribution if λ is not in the table?

In the unlikely case that λ is not in the table
- Use the formula to find the individual probabilities and then add them up
- Make sure you are confident working with inequalities for discrete values
- Only integer values will be included so it is easiest to look at which integer values you should include within your calculation
- Sometimes it is quicker to find the probabilities that are not being asked for and subtract from one

Having to type a lot of calculations into your calculator can be time consuming and cause errors
Consider the calculation $P (X \leq 3) = e^{- λ} \times \frac{λ^{0}}{0!} + e^{- λ} \times \frac{λ^{1}}{1!} + e^{- λ} \times \frac{λ^{2}}{2!} + e^{- λ} \times \frac{λ^{3}}{3!}$
- Note that $e^{- λ}$ exists in every term and can be factorised out
- Recall that $λ^{0} = 1$ and 0! = 1
- Recall also that $λ^{1} = λ$ and 1! = 1
This calculation could be factorised and simplified to
- $P (X \leq 3) = e^{- λ} (1 + λ + \frac{λ^{2}}{2!} + \frac{λ^{3}}{3!})$
- This is much simpler and easier to type into your calculator in exam conditions

How do I change the mean for a Poisson distribution?

Sometimes the mean may be given for a different interval of time or space than that which you need to calculate the probability for
A given value of λ can be adjusted to fit the necessary time period
- For example if a football team score a mean of 2 goals an hour and we want to find the probability of them scoring a certain number of goals in a 90 minute game, then we would use the distribution X ~ Po(3)
  - 90 = 1.5 × 60 so use 1.5λ

Worked example

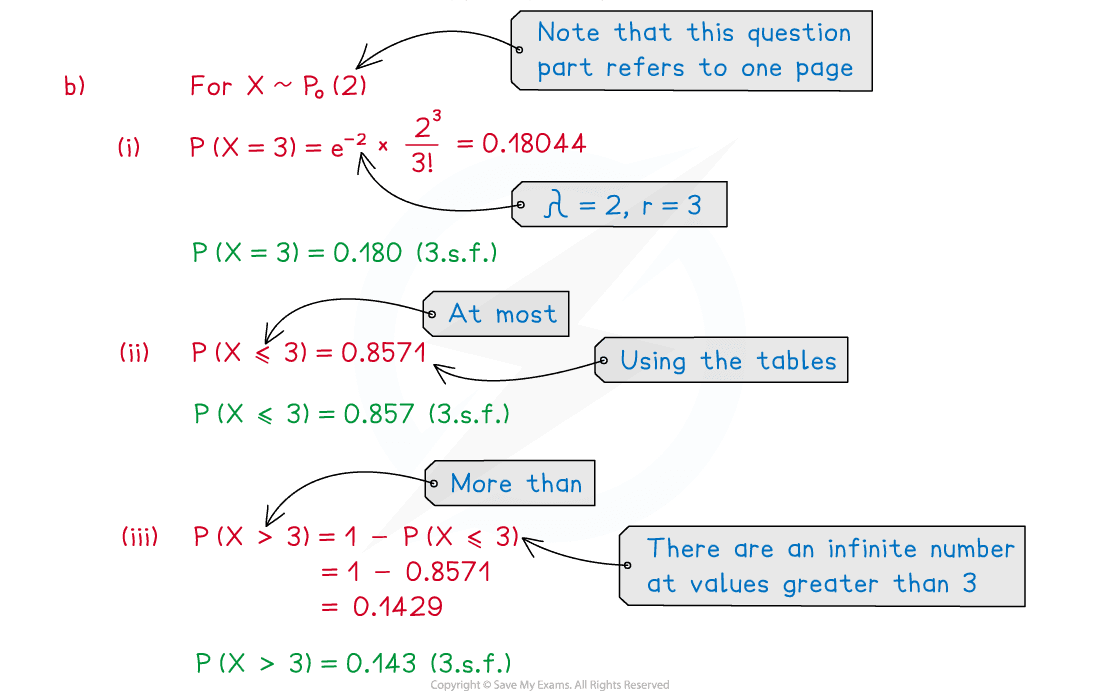
Xiao makes silly mistakes in his maths homework at a mean rate of 2 per page.

(a)

Define a suitable distribution to model the number of silly mistakes Xiao would make in a piece of homework that is five pages long.

(b)

Find the probability that in any random page of Xiao’s homework book there are

(i)

exactly three silly mistakes

(ii)

at most three silly mistakes

(iii)

more than three silly mistakes.

(a)

Define a suitable distribution to model the number of silly mistakes Xiao would make in a piece of homework that is five pages long.

1-2-2-calculating-poisson-probabilities-we-solution-part-1

(b)

Find the probability that in any random page of Xiao’s homework book there are

(i)

exactly three silly mistakes

(ii)

at most three silly mistakes

(iii)

more than three silly mistakes.

1-2-2-calculating-poisson-probabilities-we-solution-part-2

Examiner Tip

Look carefully at the given time or space interval to check if you need to change the mean before carrying out calculations.
- Be prepared for this to change between question parts!

Calculating Poisson Probabilities (Edexcel International A Level Maths: Statistics 2)

Revision Note