International A Level (IAL)MathsEdexcelStatistics 2Revision NotesStatistical DistributionsPoisson DistributionThe Poisson Distribution

The Poisson Distribution (Edexcel International A Level (IAL) Maths): Revision Note

Exam code: YMA01

Amber

Author

Amber

Last updated

Did this video help you?

Properties of Poisson Distribution

What is a Poisson distribution?

  • A Poisson distribution is a discrete probability distribution

  • The discrete random variable X follows a Poisson distribution if it counts the number of events that occur at random in a given time or space

  • For a Poisson distribution to be valid it must satisfy the following properties:

    • Events occur singly and at random in a given interval of time or space

    • The mean number of occurrences in the given interval(λ)  is known and finite

      • λ has to be positive but does not have to be an integer

    • Each occurrence is independent of the other occurrences

  • If X follows a Poisson distribution then it is denoted X tilde Po left parenthesis lambda right parenthesis

    • λ is the mean number of occurrences of the event

  • The formula for the probability of r occurrences in a given interval is:

    • P left parenthesis X equals r right parenthesis equals e to the power of negative lambda end exponent cross times fraction numerator lambda to the power of r over denominator r factorial end fraction for r=0, 1, 2, ...,n

    • e is the constant 2.718…

    • r factorial equals r open parentheses r minus 1 close parentheses open parentheses r minus 2 close parentheses....2 cross times 1

What are the important properties of a Poisson distribution?

  • The mean and variance of a Poisson distribution are roughly equal

  • The distribution can be represented visually using a vertical line graph

    • If λ is close to 0 then the graph has a tail to the right (positive skew)

    • If λ is at least 5 then the graph is roughly symmetrical

  • The Poisson distribution becomes more symmetrical as the value of the mean (λ) increases

2-1-1-poisson-distribution-diagram-1

Worked Example

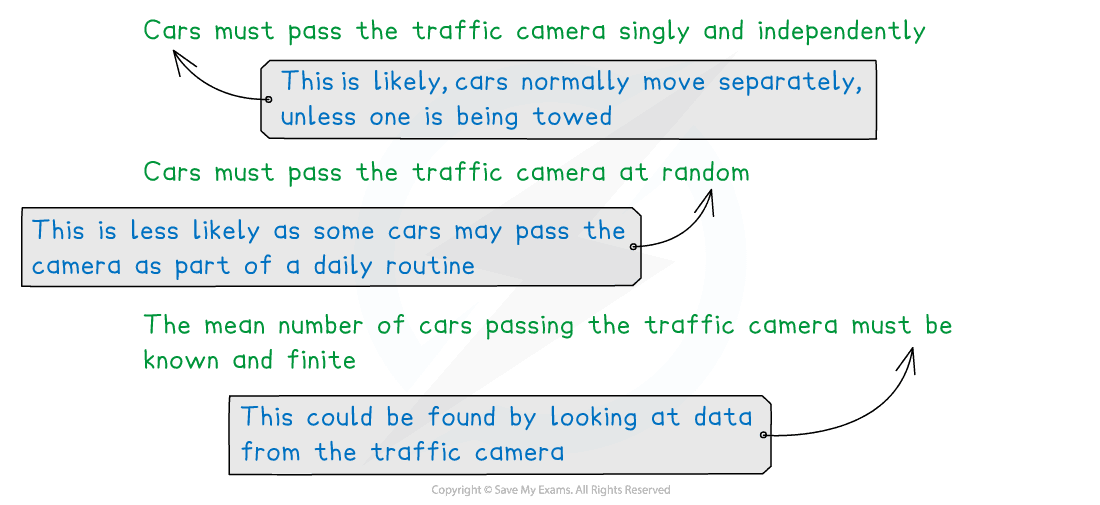
 X is the random variable ‘The number of cars that pass a traffic camera per day’. State the conditions that would need to be met for X to follow a Poisson distribution.

2-1-1-the-poisson-distribution-we-solution-1

Did this video help you?

Modelling with Poisson Distribution

How do I set up a Poisson model?

  • Find the mean and variance and check that they are roughly equal

    • You may have to change the mean depending on the given time/space interval

  • Make sure you clearly state what your random variable is

    • For example, let X be the number of typing errors per page in an academic article

  • Identify what probability you are looking for

What can be modelled using a Poisson distribution?

  • Anything that occurs singly and randomly in a given interval of time or space and satisfies the conditions

  • For example, let X  be the random variable 'the number of emails that arrive into your inbox per day'

    • There is a given interval of a day, this is an example of an interval of time

    • We can assume the emails arrive into your inbox at random

    • We can assume each email is independent of the other emails

      • This is something that you would have to consider before using the Poisson distribution as a model

    • If you know the mean number of emails per day a Poisson distribution can be used

  • Sometimes the given interval will be for space

    • For example, the number of daisies that exist on a square metre of grass

    • look carefully at the units given as you may have to change them when calculating probabilities

Worked Example

State, with reasons, whether the following can be modelled using a Poisson distribution and if so write the distribution.

(i) Faults occur in a length of cloth at a mean rate of 2 per metre.

 

(ii) On average 4% of a certain population has green eyes.

 

(iii) An emergency service company receives, on average, 15 calls per hour.

2-1-1-modelling-with-a-poisson-we-solution-2

Examiner Tips and Tricks

  • If you are asked to criticise a Poisson model always consider whether the trials are independent, this is usually the one that stops a variable from following a Poisson distribution!

You've read 1 of your 5 free revision notes this week

Unlock more, it's free!

Join the 100,000+ Students that ❤️ Save My Exams

the (exam) results speak for themselves:

  • I would just like to say a massive thank you for putting together such a brilliant, easy to use website.I really think using this site helped me secure my top gradesin science and maths. You really did save my exams! Thank you.

    Beth
    IGCSE Student

  • This website is soooo useful and I can’t ever thank you enough for organising questions by topic like this. Furthermore, the name of the website could not have been more appropriate as it literally did SAVE MY EXAMS!

    Fathima
    A Level Student
Test yourself

Did this page help you?

Previous:Calculating Binomial ProbabilitiesNext:Calculating Poisson Probabilities
Amber

Author: Amber

Expertise: Maths Content Creator

Amber gained a first class degree in Mathematics & Meteorology from the University of Reading before training to become a teacher. She is passionate about teaching, having spent 8 years teaching GCSE and A Level Mathematics both in the UK and internationally. Amber loves creating bright and informative resources to help students reach their potential.

Download notes on The Poisson Distribution