Point of Tipping (Edexcel International A Level Maths: Mechanics 2)

What is meant by point of tipping/toppling?

• These types of problems involve a lamina being placed along one of its edges on a rough inclined plane
• The (main) modelling assumption here is that the frictional force between the plane and the lamina is sufficient such that the lamina will not slide (or slip) down the plane (regardless of the angle of incline)

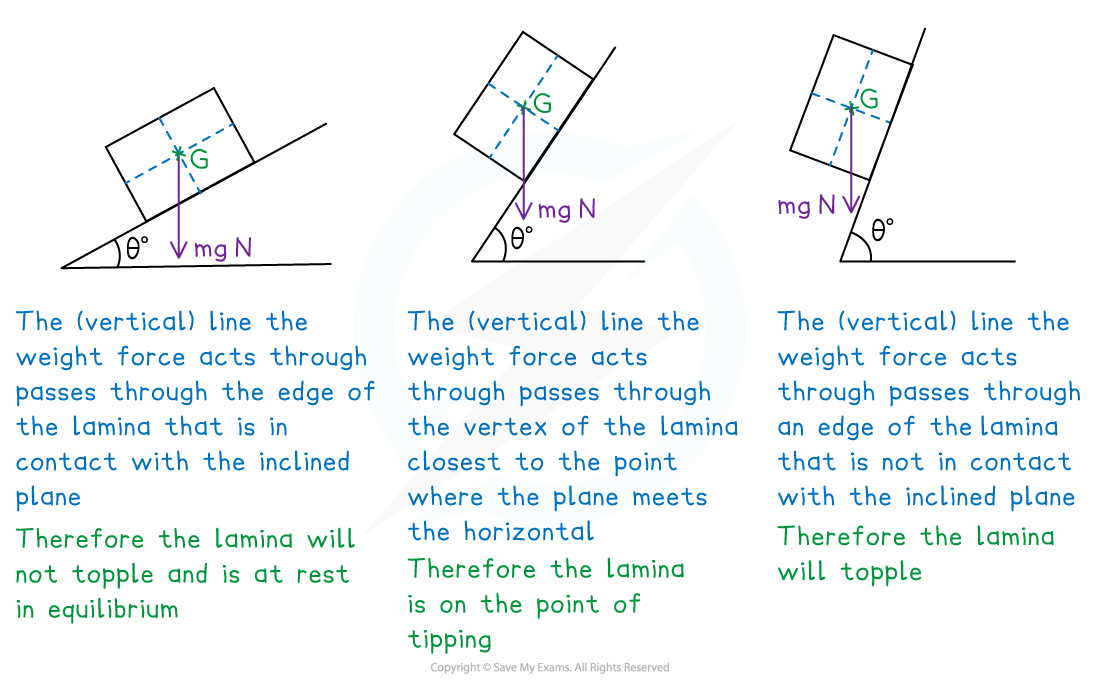
How do I solve tipping/toppling problems?

                 STEP 1   Sketch or add anything useful to a given diagram

  Define axes if necessary and add these to your diagram

• • • • • make the -axis parallel to the plane
        • make the -axis perpendicular to the plane
        • have the origin (O) at the vertex of the lamina that is in contact with the plane and closest to the point where the plane meets the horizontal (“bottom left”)
        • Add to the diagram as you progress through the question

                 STEP 2   Find the position of the centre of mass (G) of the lamina; use your diagram, 

                                 axes and symmetry (where possible) to help 

                                 The lamina may be a standard shape or a composite lamina

   Add the position of the centre of mass to your diagram; also add a downward

   vertical line (that weight  acts through) but you cannot use your diagram to

   accurately determine whether the lamina will topple

                STEP 3   Use trigonometry (A new diagram of a right-angled triangle with OG as the

                                hypotenuse can help) to determine the angle at which the lamina would be

                                on the point of tipping

                 STEP 4   Interpret the result in the context of the problem and answer the question accordingly