Enthalpy of Solution - Calculations (Edexcel International A Level Chemistry)

• Questions in this topic typically ask you to calculate the hydration enthalpy of one of the ions, given the lattice enthalpy, enthalpy of solution and hydration enthalpy of the other ion.
• This can be done by constructing an appropriate energy cycle and using Hess's Law to find the unknown energy value
• The energy cycle above shows that there are two routes to go from the gaseous ions to the ions in an aqueous solution:
  • • Route 1: going from gaseous ions → ionic solid → ions in aqueous solution (this is the indirect route)
    • Route 2: going from gaseous ions  →  ions in aqueous solution (this is the direct route)

• According to Hess’s law, the enthalpy change for both routes is the same, such that:

Δ_hydH^ꝋ = Δ_lattH^ꝋ + Δ_solH^ꝋ

• Each ion will have its own enthalpy change of hydration, ΔH_hyd^ꝋ, which will need to be taken into account during calculations
  • The total Δ_hydH^ꝋ is found by adding the Δ_hydH^ꝋ values of both anions and cations together

Answer

Step 1: Draw the energy cycle and make Δ_hydH[Cl^-] the subject of the formula:

Step 2: Substitute the values to find Δ_hydH[Cl^-]

Δ_hydH[Cl^-]   = (-711) + (+26) - (-322) = -363 kJ mol^-1

Alternative Diagram

• You can also draw a Born-Haber cycle as an alternative approach to the same problem

Energy level diagram:

Answer

Step 1: Draw an energy cycle:

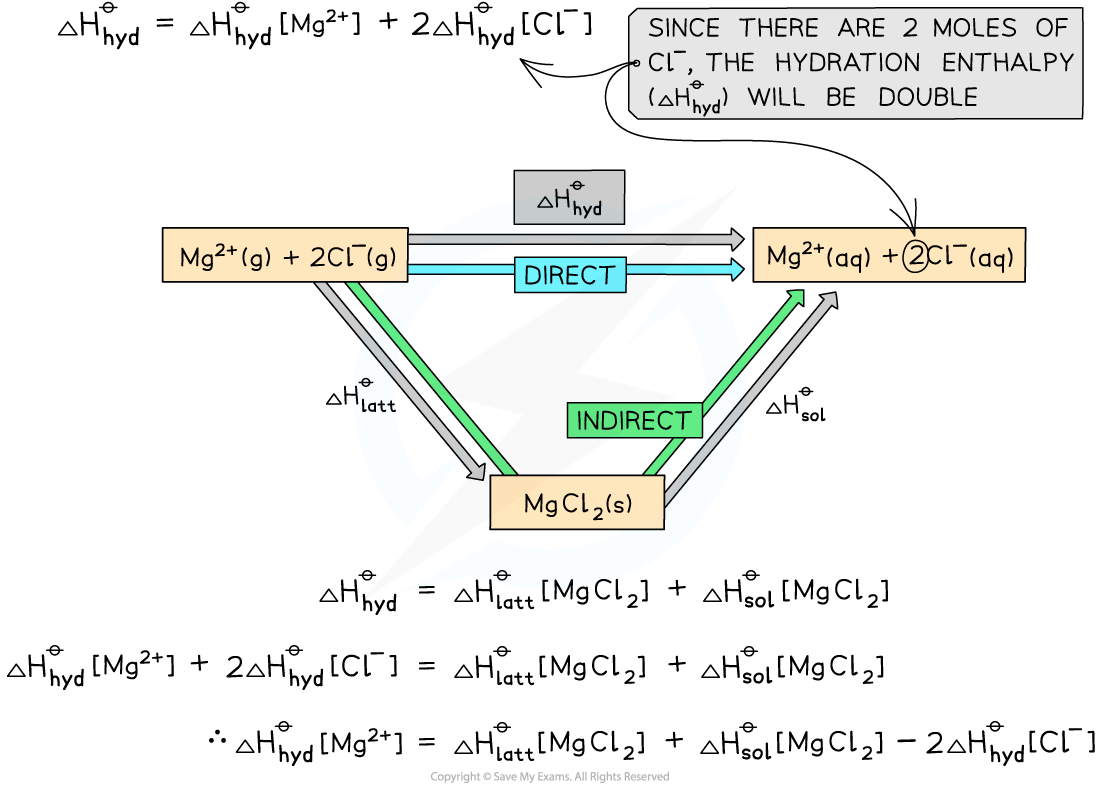
Step 2: Substitute the values to find ΔH_hyd^ꝋ [Mg²⁺]

ΔH_hyd^ꝋ[Mg²⁺]  = (-2592) + (-55) - (2 x -363) = -1921 kJ mol^-1

Alternative route to find Δ_hydH^ꝋ[Mg²⁺] 

• Here is the same solution using a Born-Haber cycle

• When an ionic compound dissolves in water, there are two changes that need to take place
  • The lattice structure needs to be broken down and the ions must become hydrated
• The breaking down of the lattice structure is endothermic (equivalent to the reverse lattice energy) it also results in an increased number of moles of particles present, therefore entropy will increase
• The hydration of the ions is an exothermic process but results in water molecules becoming more ordered as they arrange themselves around the cations and anions 
• This increasing in order decreases the entropy of the water 
• Therefore to explain why some ionic compounds are soluble and some are insoluble we must consider both entropy and enthalpy changes involved 

• As we have seen previously                                                           

ΔS_total = Δ S_system+ Δ S_surroundings

      And since

Δ S_surroundings

 The expression can become

Δ S_total= Δ S_system

• Therefore the solubility of an ionic solid depends upon three factors
  • The entropy of the system, ΔS_system
  • The enthalpy change of solution, Δ_solH
  • The temperature, in K, of the water, T

If we consider ammonium nitrate, NH₄NO₃ (s), at 298 K

NH₄NO₃ (s) → NH₄⁺ (aq) + NO₃^- (aq)

• The enthalpy change of solution, Δ_solH = +25.8 kJ mol^-1
• The temperature, in K, of the water, T = 298 K

ΔS_system = S[NH₄⁺ (aq)] + S[NO₃^- (aq)] - S[NH₄NO₃ (s)]

= +113.4 + 146.4 - 151.1

= +108.7 J K^-1 mol^-1 

 = 

= -86.6 J K^-1 mol^-1

• Therefore the total entropy is: 

ΔS_total = Δ S_system+ Δ S_surroundings

= +108.7 + (-86.6)

= +22.1 J K^-1 mol^-1

• If the entropy change is positive, as it is for dissolving ammonium nitrate in water at 298 K, then this is thermodynamically spontaneous 
• The activation energy (E_a) for this reaction is very low, we can conclude that at 298 K, ammonium nitrate is soluble in water