Entropy - Introduction (Edexcel International A Level Chemistry)

• You may have wondered why it is that endothermic reactions occur at all, after all, what can be the driving force behind endothermic reactions if the products end up in a less stable, higher energy state?
• Although the majority of chemical reactions we experience everyday are exothermic,  ΔH^ꝋ alone is not enough to explain why endothermic reactions occur

The driving force behind chemical reactions cannot be explained by enthalpy changes alone as it makes not sense for chemical to end up in a less stable higher energy state in endothermic reactions

• The answer is entropy

Chaos in the universe

• The entropy (S) of a given system is the number of possible arrangements of the particles and their energy in a given system
  • In other words, it is a measure of how disordered or chaotic a system is

• When a system becomes more disordered, its entropy will increase
• An increase in entropy means that the system becomes energetically more stable

• An example of a system that becomes more disordered is when a solid is melted
• For example, melting ice to form liquid water:

H₂O (s) → H₂O (l)

• The water molecules in ice are in fixed positions and can only vibrate about those positions
• In the liquid state, the particles are still quite close together but are arranged more randomly, in that they can move around each other
• Water molecules in the liquid state are therefore more disordered
• Thus, for a given substance, the entropy increases when its solid form melts into a liquid
• In both examples, the system with the higher entropy will be energetically favourable (as the energy of the system is more spread out when it is in a disordered state)

Melting a solid will cause the particles to become more disordered resulting in a higher entropy state

Distribution of Molecules

• Consider two gas jars, separated by a cover slip and one of the gas jars containing five molecules of orange bromine gas
• When the cover slip between two gas jars is removed, molecules of gas will spread out evenly throughout both of the gas jars 

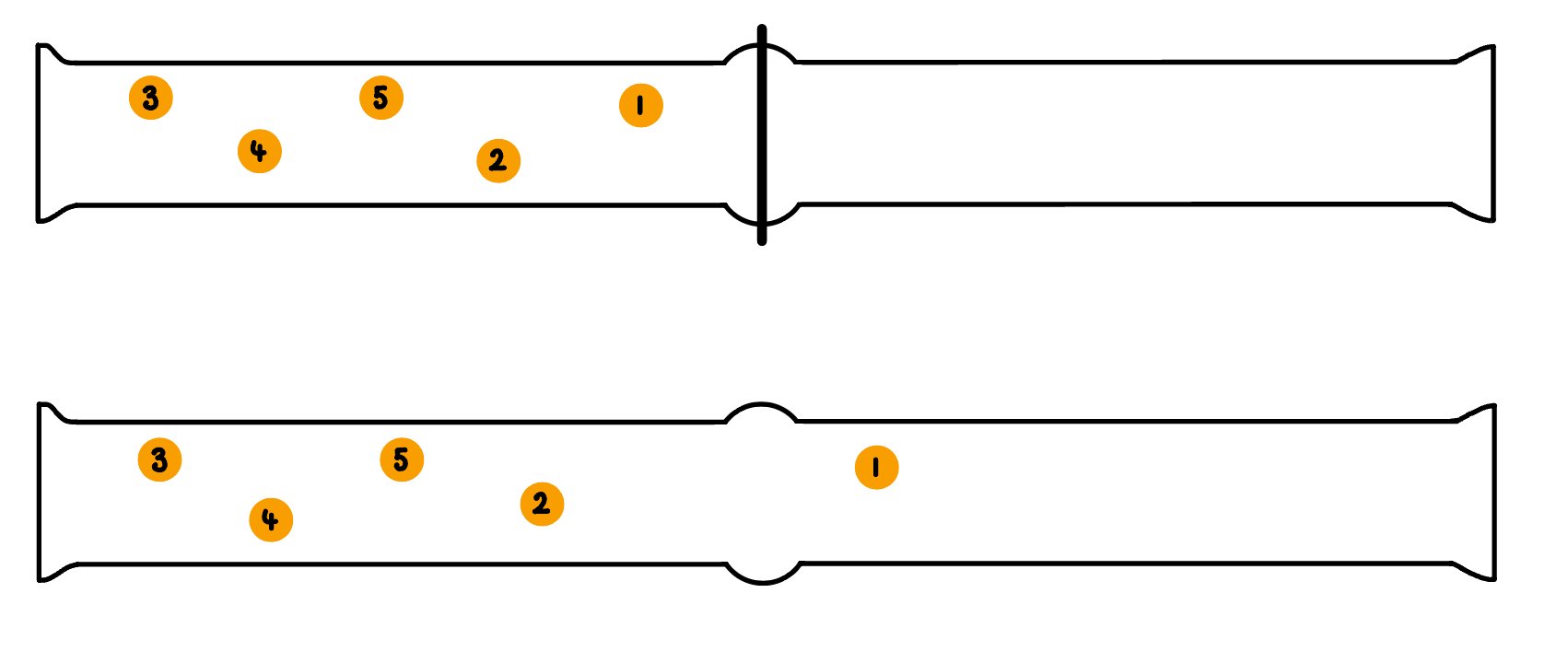The first two gas jars are separated by a glass cover slip, the second two gas jars are not separated allowing molecules to spread evenly between the two 

• Each molecule can either be in the left or the right hand gas jar, so therefore has two possible arrangements 
• So the number of possible arrangements is 2
• If there are 5 molecules in one jar to begin with, the total number of ways, W, the five molecules can be arranged is
  • W = W₁ x W₂ x W₃ x W₄ x W₅
  • W = 2 x 2 x 2 x 2 x 2
  • W = 2⁵
  • W = 32
• If we increased the number of molecules to 100, W becomes 2¹⁰⁰

• Therefore as the number of particles increases the number of possible arrangements increases rapidly 
• Entropy (S) is linked to W by the equation 

S = k InW

• • k = Boltzmann distribution constant 1.38 x 10^-23 J K^-1 mol^-1
  • The units for entropy (S) are J K^-1 mol^-1

Distribution of energy

• The spreading out of molecules in diffusion is an increase in entropy as the molecules are becoming more randomly dispersed
• Spreading out heat energy also represents an increase in entropy
• Energy exists in ‘packets’ called quanta
  • Only whole numbers of quanta are possible
• The more quanta, the more ways of arrange the particles
• The more particles the more ways of sharing quanta 

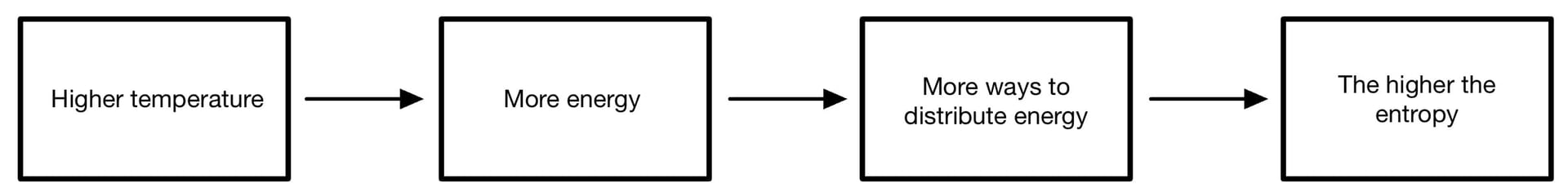
Flow chart to show the effect of increasing temperature on entropy