Area under a Velocity-Time Graph (Edexcel IGCSE Science (Double Award)) : Revision Note
Did this video help you?
Area under a velocity-time graph
How to find the area under a velocity-time graph
The area under a velocity-time graph represents the displacement (or distance travelled) by an object
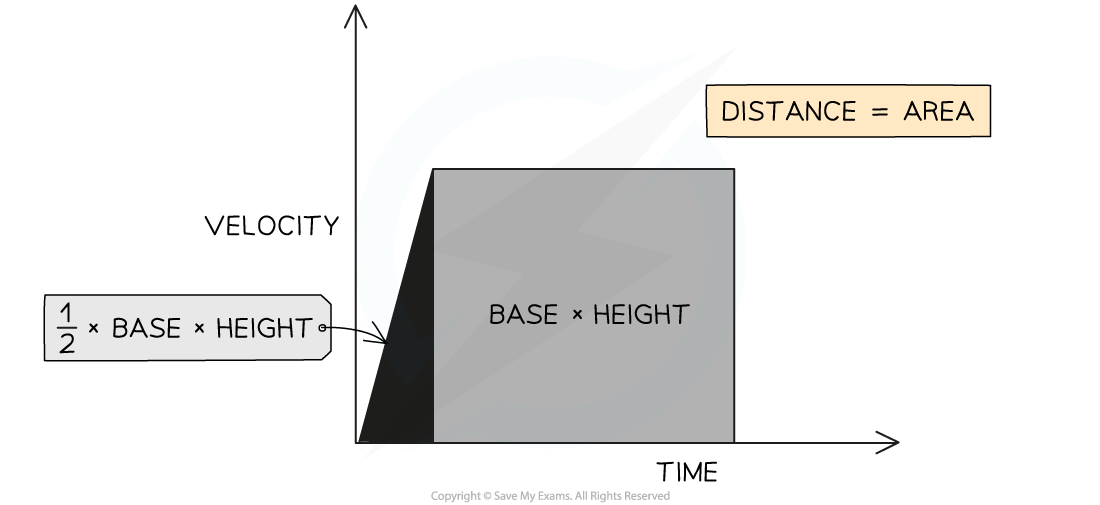
The displacement, or distance travelled, is represented by the area beneath the graph
If the area beneath the velocity-time graph forms a triangle (i.e. the object is accelerating or decelerating), then the area can be determined by using the following formula:
Area = ½ × Base × Height
If the area beneath the velocity-time graph forms a rectangle (i.e. the object is moving at a constant velocity), then the area can be determined by using the following formula:
Area = Base × Height
How to find distance from a velocity-time graph
Enclosed areas under velocity-time graphs represent total displacement (or total distance travelled) in a time interval
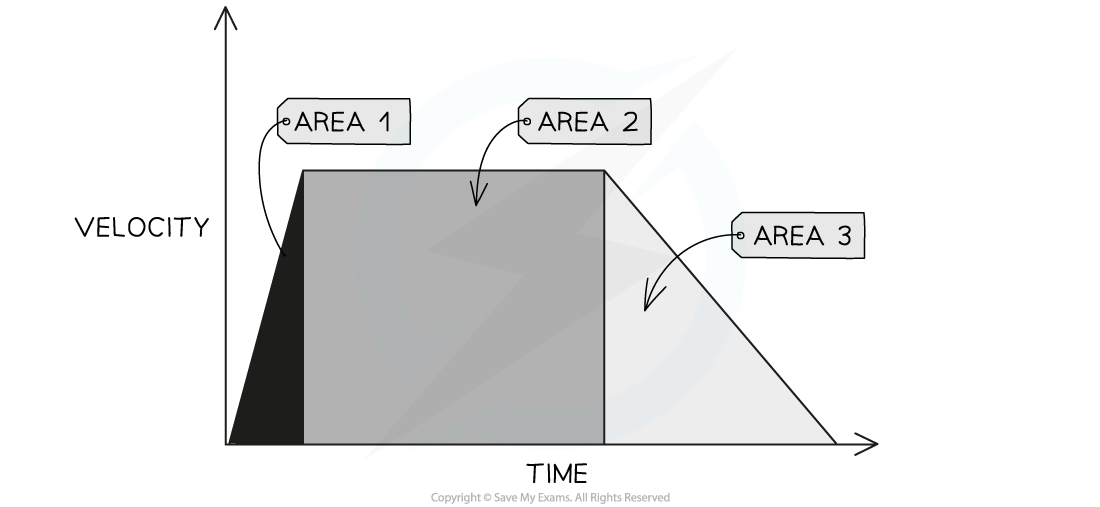
Three enclosed areas (two triangles and one rectangle) under this velocity-time graph represent the total distance travelled in the total time
If an object moves with constant acceleration, its velocity-time graph will consist of straight lines
In this case, calculate the distance travelled by working out the area of enclosed rectangles and triangles
The area of each enclosed section represents the distance travelled in that particular interval of time
The total distance travelled is the sum of all the individual enclosed areas
Worked Example
The velocity-time graph below shows a car journey that lasts for 160 seconds.

Calculate the total distance travelled by the car.
Answer:
Step 1: Recall that the area under a velocity-time graph represents the distance travelled
In order to calculate the total distance travelled, the total area underneath the line must be determined
Step 2: Identify each enclosed area
In this example, there are five enclosed areas under the line
These can be labelled as areas 1, 2, 3, 4 and 5, as shown in the image below:
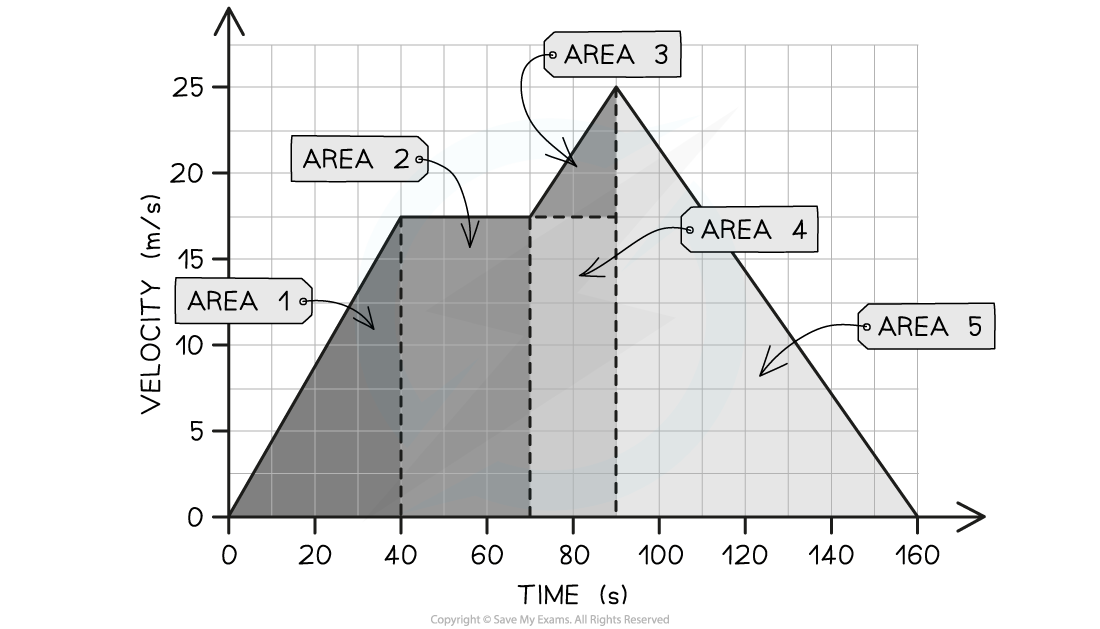
Step 3: Calculate the area of each enclosed shape under the line
Area 1 = area of a triangle
Area 2 = area of a rectangle
Area 3 = area of a triangle
Area 4 = area of a rectangle
Area 5 = area of a triangle
Step 4: Calculate the total distance travelled by finding the total area under the line
Add up each of the five areas enclosed:
Examiner Tips and Tricks
Some areas will need to be split into a triangle and a rectangle to determine the area for a specific time interval, like areas 3 & 4 in the worked example above.
If you are asked to find the distance travelled for a specific time interval, then you just need to find the area of the section above that time interval.
For example, the distance travelled between 70 s and 90 s is the sum of Area 3 + Area 4

You've read 0 of your 5 free revision notes this week
Sign up now. It’s free!
Did this page help you?
