The Principle of Moments (Edexcel IGCSE Physics (Modular))

Revision Note

Written by: Katie M

The principle of moments

The principle of moments states that:

If an object is balanced, the total clockwise moment about a pivot equals the total anticlockwise moment about that pivot

For a balanced object, the moments on both sides of the pivot are equal

clockwise moment = anticlockwise moment

Clockwise and anticlockwise moments

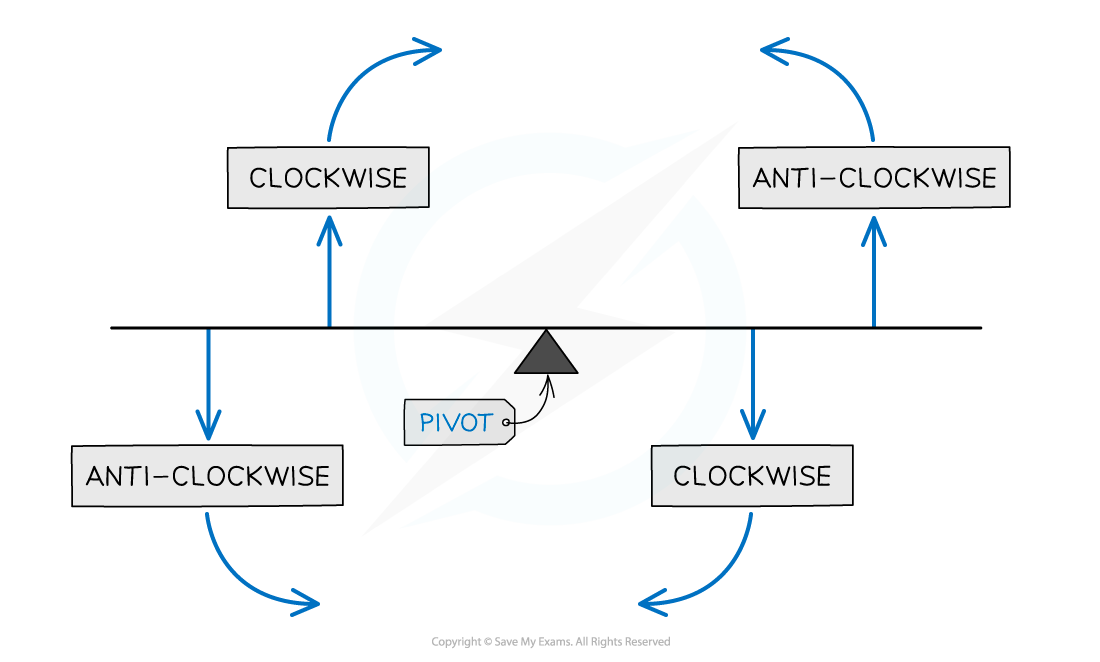
Imagine holding the beam about the pivot and applying just one of the forces. If the beam moves clockwise then the force applied is clockwise.

In the example below, the forces and distances of the objects on the beam are different, but they are arranged in a way that balances the whole system

Remember that the moment = force × distance from a pivot
The forces should be perpendicular to the distance from the pivot
- For example, on a horizontal beam, the forces which will cause a moment are those directed upwards or downwards

Using the principle of moments

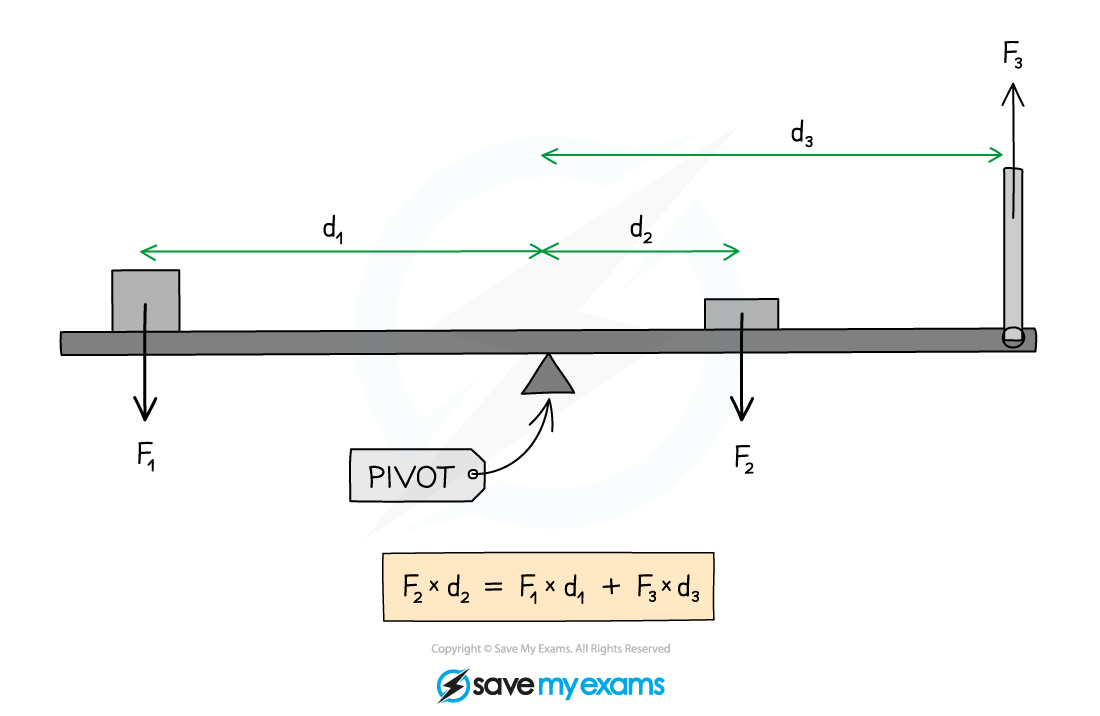
The clockwise and anticlockwise moments acting on a beam are balanced

In the above diagram:
- Force $F_{1}$ causes an anticlockwise moment of $F_{1} \times d_{1}$ about the pivot
- Force $F_{2}$ causes a clockwise moment of $F_{2} \times d_{2}$ about the pivot
- Force $F_{3}$ causes an anticlockwise moment of $F_{3} \times d_{3}$ about the pivot

Collecting the clockwise and anticlockwise moments:
- Sum of the clockwise moments = $F_{2} \times d_{2}$
- Sum of the anticlockwise moments = $(F_{1} \times d_{1}) + (F_{3} \times d_{3})$

Using the principle of moments, the beam is balanced when:

Sum of the clockwise moments = Sum of the anticlockwise moments

$F_{2} \times d_{2} = (F_{1} \times d_{1}) + (F_{3} \times d_{3})$

Worked Example

A parent and child are at opposite ends of a playground see-saw. The parent weighs 690 N and the child weighs 140 N. The adult sits 0.3 m from the pivot.

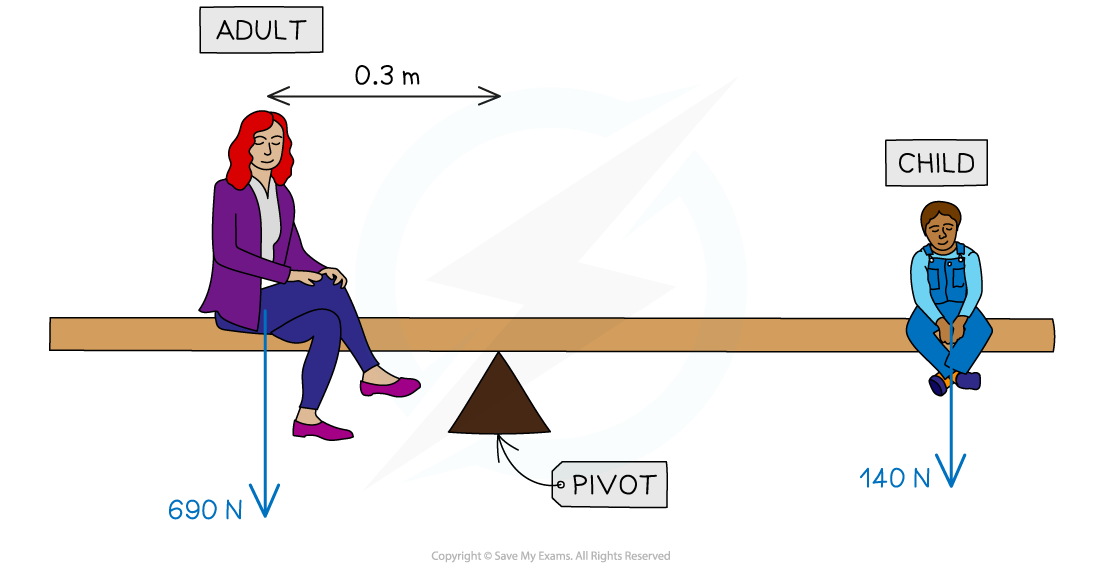
Calculate the distance the child must sit from the pivot for the see-saw to be balanced.

Answer:

Step 1: List the known quantities

Clockwise force (child), F_child = 140 N
Anticlockwise force (adult), F_adult = 690 N
Distance of adult from the pivot, d_adult = 0.3 m

Step 2: Write down the relevant equation

Moments are calculated using:

Moment = force × distance from pivot

For the see-saw to balance, the principle of moments states that

Total clockwise moments = Total anticlockwise moments

Step 3: Calculate the total clockwise moments

The clockwise moment is from the child

Moment of child (clockwise) = F_child × d_child

Moment of child (clockwise) = 140 × d_child

Step 4: Calculate the total anticlockwise moments

The anticlockwise moment is from the adult

Moment of adult (anticlockwise) = F_adult × d_adult

Moment of adult (anticlockwise) = 690 × 0.3 = 207 N m

Step 5: Substitute into the principle of moments equation

Moment of child (clockwise) = Moment of adult (anticlockwise)

140 × d_child = 207

Step 6: Rearrange for the distance of the child from the pivot

d_child = $\frac{207}{140}$ = 1.5 m

The child must sit 1.5 m from the pivot to balance the see-saw

Examiner Tips and Tricks

Make sure that all the distances are in the same units and that you’re considering the correct forces as clockwise or anticlockwise.

In your IGCSE exam, you will only be expected to apply the principle of moments to a situation where an object rotates in one plane (up and down or side to side)

Supporting a beam

A light beam is one that can be treated as though it has no mass
The supports, therefore, must exert upward forces that balance the downward acting weight of any object placed on the beam

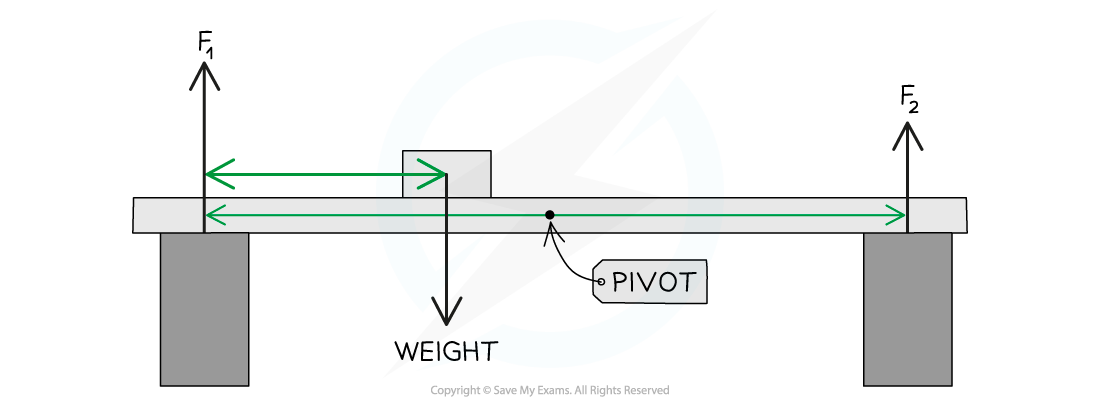
F₁ and F₂ upwards balance the weight of the beam downwards

As the mass in the above diagram is moved from the left-hand side to the right-hand side of the beam, force F₁ will decrease and force F₂ will increase

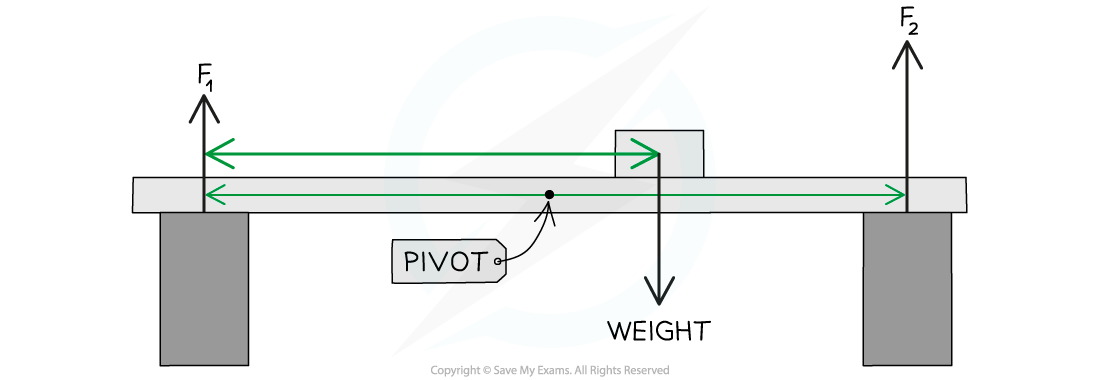
Supporting a Beam (2), downloadable IGCSE & GCSE Physics revision notes

F₁ decreases F₂ increases keep the beam balanced

Consider what would happen to the beam if the right-hand support was removed:
- Force F₂ would be 0
- The weight of the object would produce a moment about the left-hand support, causing the beam to pivot in a clockwise direction

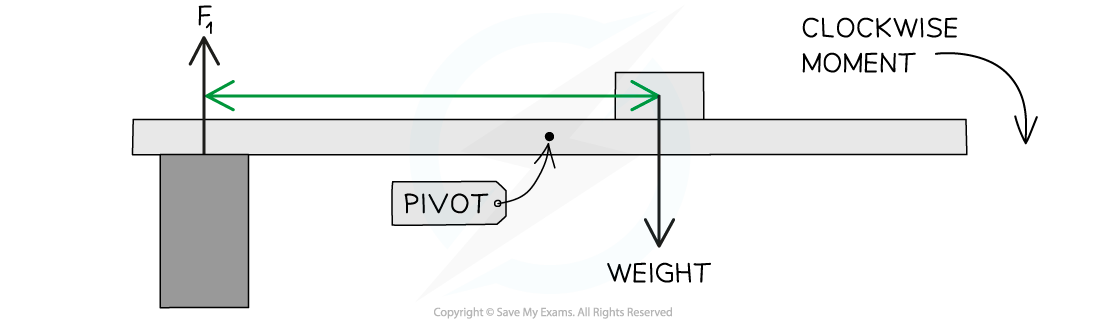
Supporting a Beam (3), downloadable IGCSE & GCSE Physics revision notes

When F₂ is removed the beam will rotate by the clockwise moment

Therefore, the force F₂ must therefore supply an anticlockwise moment about the left-hand support, which balances the moment supplied by the object

Last updated: 26 August 2024

You've read 0 of your 5 free revision notes this week

Sign up now. It’s free!

Join the 100,000+ Students that ❤️ Save My Exams

the (exam) results speak for themselves:

Test yourself

Did this page help you?

Previous:MomentsNext:Centre of Gravity