Boyle's Law (Edexcel IGCSE Physics)

Boyle's law

• For a fixed mass of a gas held at a constant temperature, the Boyle's law formula is:

pV = constant

• Where:

  • p = pressure in pascals (Pa)

  • V = volume in metres cubed (m³)

• This means that the pressure and volume are inversely proportional to each other

  • When the volume decreases (compression), the pressure increases

  • When the volume increases (expansion), the pressure decreases

• This is because when the volume decreases, the same number of particles collide with the walls of a container but more frequently as there is less space

  • However, the particles still collide with the same amount of force meaning greater force per unit area (pressure)

• The key assumption is that the temperature and the mass (and number) of the particles remains the same

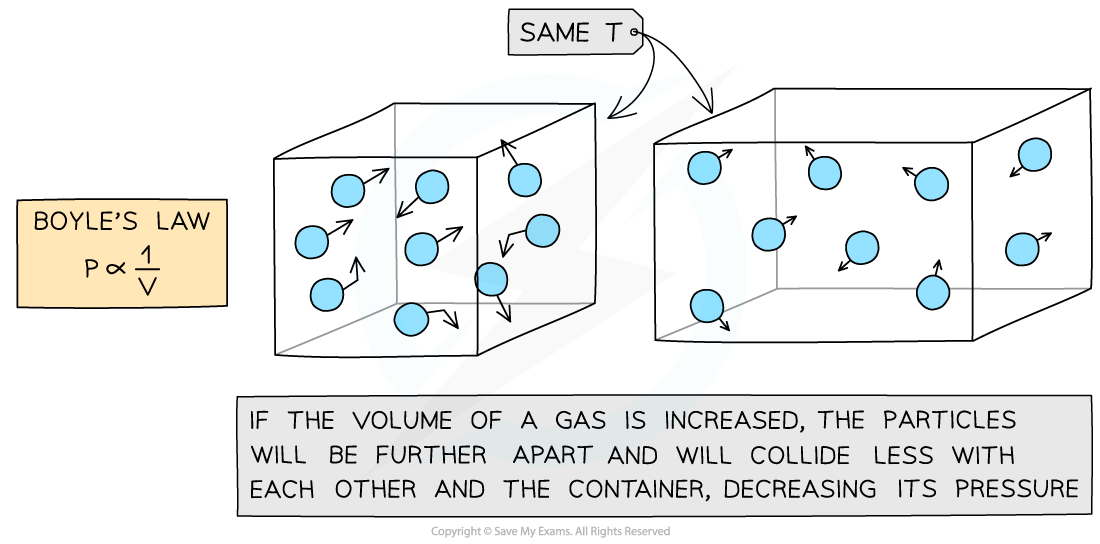
Increasing the volume of a gas decreases its pressure

• This equation can also be rewritten for comparing the pressure and volume before and after a change in a gas:

p₁V₁ = p₂V₂

• Where:

  • p₁ = initial pressure in pascals (Pa)

  • V₁ = initial volume in metres cubed (m³)

  • p₂ = final pressure in pascals (Pa)

  • V₂ = final volume in metres cubed (m³)

• This equation is sometimes referred to as Boyle's Law

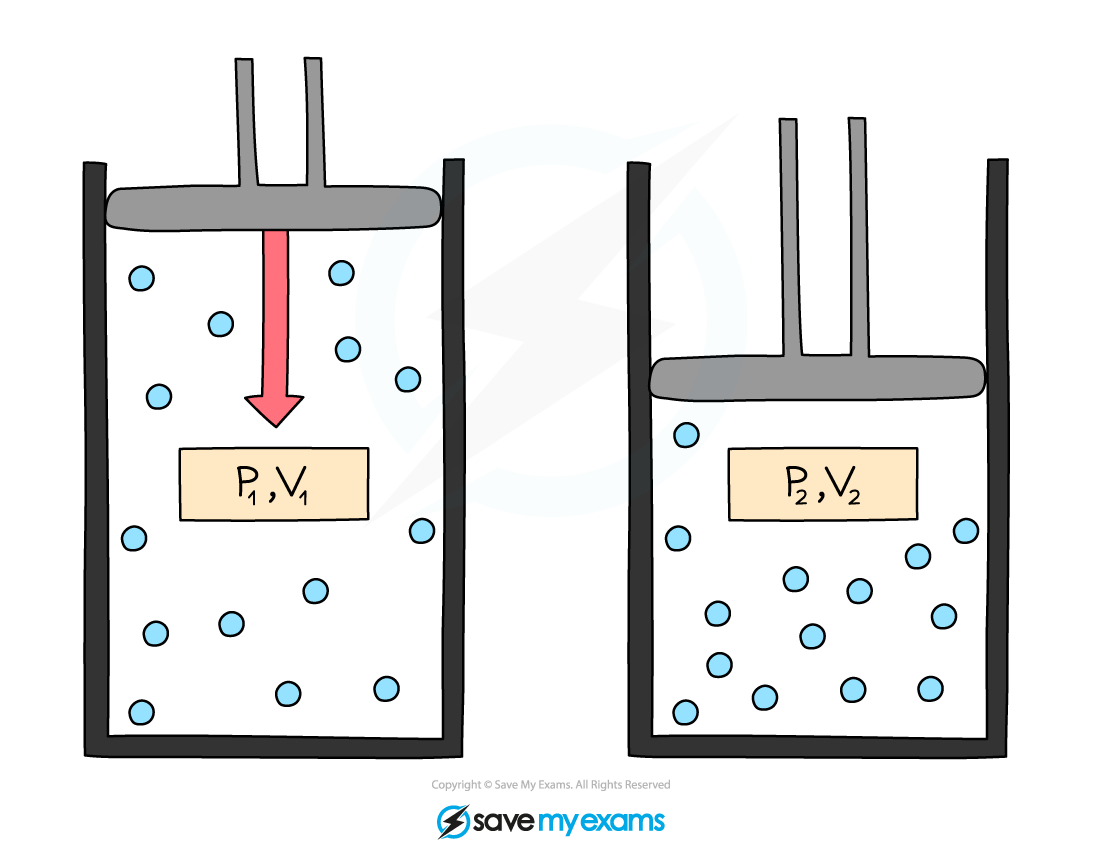
Initial pressure and volume, p₁ and V₁, and final pressure and volume, p₂ and V₂. When volume decreases, pressure increases