Conservation of Energy (Cambridge (CIE) IGCSE Physics): Revision Note
Exam code: 0625 & 0972
Conservation of energy
The principle of conservation of energy states that:
Energy cannot be created or destroyed; it can only be transferred from one store to another
The principle of conservation of energy means that for a closed system, the total amount of energy is constant
The total amount of energy transferred into the system must be equal to the total amount of energy transferred away from the system
Therefore, energy cannot be ‘lost’, but it can be transferred to the surroundings
Energy can be dissipated (spread out) to the surroundings by heating and radiation
Dissipated energy transfers are often not useful, in which case they can be described as wasted energy (opens in a new tab)
Examples of the principle of conservation of energy
Example 1: a bat hitting a ball
The moving bat has energy in its kinetic store
Some of that energy is transferred usefully to the kinetic store of the ball
Some of that energy is transferred from the kinetic store of the bat to the thermal store of the ball mechanically due to the impact of the bat on the ball
This energy transfer is not useful; the energy is wasted
Some of that energy is dissipated by heating to the thermal store of the bat, the ball, and the surroundings
This energy transfer is not useful; the energy is wasted
The total amount of energy transferred into the system is equal to the total amount of energy transferred away from the system
Conservation of energy: a bat hitting a ball
Energy flow diagram showing energy transfers when a bat hits a ball
The principle of conservation of energy applied to a bat hitting a ball
Example 2: boiling water in a kettle
When an electric kettle boils water, energy is transferred electrically from the mains supply to the thermal store of the heating element inside the kettle
As the heating element gets hotter, energy is transferred by heating to the thermal store of the water
Some of the energy is transferred to the thermal store of the plastic kettle
This energy transfer is not useful; the energy is wasted
And some energy is dissipated to the thermal store of the surroundings due to the air around the kettle being heated
This energy transfer is not useful; the energy is wasted
The total amount of energy transferred into the system is equal to the total amount of energy transferred away from the system
Conservation of energy: a kettle boiling water
The principle of conservation of energy applied to a kettle boiling water
Examiner Tips and Tricks
When describing wasted energy, always say which store it ends up in.
Energy flow diagrams
Energy stores and transfers can be represented using a flow diagram
This shows both the stores and the transfers taking place within a system
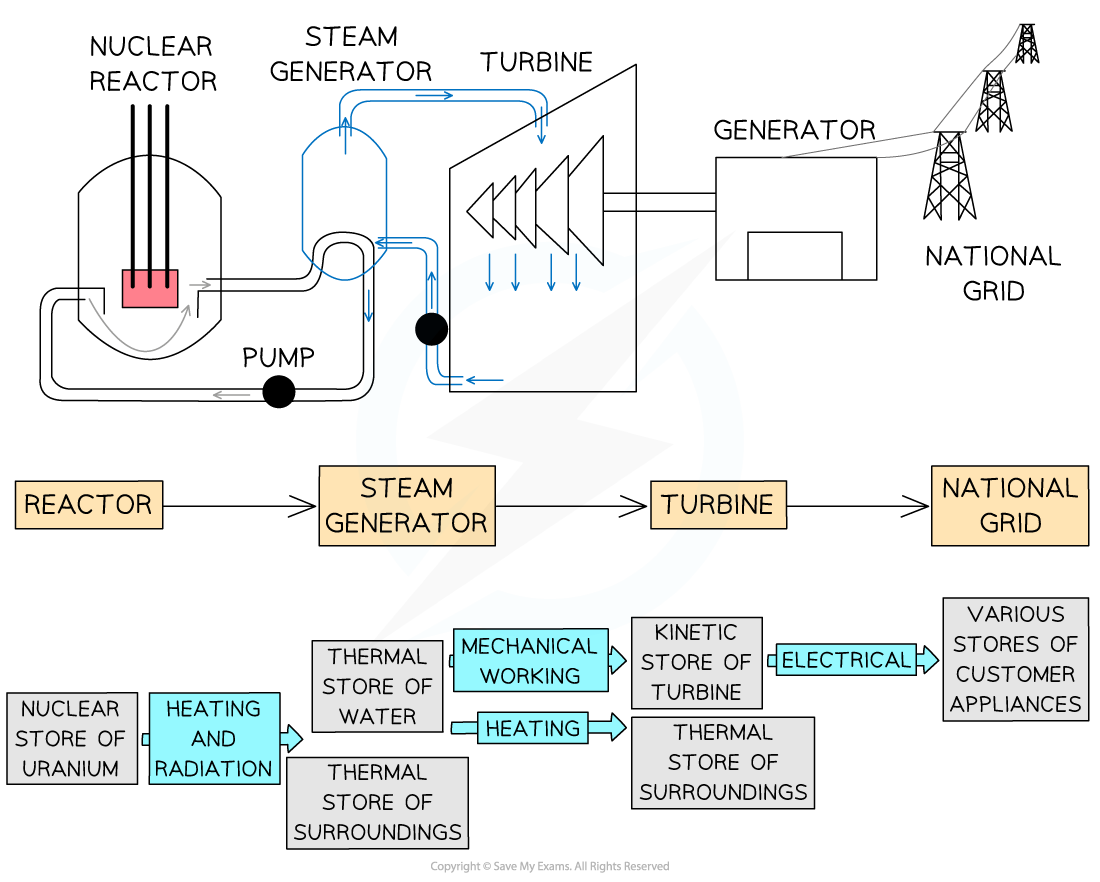
Energy flow diagram for a nuclear power plant. Stores are shown in labelled boxes and transfer pathways as arrows between them
In an energy flow diagram, energy is always conserved
total energy in = total energy out
Examiner Tips and Tricks
This is a useful way to do calculations, but it is not a statement of the principle of conservation of energy — if a question asks you to state the principle, you must say that energy cannot be created or destroyed; it can only be transferred from one store to another.
Note that you may be asked to fill in missing labels in energy flow diagrams.
Worked Example
The diagram shows a rollercoaster going down a track.
The rollercoaster takes the path A → B → C → D.
Which statement is true about the energy changes that occur for the rollercoaster down this track?
A. EK → ΔEP → ΔEP → EK
B. EK → ΔEP → EK → ΔEP
C. ΔEP → EK → EK → ΔEP
D. ΔEP → EK → ΔEP → EK
[1]
Answer:
At point A:
The rollercoaster is raised above the ground, therefore it has energy in its gravitational potential store
At point B:
As it travels down the track towards B, energy is transferred mechanically to its kinetic store
At point C:
Energy is transferred mechanically from the kinetic store to the gravitational potential store
As the kinetic energy store empties, the gravitational potential energy store fills
At point D:
Energy is transferred mechanically from the gravitational potential store to the kinetic store
The flat terrain means there is no change in the amount of energy in its gravitational potential store, the rollercoaster only has energy in its kinetic store
The kinetic energy store is full
Therefore, the energy changes that occur for the rollercoaster down this track is given by D: ΔEP → EK → ΔEP → EK [1 mark]
In reality, some energy will also be transferred to the thermal energy store of the tracks due to friction, and away from the system by sound
This energy is described as dissipated to the surroundings
The total amount of energy in the system will be constant
Total energy in = total energy out
Examiner Tips and Tricks
It is helpful to think of energy stores as beakers and the total energy in the system as water. The water can be poured from one beaker into another, back and forth, as energy is transferred between stores.
You may not always be given the energy transfers happening in the system in your IGCSE exam questions.
By familiarising yourself with the energy stores and transfer pathways, you should be able to relate these to the situation presented in the question. For example, a ball rolling down a hill transfers energy from the ball's gravitational potential energy store to its kinetic energy store mechanically, while a spring transfers energy from its elastic potential energy store to its kinetic energy store mechanically.
Sankey diagrams
Extended tier only
Sankey diagrams can be used to represent energy transfers
Sankey diagrams are characterised by arrows that split to show the proportions of the energy transfers taking place
The different parts of the arrow in a Sankey diagram represent the different energy transfers:
The left-hand side of the arrow (the flat end) represents the energy transferred into the system
The straight arrow pointing to the right represents the energy that ends up in the desired store; this is the useful energy output
The arrows that bend away represent the wasted energy
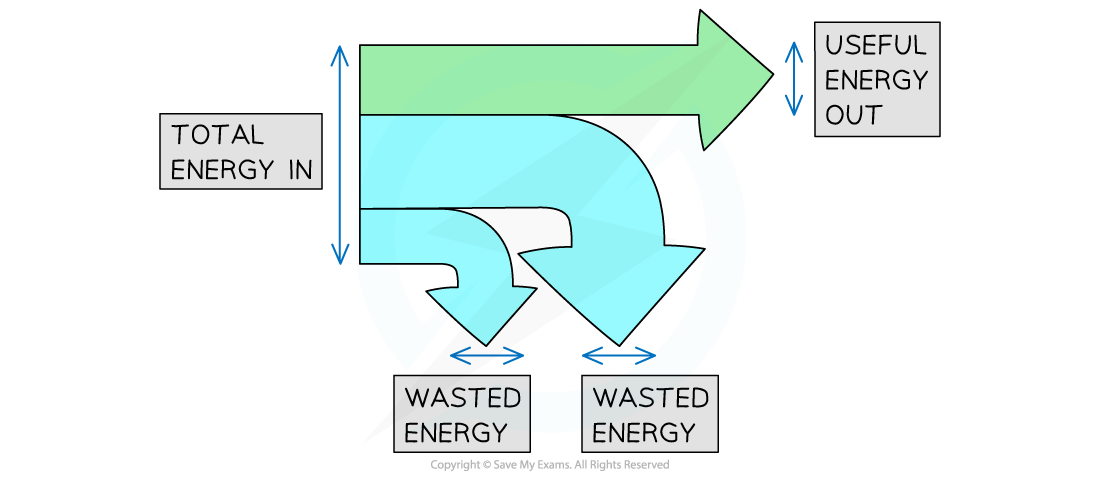
Total energy in, wasted energy and useful energy out shown on a Sankey diagram
The width of each arrow is proportional to the amount of energy being transferred
As a result of the conservation of energy:
total energy in = total energy out
total energy in = useful energy out + wasted energy
A Sankey diagram for a modern efficient light bulb will look very different from that for an old filament light bulb
A more efficient light bulb has less wasted energy
This is shown by the smaller arrow downwards representing the energy transferred to the thermal store of the surroundings
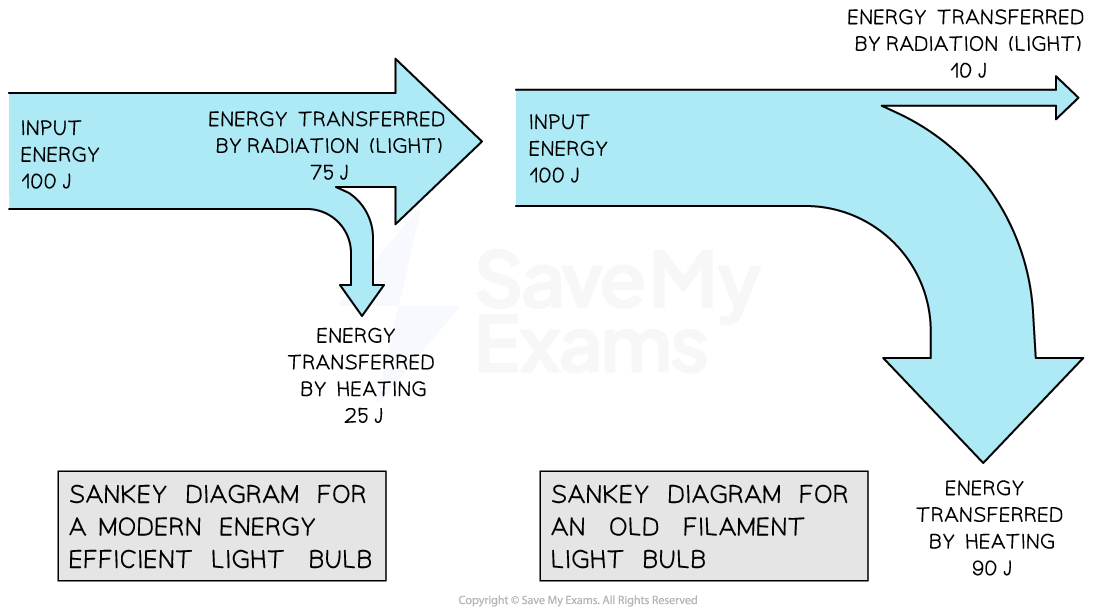
Sankey diagrams showing filament bulbs have a much greater proportion of wasted energy than modern energy efficient bulbs
Worked Example
An electric motor is used to lift a weight. The diagram represents the energy transfers in the system.
Calculate the amount of wasted energy.
[2]
Answer:
Step 1: State the conservation of energy
Energy cannot be created or destroyed, it can only be transferred from one store to another
Step 2: Rearrange the equation for the wasted energy
[1 mark]
Step 3: Substitute the values from the diagram
[1 mark]
Examiner Tips and Tricks
Since arrow width is proportional to the amount of energy being transferred, scaled diagrams and grid lines can be used to measure the amount of energy represented by an arrow.
When energy is wasted in more than one way on a Sankey diagram, remember to add each wasted arrow to get the total wasted energy.
Unlock more, it's free!
Join the 100,000+ Students that ❤️ Save My Exams
the (exam) results speak for themselves:
Was this revision note helpful?
Build on this topic
