IGCSEPhysicsCIERevision Notes1. Motion, Forces & EnergyMomentumMomentum

Momentum (CIE IGCSE Physics)

Revision Note

Test yourselfFlashcards
Leander

Author

Leander

Expertise

Physics

Did this video help you?

Momentum

Extended tier only

  • An object with mass that is in motion has momentum 

The momentum equation

  • Momentum is defined by the equation:

momentum space equals space mass space cross times space velocity

p space equals space m v

  • Where:
    • p = momentum, measured in kilogram metres per second (kg m/s)
    • m = mass in kilograms (kg)
    • v = velocity in metres per second (m/s)

  • This means that an object at rest (i.e. v = 0) has no momentum

  • Momentum keeps an object moving in the same direction
    • It is difficult to change the direction of an object that has a large momentum
  • Velocity is a vector with both magnitude and direction
    • This means that the momentum of an object also depends on its direction of travel
    • Therefore, momentum can be either positive or negative
  • If an object has positive momentum, then an object travelling in the opposite direction will have negative momentum 

How does the momentum of a ball change after a collision?

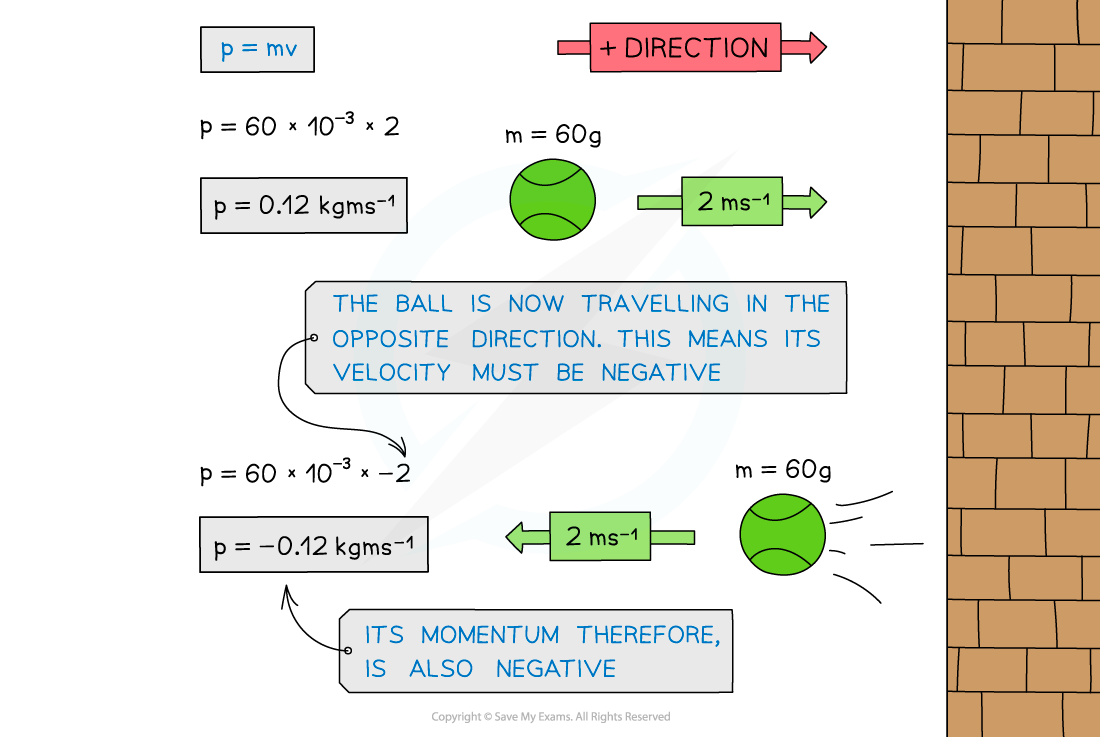
Negative momentum, downloadable AS & A Level Physics revision notes

The momentum of the tennis ball is positive as it approaches the wall and negative after the collision, as it moves in the opposite direction

Worked example

Determine which object has the most momentum.

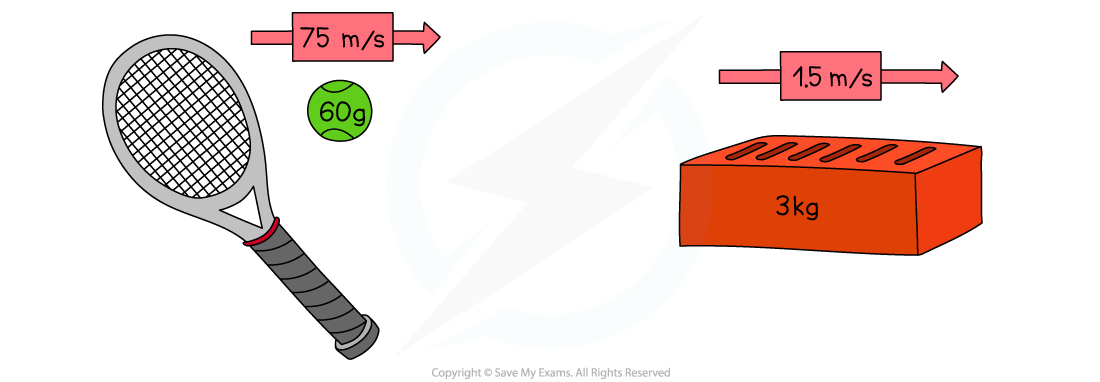
WE - Momentum comparison question image, downloadable AS & A Level Physics revision notes

Answer:

Step 1: Determine the momentum of the tennis ball using the momentum equation

p space equals space m v

p space equals space 0.06 space cross times space 75

p space equals space 4.5 space kg space straight m divided by straight s

Step 2: Determine the momentum of the brick using the momentum equation

p space equals space m v

p space equals space 3 space cross times space 1.5

p space equals space 4.5 space kg space straight m divided by straight s

Step 3: Compare the momentum of each object

  • Both the tennis ball and the brick have the same momentum
  • Even though the brick is much heavier than the ball, the ball is travelling much faster than the brick
  • This means that on impact, they would both exert a similar force (depending on the time it takes for each to come to rest)

Exam Tip

You can remember momentum as mass in motion. The units of momentum are kg m/s which is the product of the units of mass (kg) and velocity (m/s).

Which direction is taken as positive is completely up to you in the exam, as long as you are consistent throughout a question. In general, the right and upwards are taken as positive, and down or to the left as negative.

Did this video help you?

Conservation of momentum

Extended tier only

  • The principle of conservation of momentum states that:

In a closed system, the total momentum before an event is equal to the total momentum after the event

  • A system, in physics, is an object or group of objects
  • A closed system means that no energy is transferred into or out of the system and there are no external forces acting

  • The principle of conservation of momentum can also be written as:

The total momentum before a collision = The total momentum after a collision

  • Since momentum is a vector quantity, a system of objects moving in opposite directions (e.g. towards each other) at the same speed will have an overall momentum of 0 since they will cancel out
    • Momentum is always conserved over time

  • The diagram below shows two masses m with velocity u and M at rest (ie. zero velocity)

Principle of conservation of momentum for a collision

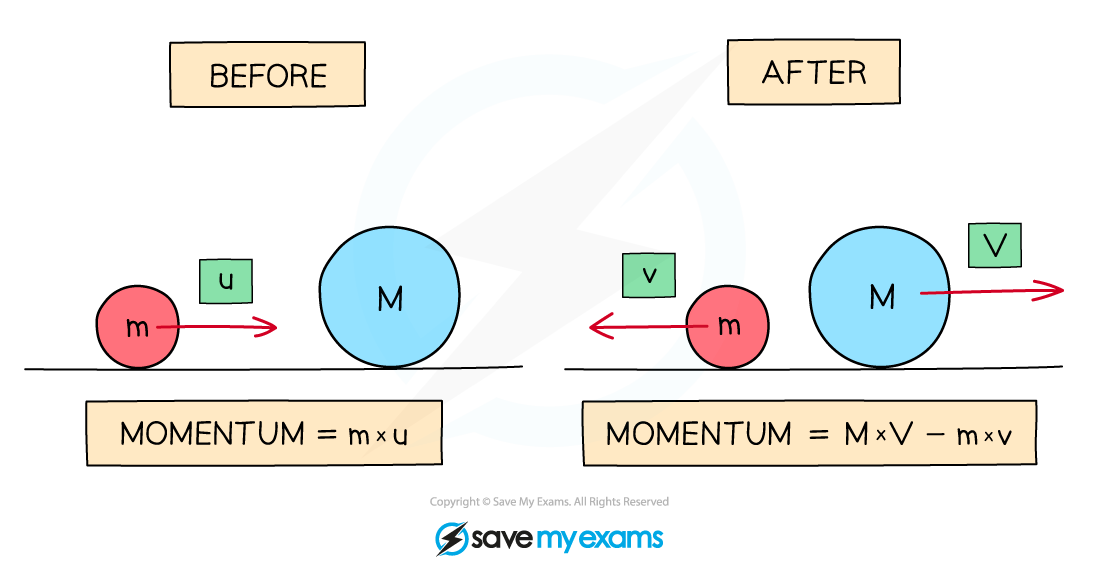
conservation-of-momentum, IGCSE & GCSE Physics revision notes

The momentum of a system before and after a collision is constant

  • Before the collision:
    • The momentum is only of mass m which is moving
    • If the right is taken as the positive direction, the total momentum of the system is m × u

  • After the collision:
    • Mass M also now has momentum
    • The velocity of m is now -v (since it is now travelling to the left) and the velocity of M is V
    • The total momentum is now the momentum of M + momentum of m
    • This is (M × V) + (m × -v) or (M × V) – (m × v)

Worked example

The diagram shows a car and a van, just before and just after the car collided with the van, which is initially at rest.

WE Conservation of Momentum Question image, downloadable IGCSE & GCSE Physics revision notes

Use the idea of conservation of momentum to calculate the velocity of the van when it is pushed forward by the collision.

Answer:

Step 1: State the principle of conservation of momentum

total space momentum space before space equals space total space momentum space after

Step 2: Calculate the total momentum before the collision

p space equals space m v

  • Momentum of the car

p space equals space 990 space cross times space 10

p space equals space 9900 space kg space straight m divided by straight s

  • Momentum of the van
    • The van is at rest
    • Therefore, v = 0 m/s
    • Therefore, p = 0 kg m/s
  • Total momentum before collision

p subscript b e f o r e end subscript space equals space 9900 space plus space 0 space equals space 9900 space kg space straight m divided by straight s

Step 3: Calculate the total momentum after the collision

  • Momentum of the car

p space equals space 990 space cross times space 2

p space equals space 1980 space kg space straight m divided by straight s

  • Momentum of the van

p space equals space 4200 space cross times space v

  • Total momentum after collision

p subscript a f t e r end subscript space equals space 1980 space plus space 4200 v

Step 4: Rearrange the conservation of momentum equation to solve for v

p subscript b e f o r e end subscript space equals space p subscript a f t e r end subscript

9900 space equals space 1980 space plus space 4200 v

9900 space minus space 1980 space equals space 4200 v

v space equals space fraction numerator 9900 space minus space 1980 over denominator 4200 end fraction

v space equals space 1.9 space straight m divided by straight s

Exam Tip

If it is not given in the question already, drawing a diagram of before and after helps keep track of all the masses and velocities (and directions) in the conversation of momentum questions.

Did this video help you?

You've read 0 of your 10 free revision notes

Unlock more, it's free!

Join the 100,000+ Students that ❤️ Save My Exams

the (exam) results speak for themselves:

    Test yourselfFlashcardsNext topic

    Did this page help you?

    Leander

    Author: Leander

    Leander graduated with First-class honours in Science and Education from Sheffield Hallam University. She won the prestigious Lord Robert Winston Solomon Lipson Prize in recognition of her dedication to science and teaching excellence. After teaching and tutoring both science and maths students, Leander now brings this passion for helping young people reach their potential to her work at SME.