Calculating with Vectors (Cambridge (CIE) IGCSE Physics)

Calculations with vectors

Extended tier only

• Vectors can be drawn using vector diagrams

Vector diagrams

• Vectors are represented by an arrow

  • The length of the arrow represents the magnitude 

  • The direction of the arrow indicates the direction 

  • the scale of the arrows should be proportional to the relative magnitudes of the forces

    • an arrow for a 4 N force should be twice as long as an arrow for a 2 N force

Vector diagram of two forces acting on an object

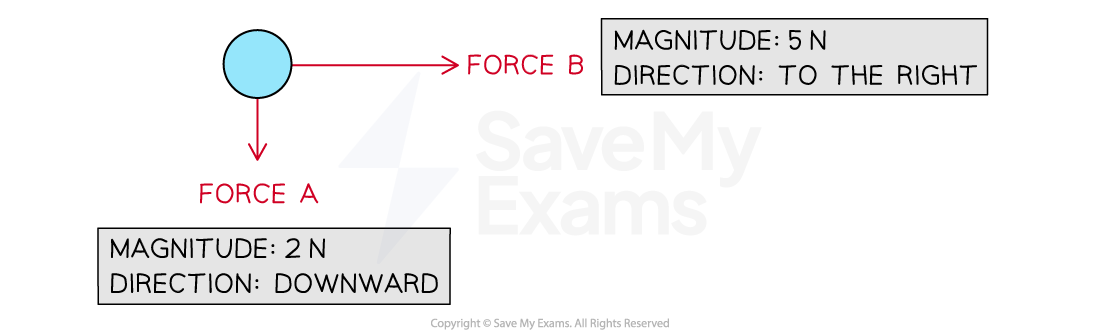
The length of the arrows are proportional to the magnitude of the forces, and show the direction that forces act in

Calculating vectors graphically

• Vector diagrams can be used to combine vectors

• Vectors at right angles to one another can be combined into one resultant vector
  

  • The resultant vector will have the combined effect of the two original vectors

  • For example, a resultant force vector will have the combined effect of two component forces

• Component vectors are sometimes drawn with a dotted line and a subscript indicating horizontal or vertical

  • A force , for example, may have two components:

    • is the vertical component of the force 

    • is the horizontal component of force 

• To calculate vectors graphically means carefully producing a scale drawing with all lengths and angles correct

  • This should be done using a sharp pencil, ruler and protractor

• Follow these steps to carry out calculations with vectors on graphs

1. Choose a scale which fits the page

   • For example, use 1 cm = 10 m or 1 cm = 1 N, so that the diagram is around 10 cm high

2. Draw the vectors at right angles to one another

3. Complete the rectangle

4. Draw the resultant vector diagonally from the origin

5. Carefully measure the length of the resultant vector

6. Use the scale factor to calculate the magnitude

7. Use the protractor to measure the angle

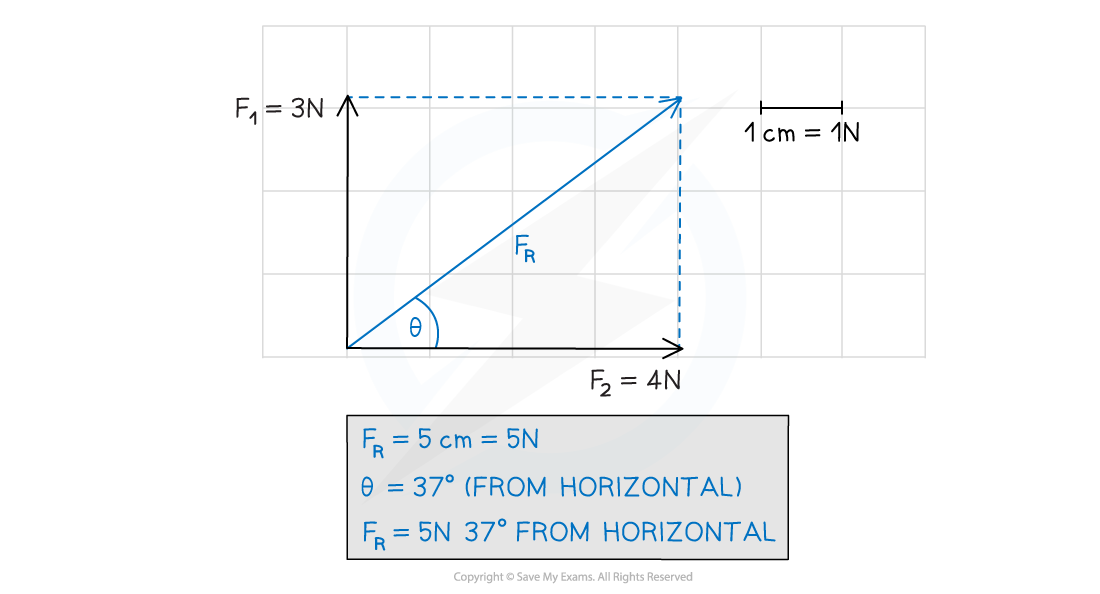
Vectors can be measured or calculated graphically using scaled vector diagrams

Combining vectors by calculation

• In this method, a vector diagram is still essential but it does not need to be exactly to scale

• The vector diagram can take the form of a sketch, as long as the resultant side, component sides are clearly labelled

Using a vector diagram to resolve two force vectors F₁ and F₂ into a resultant force vector F_R

• When the magnitude of only one vector is known, and the angle is known, then trigonometry can be used to find the magnitude of the missing vector

  • The mnemonic 'soh-cah-toa' can used to remember the trigonometric functions

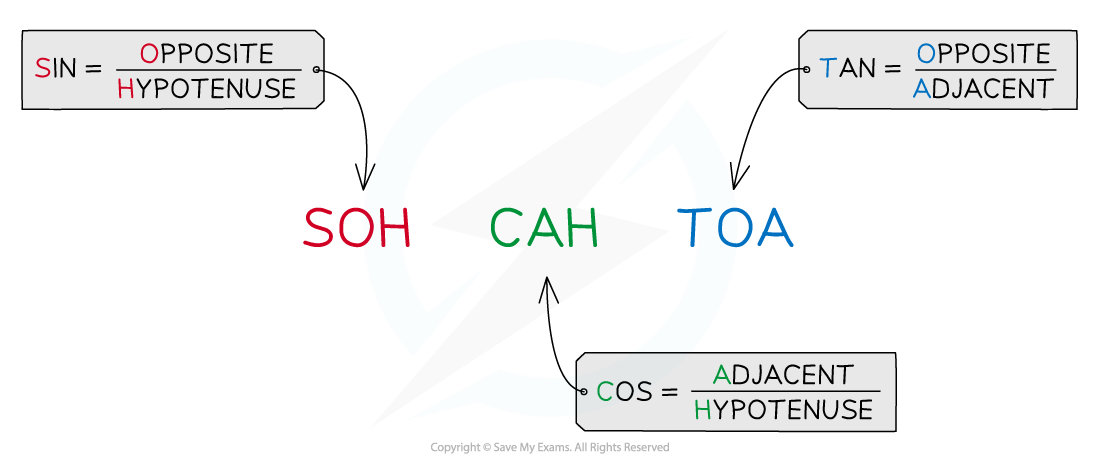
Trigonometry can be used when the magnitude of one vector and the angle is known

• When the magnitudes of two of the vectors are known, then Pythagoras' theorem can be used to find the magnitude of the missing vector

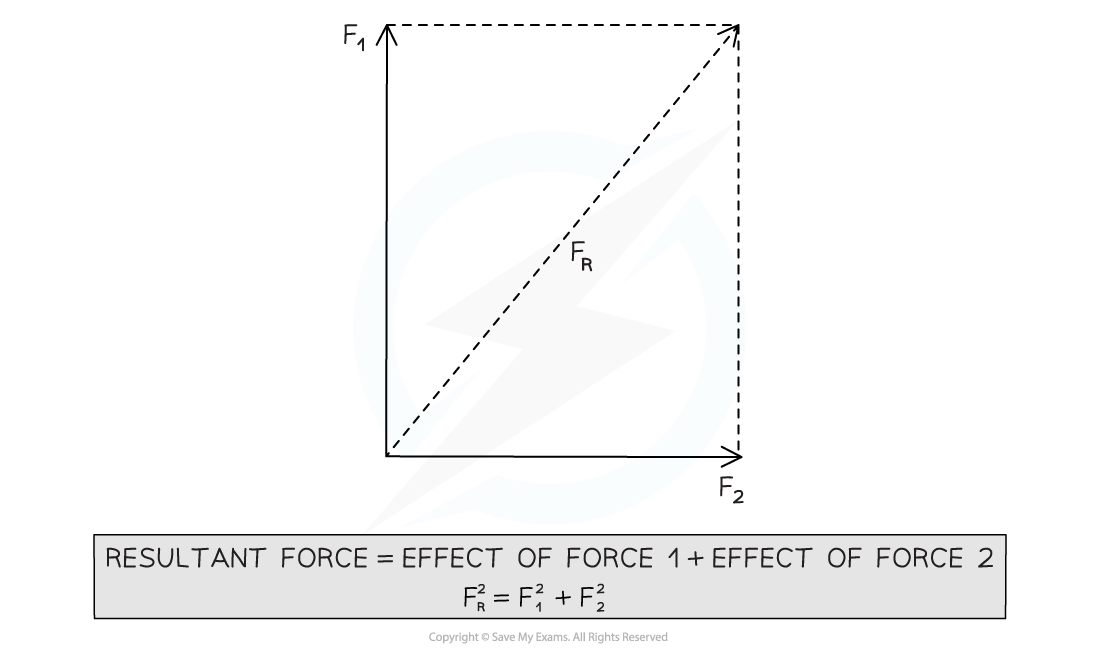
Pythagoras's theorem can be used when the magnitudes of two of the three vectors are known