Reading & Interpreting Statistical Diagrams (Cambridge (CIE) IGCSE Maths): Revision Note
Exam code: 0580 & 0980
Reading & interpreting statistical diagrams
How do I interpret statistical diagrams?
Read and understand the initial sentences describing the situation (context)
Underline important words if necessary
Look for any keys that may help you to understand the diagram
For example
1 unit represents 20 people
Year 10 is the solid line, Year 11 is the dotted line
Class A is shaded, class B is striped
Read the titles of diagrams and all axes labels
A graph for 'new students at a school' is different to 'all students at the school'
A frequency axis that starts at 50 is different to one starting at 0
Understand which units are being used
Individual lengths may be in centimetres but total length may be in metres
Populations may be measured in thousands
Look out for any extreme values (outliers / anomalies)
One month's temperature might be unusually high
Was it a heat wave or a recording error?
How do I draw conclusions from diagrams?
Look for overall trends in the diagram
Prices increase year on year
The temperature peaks in June
Use numbers from the graphs
Refer to any changes
The steepness (gradient) of graph may change
Write in full sentences that copy the exact wording from the question
'The number of goats in farm A has decreased by 12 over the 8 month period'
Not 'There are fewer of them now'
You may need to calculate the mode, median, mean or range to support any explanations
Understand why drawing conclusions may not be suitable
The data set may be too small to be representative
The data set may be biased
Consider the scope of the data
e.g. Data for January to March cannot be used to predict August
Worked Example
The diagram below shows the temperature of a garden in the UK, recorded at 7am on each day of a particular week in March.
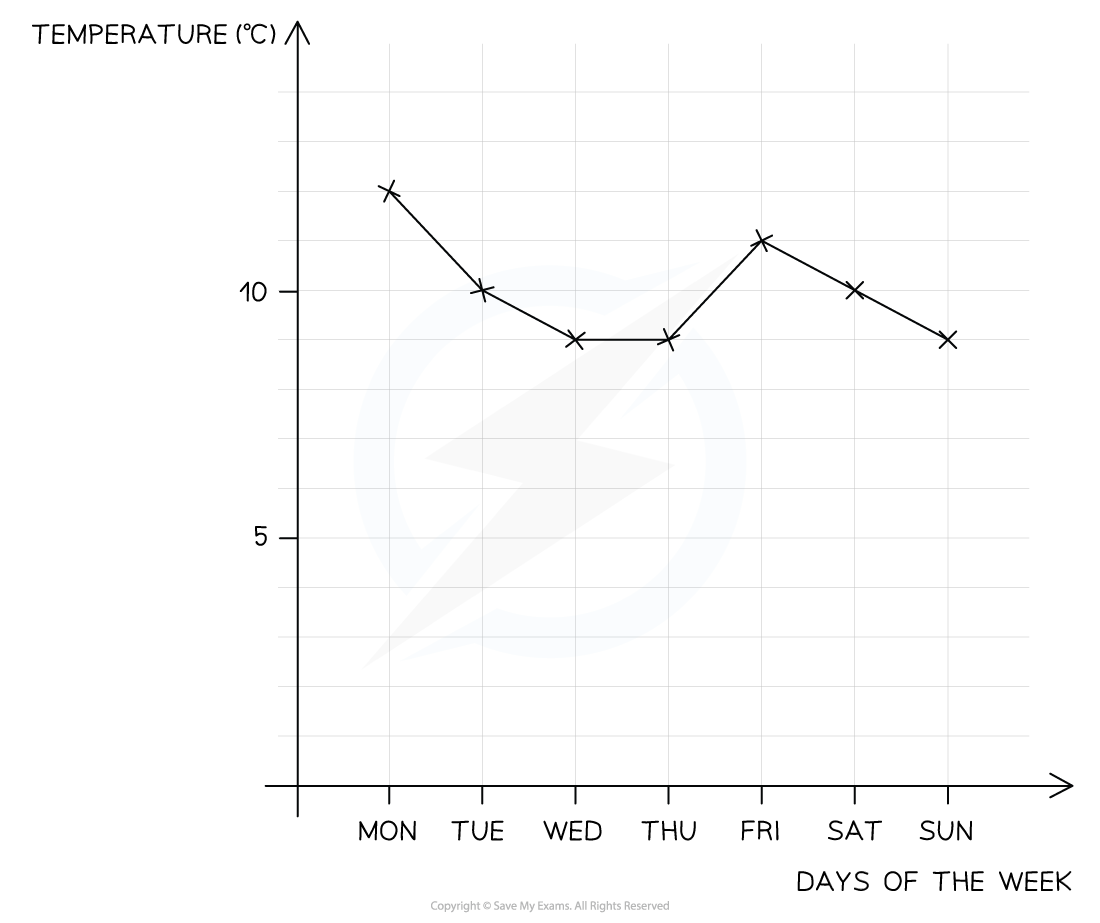
(a) Describe the change in temperature over the first four days.
The trend shows a decrease in the first three days, then a constant temperature
Find numbers from the graph to use in your answer
Refer to the steepness of the change
The temperature decreases from 12°C on Sunday to 9°C on Wednesday
The decrease is steeper over the first two days
There is then a constant temperature of 9°C on both Wednesday and Thursday
(b) A gardener claims that, based on the graph, Monday must have experienced the highest temperature that week.
Give a reason as to why this might not be true.
Reread the information at the start
These temperatures were recorded at 7am in the morning (we don't know how hot the rest of the day was)
The temperatures on the graph are at 7am each day
The maximum temperature may have been after 7am, on a day that was not Monday
(c) A journalist wants to use the data shown to claim that the average temperature that week was below 10°C.
The mean of the temperatures shown is 10°C.
Which type of average would you suggest they use? Explain your answer.
The mean of 10°C does not support the claim that the average temperature is below 10°C
Try calculating the mode instead
12, 10, 9, 9, 11, 10, 9
The most frequent number is 9
The modal temperature is 9°C
9°C < 10°C so using the mode would help the journalist's claim
You could try the median, but it is also 10°C

You've read 0 of your 5 free revision notes this week
Unlock more, it's free!
Did this page help you?