Revision NotesExam QuestionsFlashcardsPast Papers
IGCSEMathsCambridge (CIE)ExtendedRevision NotesPythagoras & Trigonometry3D Pythagoras & Trigonometry3D Pythagoras & Trigonometry

3D Pythagoras & Trigonometry (Cambridge (CIE) IGCSE Maths)

Revision Note

Amber

Written by: Amber

Reviewed by: Dan Finlay

Cambridge (CIE) Maths: ExtendedEdexcel Maths A (Modular): Higher Unit 1Cambridge (CIE) International Maths: ExtendedEdexcel Maths A: Higher

3D Pythagoras & Trigonometry

How do I use Pythagoras' theorem in a 3D shape?

  • You can often find right-angled triangles within 3D shapes

    • If two sides of the triangle are known, you can use Pythagoras’ theorem

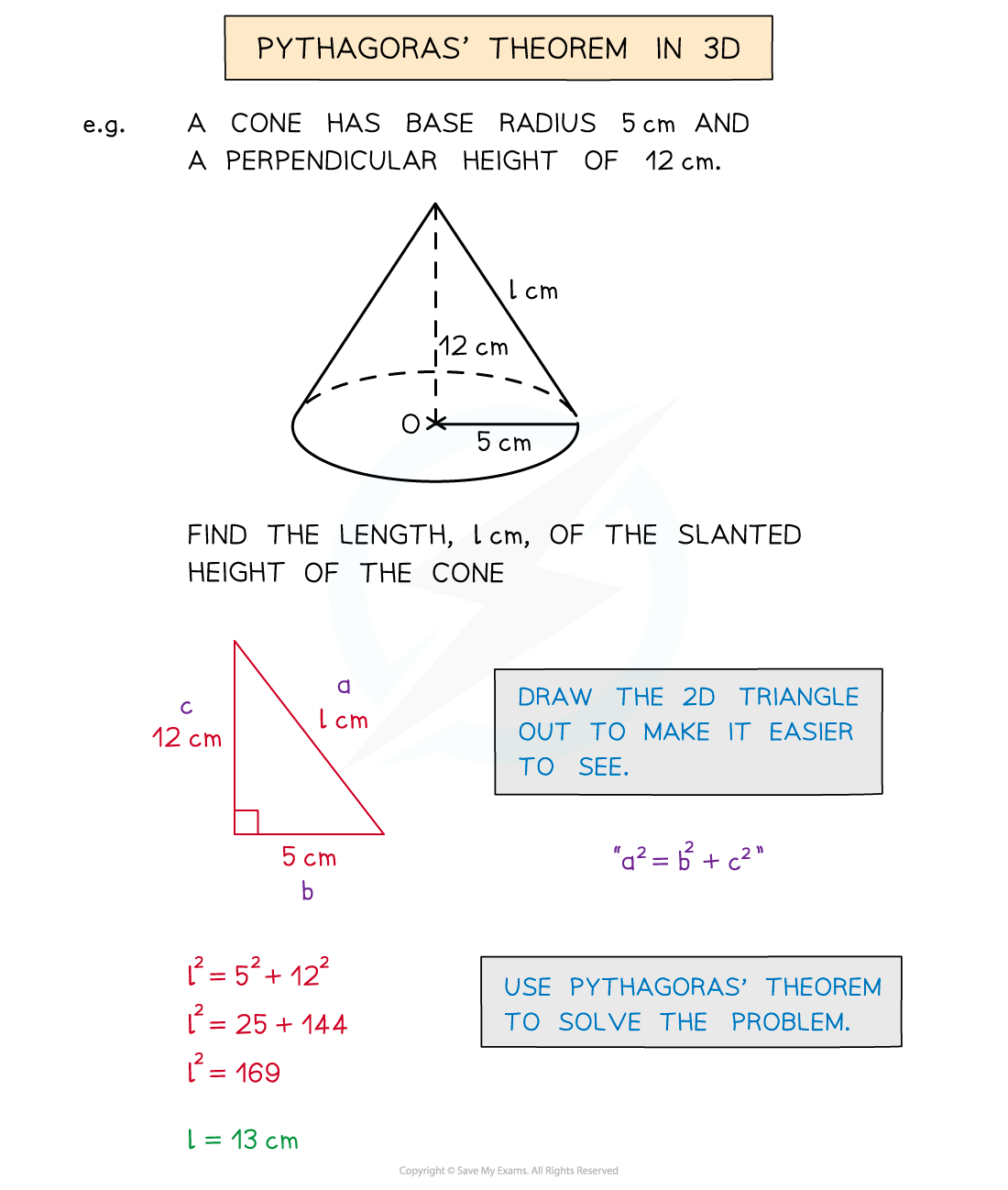
Example showing Pythagoras' theorem used to find the slant height of a cone given its radius and perpendicular height.

Is there a 3D version of the Pythagoras' theorem formula?

  • There is a 3D version of Pythagoras’ theorem: d squared equals x squared plus y squared plus z squared

    • d is the distance between two points

    • x comma space y and z are the distances in the three different perpendicular directions between the two points

Example showing Pythagoras' theorem to find the diagonal in a cuboid (3D formula).

  • However, all 3D situations can be broken into two 2D problems

    • Form two right-angle triangles

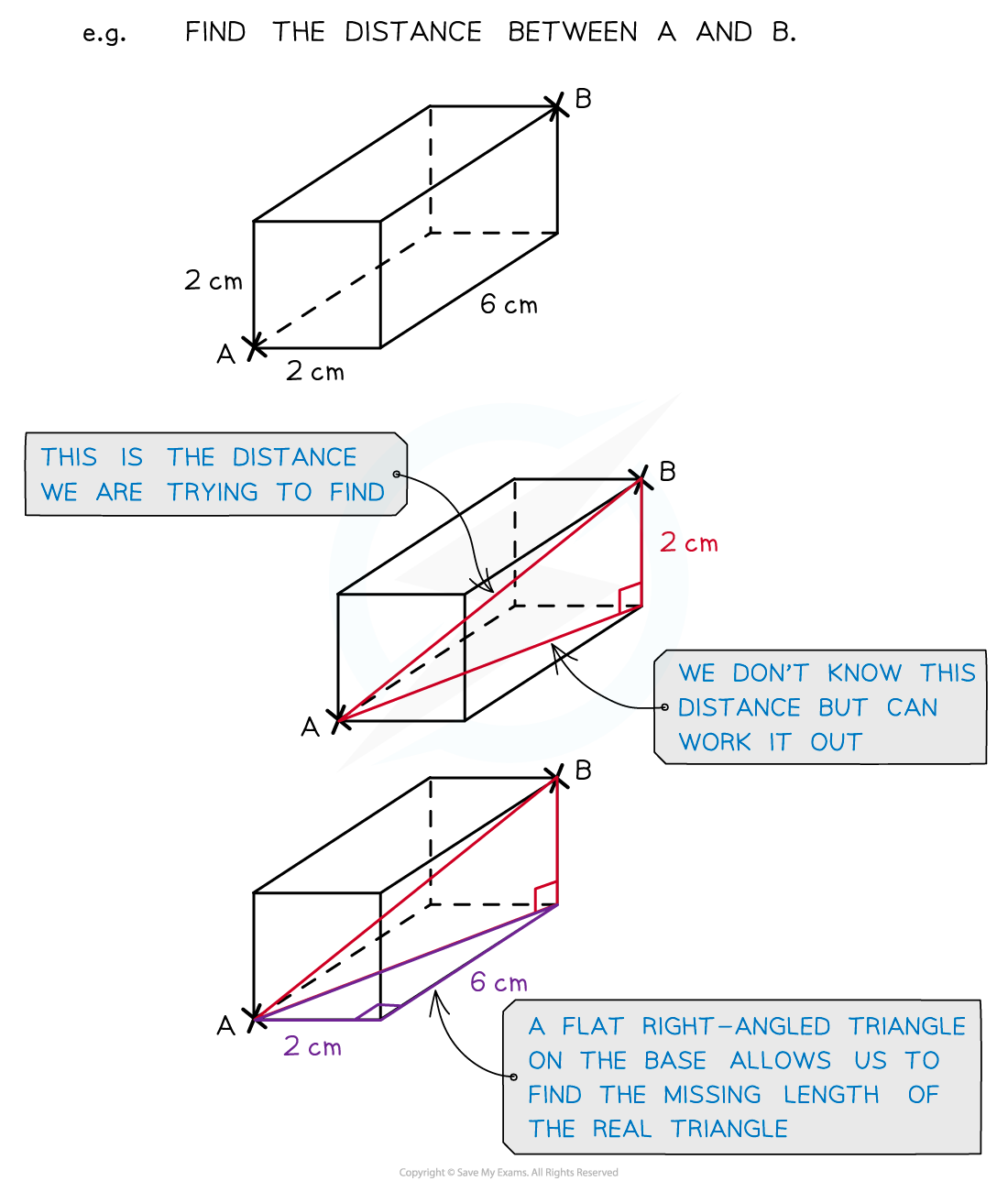
Example showing Pythagoras' theorem to find the diagonal in a cuboid (splitting into two 2D triangles).
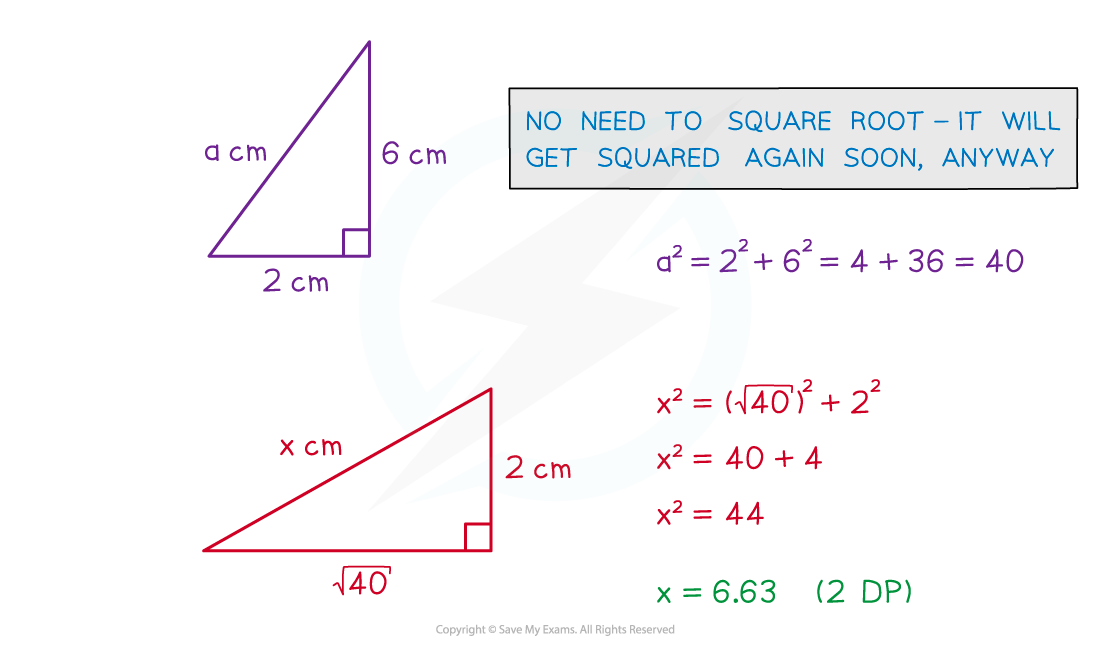
3DPythagTrig Notes fig3 (3), downloadable IGCSE & GCSE Maths revision notes

Examiner Tips and Tricks

  • You are not given the 3D Pythagoras formula in the exam

    • You can always split 3D problems into two 2D problems (which don't need this formula)

How do I use SOHCAHTOA in 3D?

  • Again, look for right-angled triangles to use with SOHCAHTOA

    • You may need combinations of triangles that lead to the missing side or angle

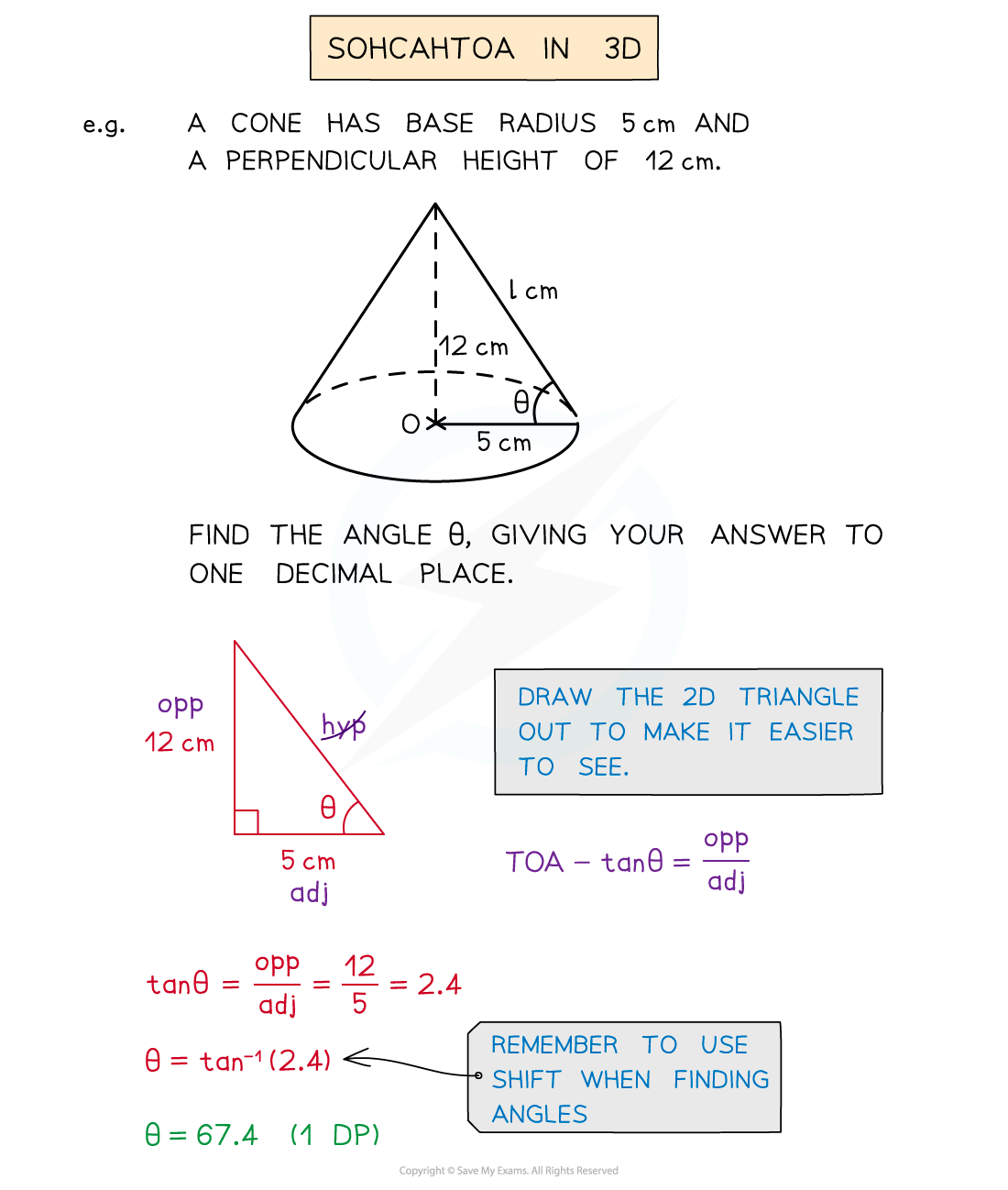
Example showing SOHCAHTOA to find the angle between the base and the slant height of a cone , given its radius and perpendicular height.

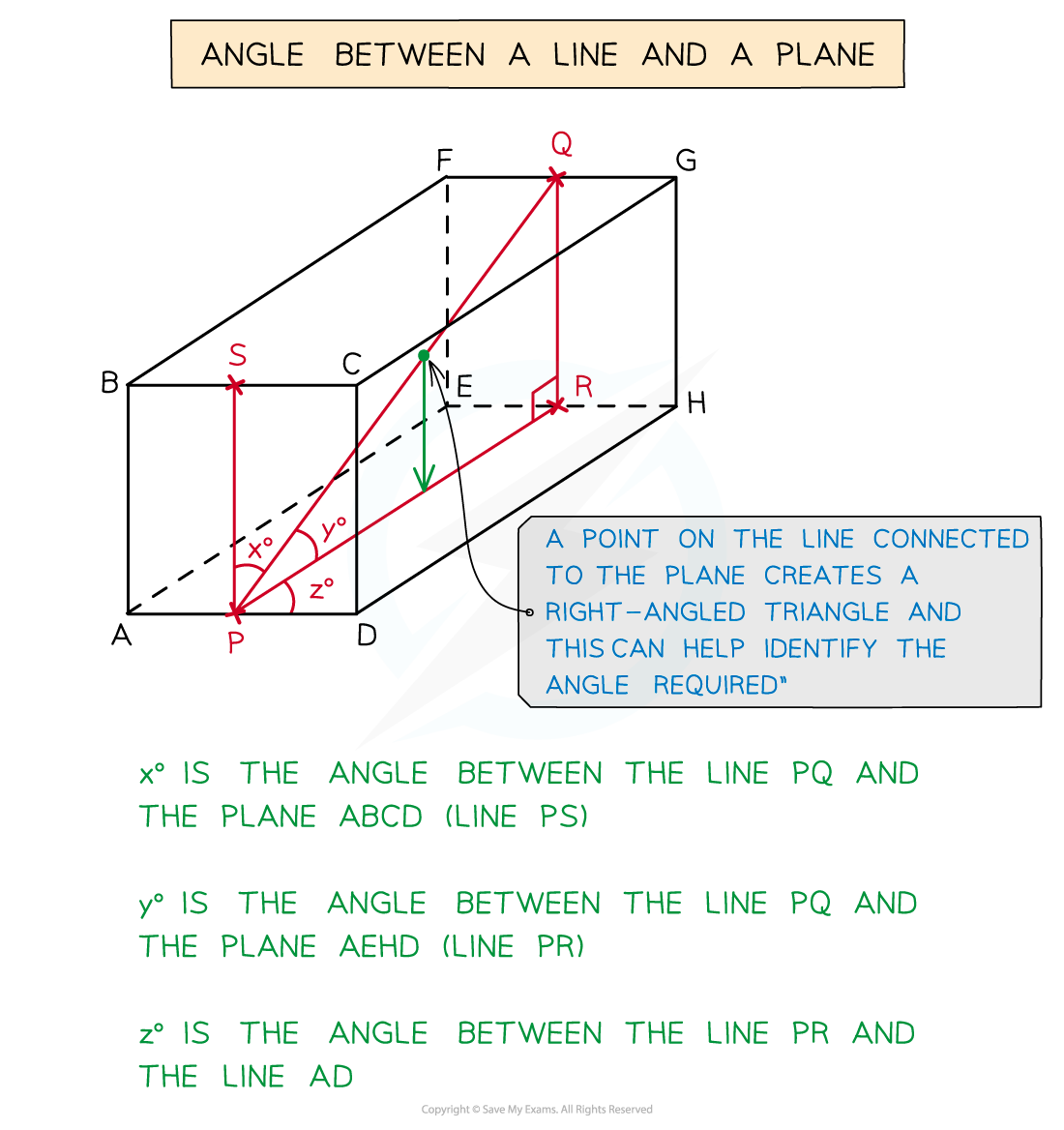
How do I find the angle between a line and a plane?

  • To find the angle between a line and a plane:

    • first identity the plane in the question

    • then draw on the line in the question (if it is not already drawn on)

    • form a right-angled triangle between the line and plane

      • The height of the triangle must be perpendicular to the plane

    • then use SOHCAHTOA

  • The angle between a line and a plane is sometimes not obvious

    • If unsure, put a different point on the same line and see if it helps

      • Try to create a right-angled triangle

    • Some people like to imagine the line as a fishing rod, from which they lower the hook (and fishing line) vertically down to the plane!

      • Others like to imagine they are sitting inside the 3D object, looking around it (like corners of a room)

Diagram showing how to find the angle between a line and a plane.

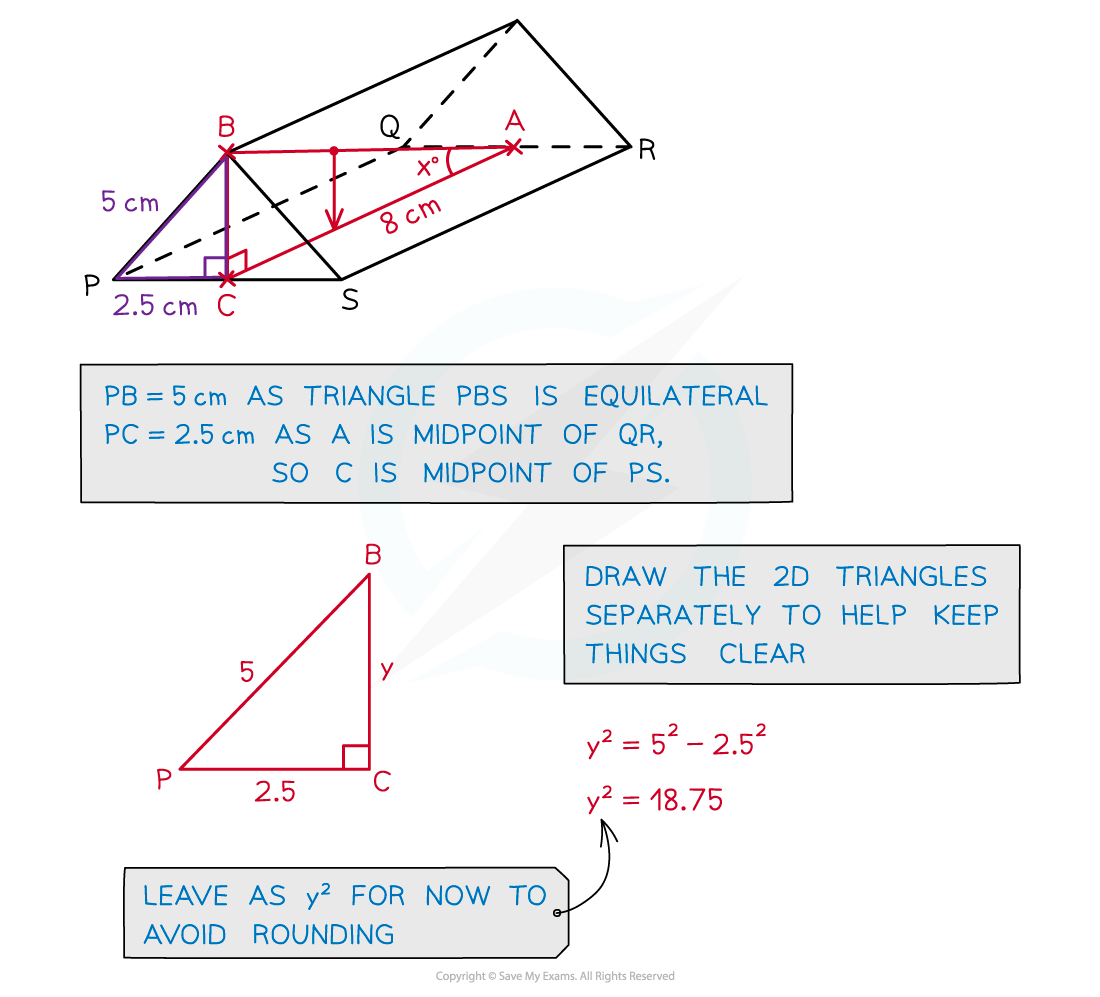
How do I apply 3D Pythagoras and trigonometry to more complicated problems?

  • Always split up a complicated problem into 2D right-angled triangles

    • Some questions may requires more than one 2D right-angled triangle

  • Some 2D triangles on the diagram are still drawn in 3D

    • It helps to redraw these 2D triangles flat on the page (not at angles)

    • You can then spot any uses of Pythagoras' theorem and SOHCAHTOA

Example showing SOHCAHTOA to find the angle between a line and the base of a triangular prism. (Image 1).
Example showing SOHCAHTOA to find the angle between a line and the base of a triangular prism. (Image 2).
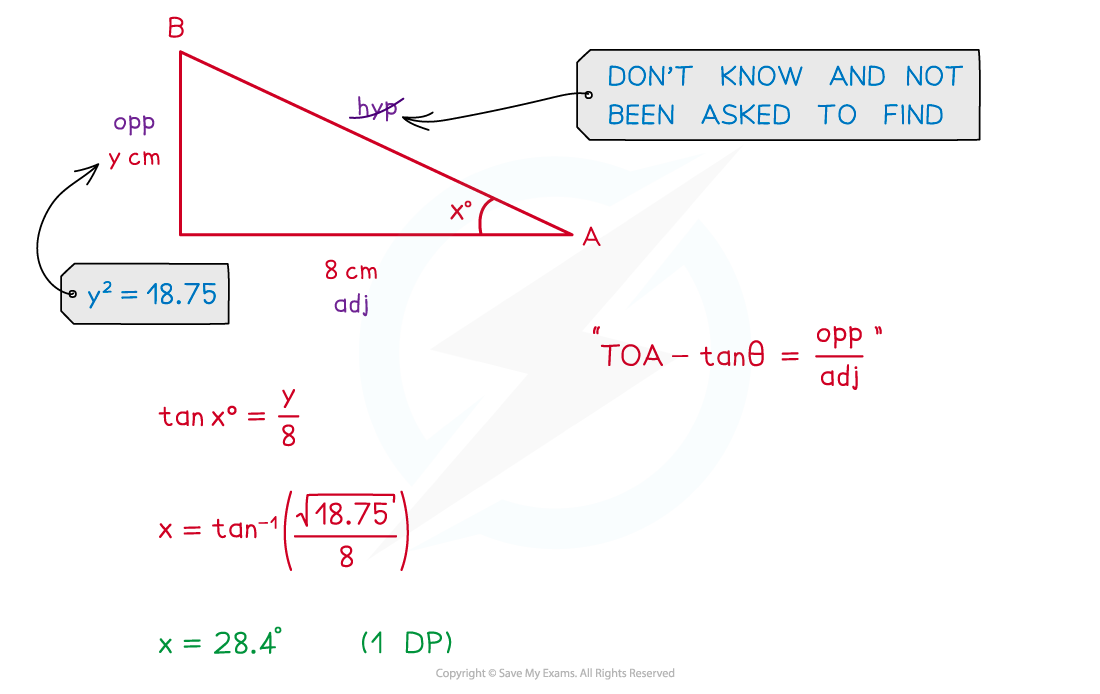
Example showing SOHCAHTOA to find the angle between a line and the base of a triangular prism. (Image 3).

Examiner Tips and Tricks

  • If you are stuck in the exam with a complicated 3D diagram, it is always better to just start finding any lengths and angles in the shape, as:

    • these may end up being useful

    • you may score more marks than if you had left the question blank

Worked Example

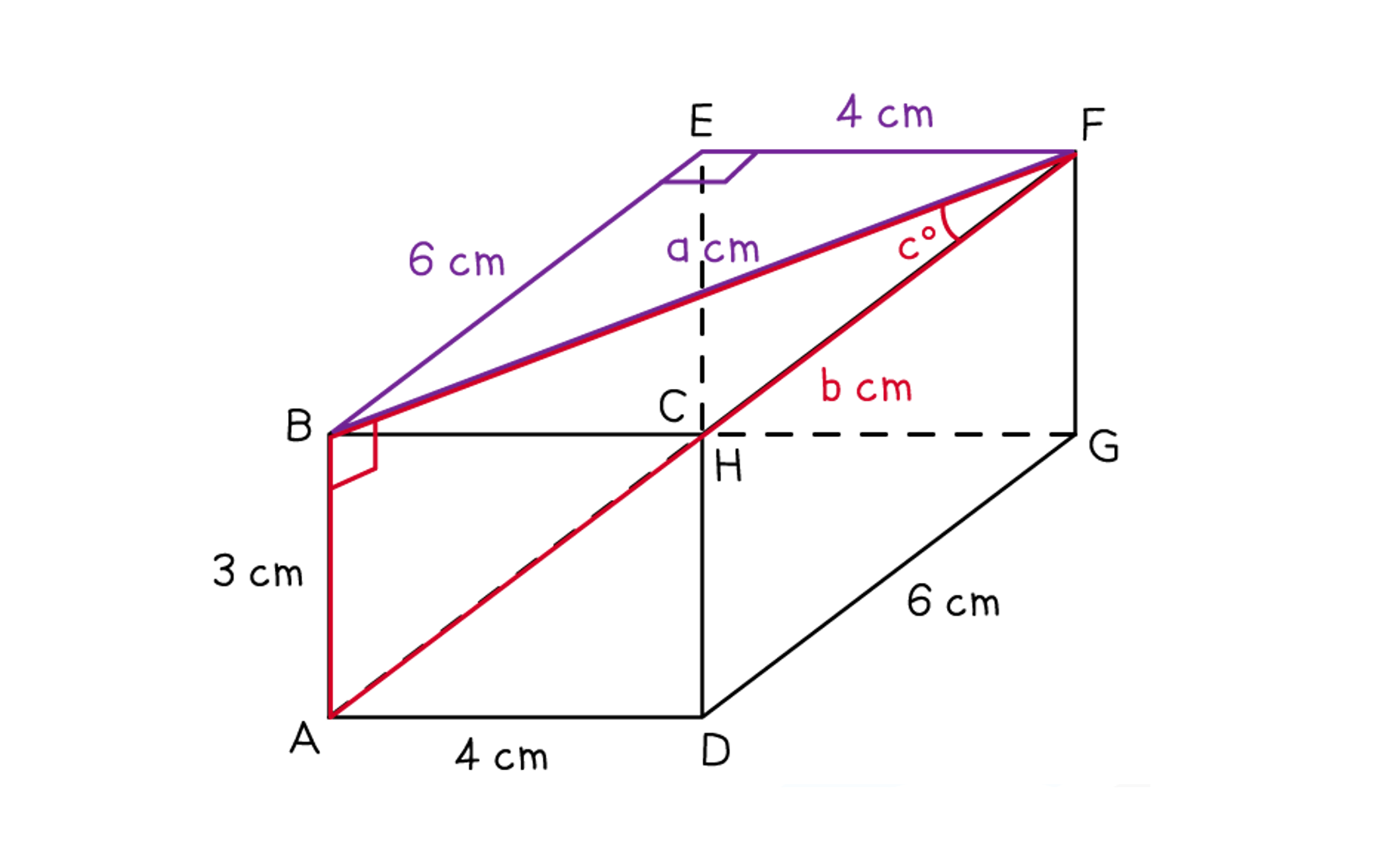
A pencil is being put into a cuboid shaped box.

The box has dimensions 3 cm by 4 cm by 6 cm.

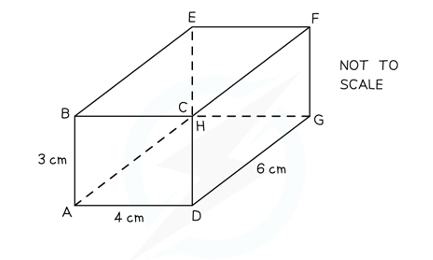
A cuboid ABCDEFGH. AB = 3 cm, AD = 4 cm, DG = 6 cm.

(a) Find the length of the longest pencil that can fit inside the box.

The longest possible pencil will fit between diagonally opposite vertices, e.g. AF

Form a 2D right-angled triangle, such as triangle ABF

Cuboid ABCDEFGH with a right-angled triangle ABF highlighted and another right-angled triangle BEF also highlighted.

Method 1

To find the length AF, there are a few different options
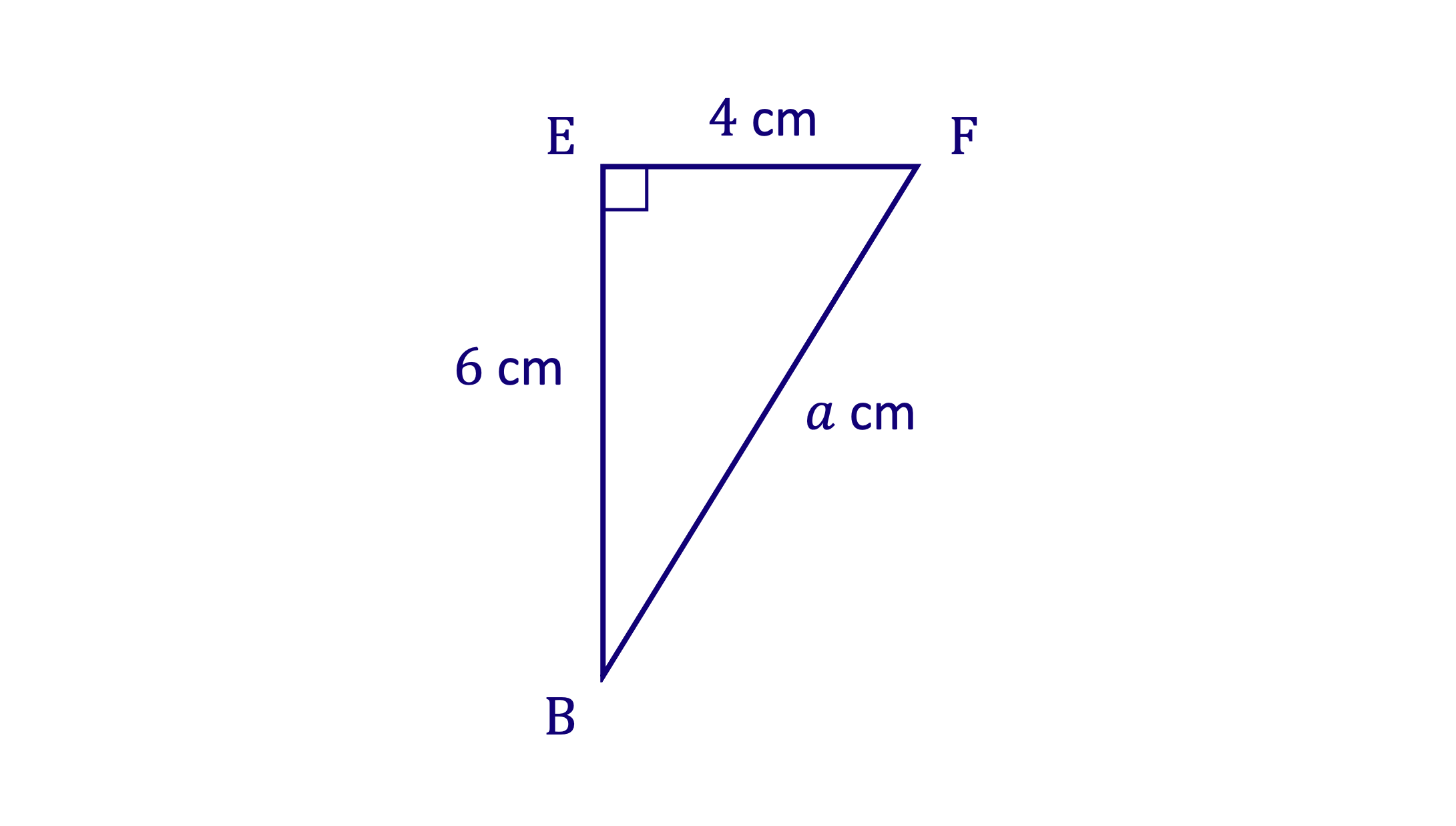
One option is to find length BF (from triangle BEF) then AF (from triangle ABF)
Draw triangle BEF flat and use Pythagoras' theorem to find BF

Triangle BEF, BE = 6 cm, EF = 4 cm, BF = a cm.

a squared equals 4 squared plus 6 squared a squared equals 16 plus 36 a squared equals 52

Draw triangle ABF flat and use Pythagoras' theorem to calculate AF

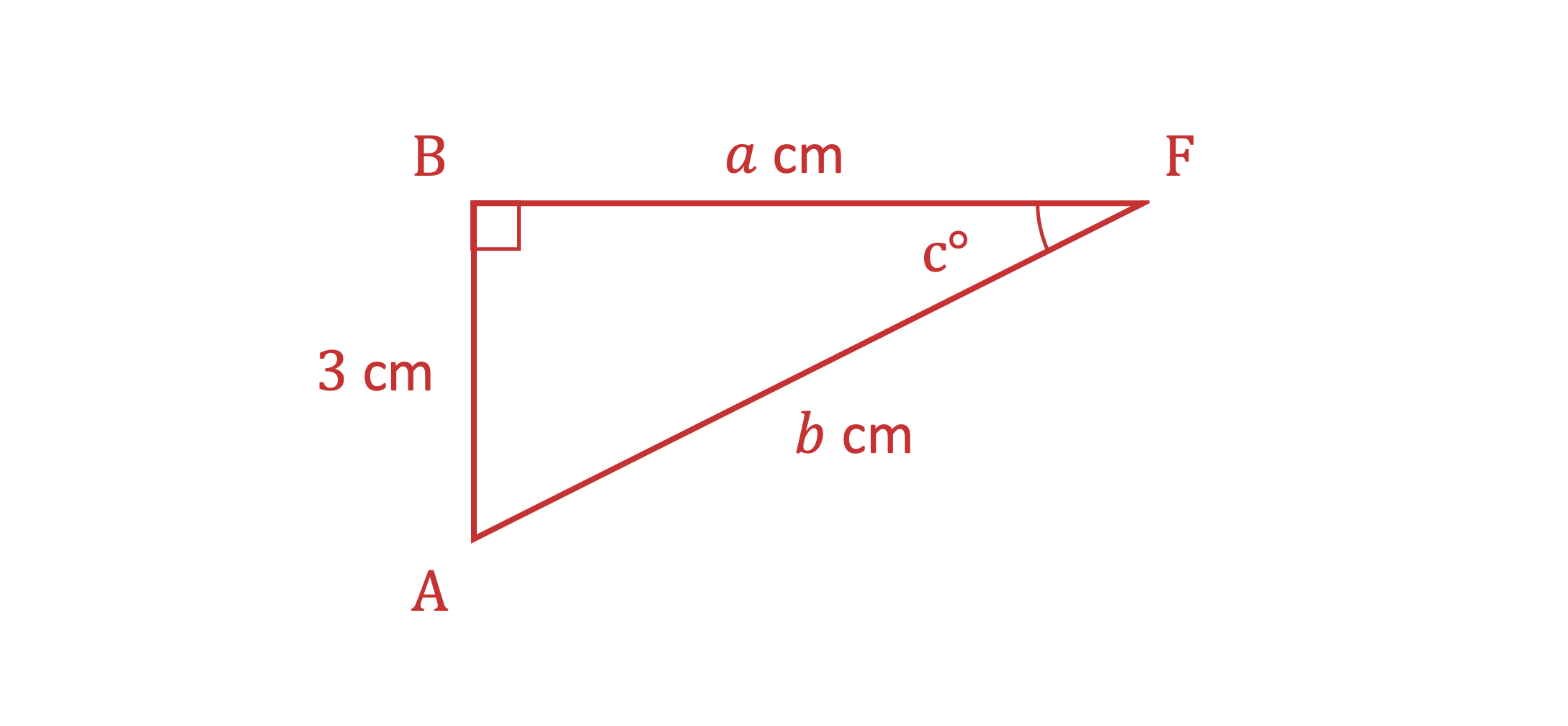
Triangle ABF. AB = 3 cm, BF = a cm and AF = b cm.

table row cell b squared end cell equals cell 3 squared plus a squared end cell row cell b squared end cell equals cell 9 plus 52 end cell row cell b squared end cell equals 61 row b equals cell square root of 61 equals 7.81024... end cell end table

The longest pencil that can fit inside the box is 7.81 cm (to 3 s.f.)

Method 2

Apply the 3D version of Pythagoras’ theorem: d squared equals x squared plus y squared plus z squared

The distance in the x direction is 4 cm
The distance in the y direction is 6 cm
The distance in the z direction is 3 cm

table row cell d squared end cell equals cell 4 squared plus 6 squared plus 3 squared end cell row d equals cell square root of 4 squared plus 6 squared plus 3 squared end root end cell row d equals cell square root of 61 equals 7.81024... end cell end table

The longest pencil that can fit inside the box is 7.81 cm (to 3 s.f.)

(b) Find the angle that the pencil would make with the plane BEFC.

The plane BEFC is the horizontal top surface of the box

To see the angle between AF and the plane, form a triangle with side AB (which is a height perpendicular to the plane)
The angle needed is marked c

Cuboid ABCDEFGH with a right-angled triangle ABF highlighted and another right-angled triangle BEF also highlighted.

Triangle ABF is the 2D triangle needed, but it is currently drawn in 3D
Draw triangle ABF flat on the paper and write on the lengths from part (a)

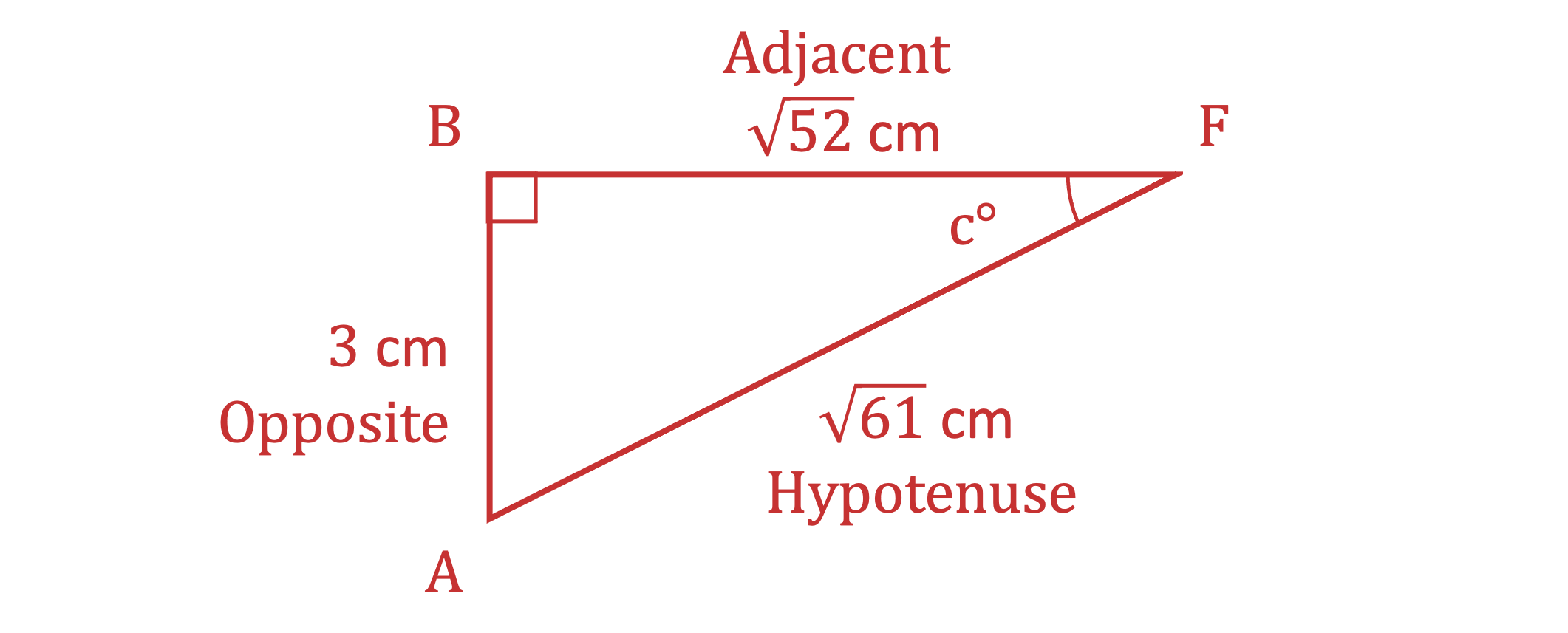
Triangle ABF with sides AB (opposite) = 3 cm, BF (adjacent) = √52 cm and AF (hypotenuse) = √61 cm.

This is now ready for SOHCAHTOA
We know all three sides so could use any trig ratio, for example tan space straight theta equals straight O over straight A
Use this to find angle c

table row cell tan space c end cell equals cell fraction numerator 3 over denominator square root of 52 end fraction end cell row c equals cell tan space to the power of negative 1 end exponent open parentheses fraction numerator 3 over denominator square root of 52 end fraction close parentheses end cell row c equals cell 22.58853... end cell end table

The angle between plane BEFC and the pencil is 22.6º (to 1 d.p.)

Last updated:

You've read 0 of your 5 free revision notes this week

Sign up now. It’s free!

Join the 100,000+ Students that ❤️ Save My Exams

the (exam) results speak for themselves:

    Test yourselfFlashcards

    Did this page help you?

    Previous:Deciding the Trig RuleNext:Trigonometric Graphs
    Author
    Reviewer
    Amber

    Author: Amber

    Expertise: Maths

    Amber gained a first class degree in Mathematics & Meteorology from the University of Reading before training to become a teacher. She is passionate about teaching, having spent 8 years teaching GCSE and A Level Mathematics both in the UK and internationally. Amber loves creating bright and informative resources to help students reach their potential.

    Dan Finlay

    Author: Dan Finlay

    Expertise: Maths Lead

    Dan graduated from the University of Oxford with a First class degree in mathematics. As well as teaching maths for over 8 years, Dan has marked a range of exams for Edexcel, tutored students and taught A Level Accounting. Dan has a keen interest in statistics and probability and their real-life applications.