Differentiation (Cambridge (CIE) IGCSE Maths: Extended): Revision Note

Differentiation

What is a gradient function?

• Recall that the equation of a curve gives the y-coordinate of a point when you substitute in its x-coordinate

  • For example, $y=x^2+3x+5$

    • Substitute $x=2$ in to get $y=2^2+3\times 2+5=15$

    • The point $\left(2,15\right)$ lies on the curve

• A gradient function gives the gradient of the curve at a point when you substitute in its x-coordinate

  • A gradient function is written as $\frac{\mathrm{d}y}{\mathrm{d}x}=...$

    • pronounced "dy by dx"

    • This refers to the change in y over change in x

  • For example, the gradient function $\frac{\mathrm{d}y}{\mathrm{d}x}=2x+3$

    • Substitute $x=2$ in to get $\frac{\mathrm{d}y}{\mathrm{d}x}=2\times 2+3=7$

    • The gradient at the point where $x=2$ is 7

• The gradient function $\frac{\mathrm{d}y}{\mathrm{d}x}$ can also be called the derivative or derived function

What is differentiation and how does it work?

• Differentiation is an algebraic method that changes the equation of a curve, $y=...$, into a gradient function, $\frac{\mathrm{d}y}{\mathrm{d}x}=...$

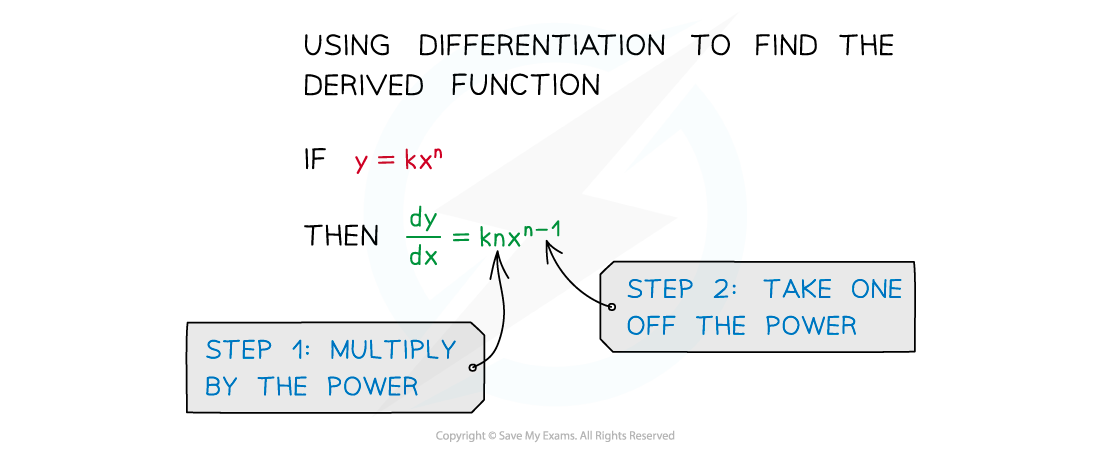
• To differentiate a power of $x$, bring down the power and reduce the power by 1

  • Differentiating $y=x^5$ gives $\frac{\mathrm{d}y}{\mathrm{d}x}=5x^4$

  • Differentiating $y=x^8$ gives $\frac{\mathrm{d}y}{\mathrm{d}x}=8x^7$

  • Differentiating $y=x^n$ gives $\frac{\mathrm{d}y}{\mathrm{d}x}=n{x}^{n-1}$

• Any number that is already in front of $x$ is multiplied by the power that was brought down

  • Differentiating $y=2x^5$ gives $\frac{\mathrm{d}y}{\mathrm{d}x}=2\times 5x^4=10x^4$

  • Differentiating $y=\frac{1}{2}{x}^8$ gives $\frac{\mathrm{d}y}{\mathrm{d}x}=\frac{1}{2}\times 8x^7=4x^7$

  • Differentiating $y=k{x}^n$ gives $\frac{\mathrm{d}y}{\mathrm{d}x}=kn{x}^{n-1}$

• Be careful with two special cases:

  • Differentiating $y=kx$ gives $\frac{\mathrm{d}y}{\mathrm{d}x}=k$

    • The $x$ disappears leaving just a number

    • For example, $y=2x$ differentiates to $\frac{\mathrm{d}y}{\mathrm{d}x}=2$

    • This makes sense, the gradient of the straight line $y=2x$ is 2

  • Differentiating just a number (a constant term), $y=c$, gives $\frac{\mathrm{d}y}{\mathrm{d}x}=0$

    • Numbers disappear!

    • For example, $y=4$ differentiates to $\frac{\mathrm{d}y}{\mathrm{d}x}=0$

    • This makes sense, the gradient of the horizontal line $y=4$ is zero

How do I differentiate sums and differences of terms?

• The equation of a curve may include a number of different terms

  • You can differentiate each term individually

    • Differentiating $y=x^5+x^8$ gives $\frac{\mathrm{d}y}{\mathrm{d}x}=5x^4+8x^7$

    • Differentiating $y=4x^3-10x^6$ gives $\frac{\mathrm{d}y}{\mathrm{d}x}=12x^2-60x^5$

• Remember the two special cases of:

  • $kx$ differentiating to just $k$

  • and constant terms, $c$, differentiating to zero

    • E.g. differentiating $y=8x+1$ gives $\frac{\mathrm{d}y}{\mathrm{d}x}=8$

How do I find the gradient of a curve using the gradient function?

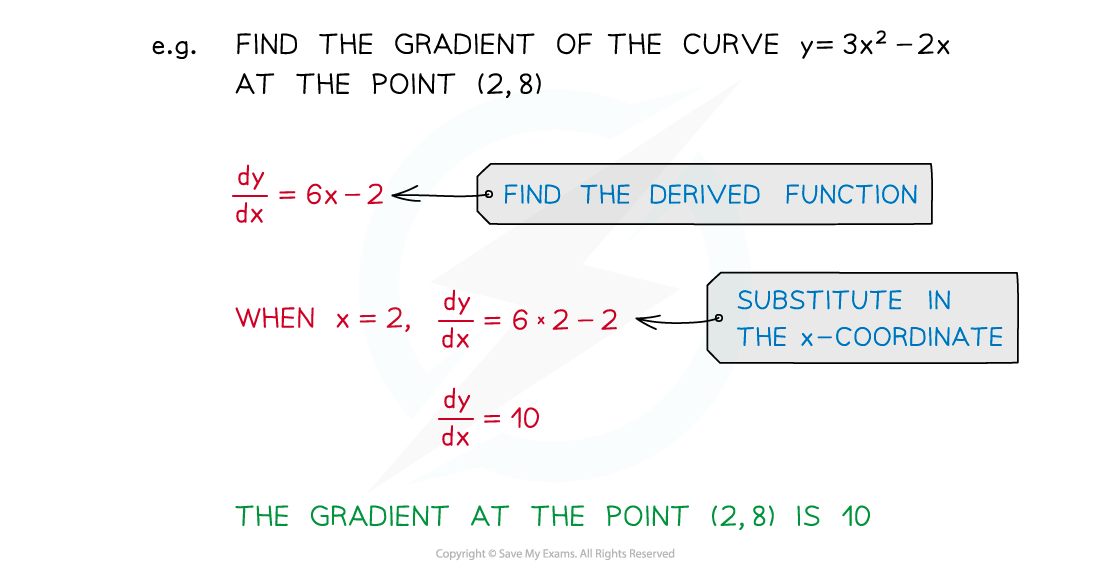
• Find the x-coordinate of the point on the curve you're interested in

• Use differentiation to turn the equation of the curve, $y=...$, into the gradient function, $\frac{\mathrm{d}y}{\mathrm{d}x}=...$

• Substitute the x-coordinate into the gradient function to find the gradient

  • The y-coordinate is not needed

• If instead you are given a gradient and asked to find the x-coordinate

  • set $\frac{\mathrm{d}y}{\mathrm{d}x}$ equal to that gradient and solve the equation

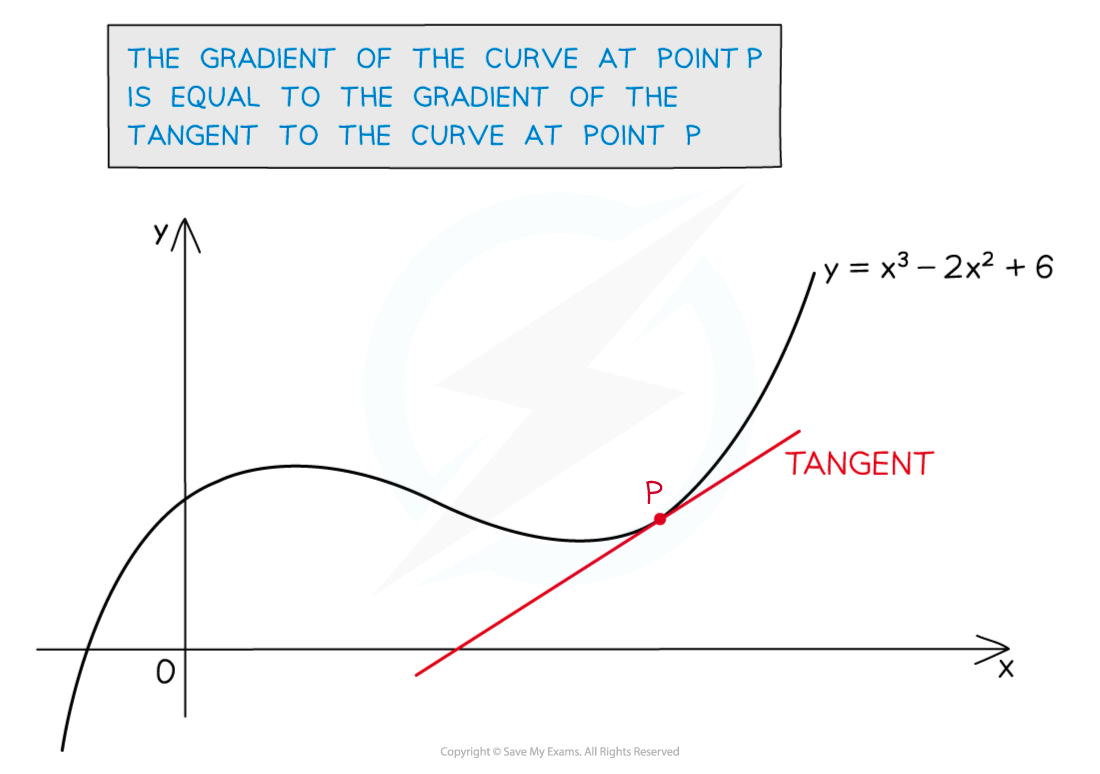
How is the gradient function related to drawing tangents?

• The gradient of a curve changes as you move along the curve

  • To find the gradient at a particular point you can draw a tangent and find its gradient

  • This is a graphical method that is not accurate

    • It depends on how well you draw the tangent

• Instead, you can use differentiation to find the gradient function, then substitute the x-coordinate of the point into the gradient function to find the gradient

  • This is an algebraic method that is exact

• For example, to find the gradient of the curve $y=x^3-2x^2+6$ at the point P where $x=2$

  • either try to draw a tangent at $x=2$ and measure its gradient (see below)

  • or differentiate the equation to get $\frac{\mathrm{d}y}{\mathrm{d}x}=3x^2-4x$

    • Substitute in $x=2$ to get $\frac{\mathrm{d}y}{\mathrm{d}x}=3\times 2^2-4\times 2=4$

    • The gradient is 4