Area & Circumference of Circles | CIE IGCSE Maths: Core Revision Notes 2025

Area & Circumference

What are the properties of a circle?

A circle is a shape that is made up of all the points on a 2D plane that are equidistant from a single point
- Equidistant means the same distance
The circumference of a circle is its perimeter
The diameter, d, of a circle is twice its radius, r
$π$ (pi) is the number (3.14159 …) that is the ratio between a circle’s diameter and its circumference
- You may be asked to give an answer to a question as an 'exact value' or 'in terms of '
  - This topic could appear on the non-calculator paper

What circle formulae do I need to know?

The formulae for the area and circumference of a circle are given to you in your exam
- However, it can be useful to remember them!
The area of a circle can be calculated using the formula:
- $A = π r^{2}$
The circumference of a circle can be calculated using either of the following two formulae:
- $C = π d$
- $C = 2 π r$

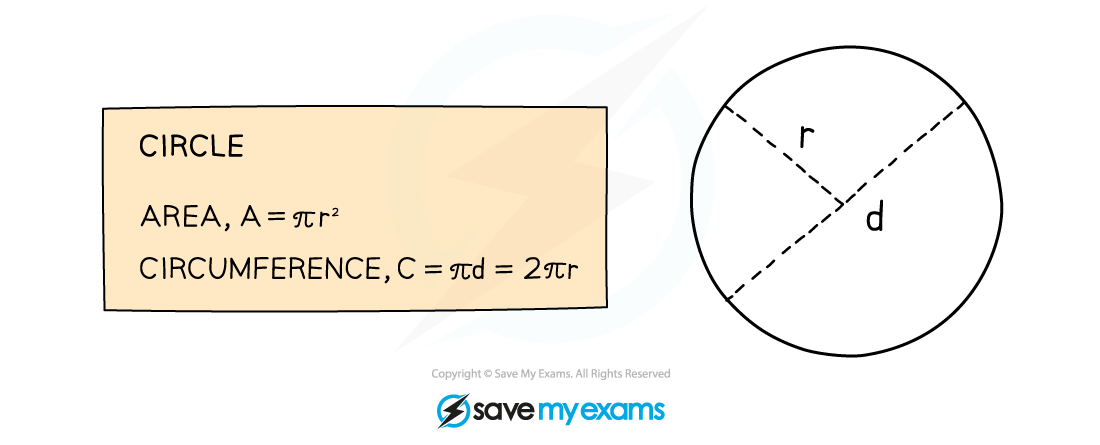
Circumference-Formulae, IGCSE & GCSE Maths revision notes

Working with circle formulae is just like working with any other formula:
- Write down what you know (or what you want to know)
- Pick the correct formula
- Substitute the values in and solve

How do I find the circumference of a circle?

Identify the diameter
- This is double the length of the radius
Multiply the diameter by π

How do I find the area of a circle?

Identify the radius
- This is half the length of the diameter
Square the radius
Multiply the radius squared by π

Examiner Tip

Remember that area is always measured in square units (cm², m², ... etc.) and circumference is always measured in units of a single length (cm, m, ... etc.)

Worked example

Find the area and perimeter of the semicircle shown in the diagram.

Give your answers in terms of $π$ .

Semicircle-d=16, IGCSE & GCSE Maths revision notes

The area of a semicircle is half the area of the full circle with the same diameter, so begin by finding the area of the full circle

Find the radius by dividing the diameter by 2

$r = \frac{16}{2} = 8 cm$

Substitute this into the formula for the area of a circle $A = π r^{2}$ .
Leave your answer in terms of $π$ , (this just means do not multiply by $π$ on your calculator)

$A_{full circle} = π {(8)}^{2} = 64 π$

Find the area of the semicircle by dividing the full area by 2

$A_{semicircle} = \frac{1}{2} (64 π) = \frac{64}{2} π = 32 π$

Area = $32 π$ cm²

The perimeter of the semicircle is made up of both the arc of the circle (half of the circumference) and the diameter of the semicircle

Find the full length of the circumference of the circle using the formula $C = 2 π r$ (or $C = π d$ )
Substitute the radius = 8 cm into the formula
Again, leave your answer in terms of $π$

$C = 2 π (8) = 16 π$

Find the length of the arc (the curved part of the perimeter of the semicircle) by dividing the full area by 2

$C u r v e d l e n g t h = \frac{1}{2} (16 π) = \frac{16}{2} π = 8 π$

Find the full perimeter by adding this to the length of the diameter of the circle

Perimeter = $8 π + 16$ cm

Area & Circumference of Circles (CIE IGCSE Maths: Core)

Revision Note