Syllabus Edition

First teaching 2021

Last exams 2024

Set Notation & Venn Diagrams (CIE IGCSE Maths: Core)

Revision Note

Test yourself

Author

Jamie W

Last updated

4 March 2023

Set Notation

What is a set?

A set is a collection of elements
- Elements could be anything - numbers, letters, coordinates etc
You could describe a set by writing its elements inside curly brackets {}
- e.g. {1, 2, 3, 6} is the set of the factors of 6
If the set of elements follow a rule then you can write this using a colon inside the curly brackets {... : ...}
- The bit before the colon is just the type of element
- The bit after the colon is the rule
- e.g. {x : x² < 100} is the set of numbers which are less than 100 when squared

What do I need to know about set notation?

$E$ is the universal set (the set of everything)
- e.g. if talking about factors of 24 then $E$ = {1, 2, 3, 4, 6, 8, 12, 24}
- You may see alternative notations used for $E$
  - U is a common alternative
  - S or the Greek letter ξ (xi) may also be seen
We normally use upper case letters to represent sets (A, B, C, ...) and lower case letters to represent elements (a, b, c, ...)
n(A) is the number of elements in set A
- e.g. n({1, 4, 9}) = 3
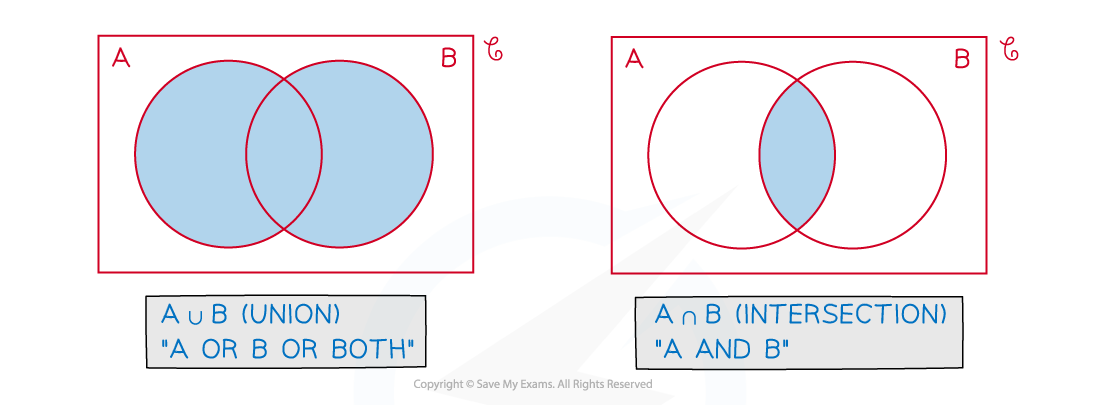
A ∩ B means the intersection of A and B (the overlap of A and B)
- This is the set of elements that are in both set A and set B
A ∪ B means the union of A and B (everything in A or B or both)
- This is the set of elements that are in at least one of sets

Sets & Venn Diagrams

What is a Venn diagram?

A Venn diagram is a way to illustrate all the elements within sets and any intersections
A Venn diagram consists of
- a rectangle representing the universal set ( $E$ )
- a circle for each set
  - Circles may or may not overlap depending on which elements are shared between sets

What do the different regions mean on a Venn diagram?

$A \cap B$ is represented by the region where the A and B circles overlap
$A \cup B$ is represented by the regions that are in A or B or both

3-2-1-fig1-venn-and-set-notation-core

Worked example

Two sets A and B are shown in the Venn diagram.

Venn-Set-1, downloadable IGCSE & GCSE Maths revision notes

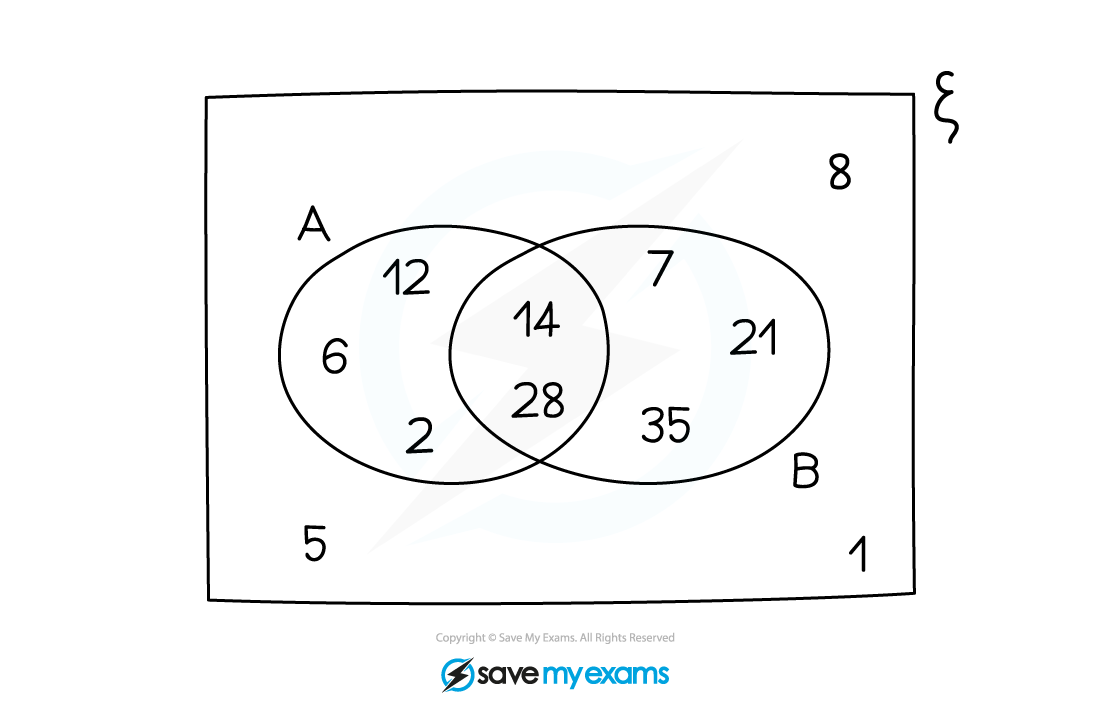
(a)

Write down n(A).

The elements of A are anything that is inside the A circle. A = {2, 6, 12, 14, 28}. There are 5 elements in it.

n(A) = 5

(b)

Use set notation to complete the sentence {14, 28} = ...

14 and 28 are the elements that are in both A and B.

{14, 28} = A∩B

(c)

Write down the elements that are in set A∪B.

A is the set of elements in A so A = {2, 6, 12, 14, 28}
B = {7, 14, 21, 28, 35}.
A∪B is the set of elements that are in at least one of the sets

A∪B = {2, 6, 7, 12, 14, 21, 28, 35}

You've read 0 of your 5 free revision notes this week

Sign up now. It’s free!

Join the 100,000+ Students that ❤️ Save My Exams

the (exam) results speak for themselves: