Properties of Lines & Angles
What properties do I need to know about lines and line segments?
- A line is a straight, one dimensional path that extends forever
- A line segment is a part of a line, it has a start and an end point
- A line segment that starts at the point A and ends at the point B is usually labelled AB
- Dashes are used to identify equal length line segments in a diagram
- If there is more than one pair (or set) of equal length lines in a diagram then multiple dashes will be used
- Two lines are parallel if they continue in the same direction forever
- Parallel lines will never intersect (meet)
- Parallel lines should be marked with arrows
- If there is more than one pair (or set) of parallel lines in a diagram then multiple arrows will be used
- If there is more than one pair (or set) of parallel lines in a diagram then multiple arrows will be used
- Two lines are perpendicular if they intersect at right-angles (90°)
What properties do I need to know about angles?
- When two lines intersect (meet) they will form an angle
- There are different types of angles
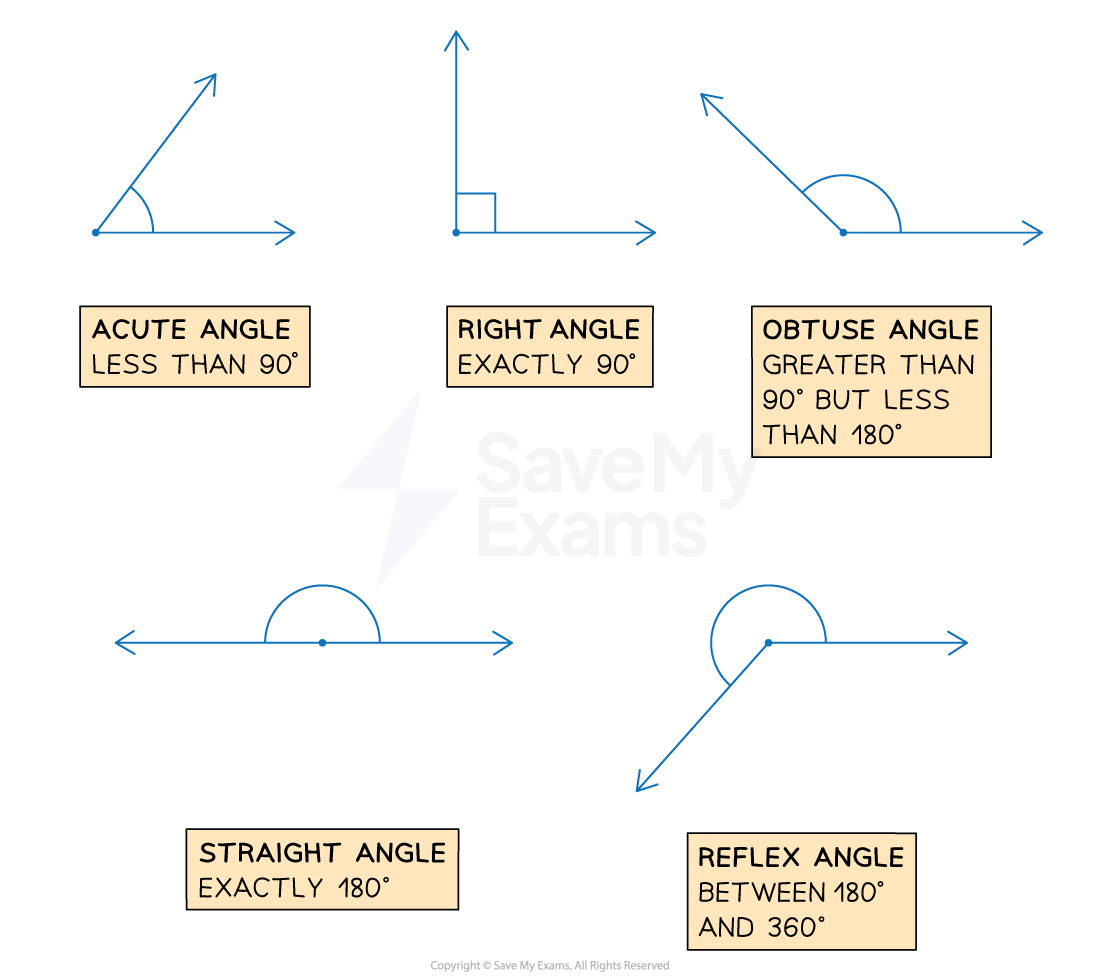
- An angle that is less than 90° is called an acute angle
- An angle that is exactly 90° is called a right angle
- An angle that is greater than 90° and less than 180° is called an obtuse angle
- An angle that is exactly 180° is called a straight angle
- An angle that is greater than 180° but less than 360° is called a reflex angle
- Angles at a point add up to 360°
- Angles on a straight line add up to 180°
- Angles that add up to 180° are called supplementary angles
Examiner Tip
- You must know the correct names of the angles as you are likely to be asked questions about them in the exam
- Do not assume two lines are parallel or perpendicular just because they look it, always look out for the arrows or right angle in a diagram, or read the question to look for clues
- Knowing which shapes have parallel and perpendicular lines will help you to recognise where they might be
Worked example
Write down the mathematical name for an angle which is greater than 90° but less than 180°.
An obtuse angle
