Problem Solving with Differentiation (Edexcel IGCSE Maths A) : Revision Note
Did this video help you?
Problem Solving with Differentiation
What is problem solving using differentiation?
You can use the same method of differentiating curves to find turning points to help with problems involving finding the maximum or minimum value of a quantity
These are called optimisation problems
Questions may use different variables
For example,
and
How do I apply differentiation to different contexts?
This is easiest explained using an example
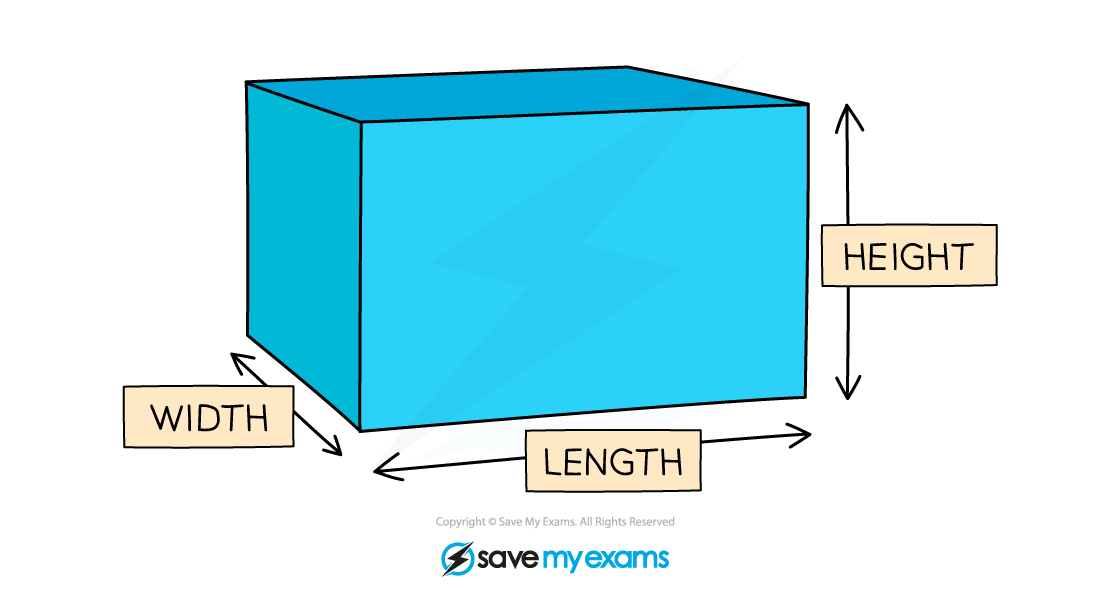
Find the maximum volume,
m3, of the cuboid shown with width 2 m, length
m and height
m
Find a formula for its volume in terms of
Volume = width
length
height
Expand this into individual terms in
This is a negative quadratic in
(it has an
shape)
The graph of
against
will have a maximum point when
Find
by differentiating each term
Set this equal to zero and solve
so
giving
This is the value of
at the maximum point (not the value of
)
Substitute this value of
back into the equation for
The maximum volume is 50 m3
Examiner Tips and Tricks
A common problem in the exam is to forget to substitute the value of back into the formula!
How do I know if I have found a maximum value or a minimum value?
Sometimes there are two values of
for different turning points and you need one of them
either substitute them both back into the formula
See which gives the max value and which gives the min value
or use any acceptable techniques for classifying turning points
For example, using a sketch to see if its a max or min
Remember that
Positive quadratics have minimum points
Negative quadratics have maximum points
How do I use differentiation if there are lots of variables?
Sometimes a formula has lots of letters
You need to find an extra relationship between these letters
then substitute it into the formula
If, in the above example, you had width 2 m, length
m and height
m then
But you need a formula in
only
You will be given an extra piece of information, such as the length and height sum to 10 m
Therefore
Make
the subject,
Substitute it into
to get
Worked Example
A farmer has 60 metres of fencing and wants to fence off the biggest rectangular area possible next to an existing wall.
The area has dimensions metres by
metres, as shown.
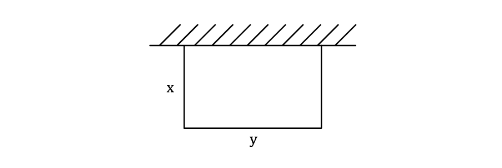
(a) Explain why .
The wall is not part of the fencing
Find the total length of the three sides of the fence shown
This must equal 60 metres
The length of the fence must equal 60 metres so
(b) Show that the area, m2, is given by
.
Find the area of the rectangle shown
You need a right-hand side in terms of only
Make the subject of part (a)
Substitute this into the area formula
This is now all in terms of
Expand
(c) Find the maximum possible area.
To find a maximum point, set
First find by differentiating each term
Set this equal to zero and solve
This is the value of at the maximum point (but not the maximum of
)
Substitute this value of back into
The maximum area is 450 m2
(d) Explain how you know that the answer in part (c) is a maximum area, not a minimum area.
See how the area depends on
Think about what it would look like as a graph
is a negative quadratic curve
A negative quadratic curve has an shape
There is only one turning point on this graph and it is a maximum point
The curve is a negative quadratic curve
This means it can only have a maximum point, not a minimum point

You've read 0 of your 5 free revision notes this week
Sign up now. It’s free!
Did this page help you?

