Enlargements (Edexcel IGCSE Maths A): Revision Note
Exam code: 4MA1
Did this video help you?
Enlargements
What is an enlargement?
An enlargement changes the size and position of a shape
The length of each side of the shape is multiplied by a scale factor
If the scale factor is greater than 1 then the enlarged image will be bigger than the original object
If the scale factor is between 0 and 1 (fractional) then the enlarged image will be smaller than the original object
The centre of enlargement determines the position of the enlarged image
If the scale factor is greater than 1 then the enlarged image will be further away from the centre of enlargement
If the scale factor is between 0 and 1 then the enlarged image will be closer to the centre of enlargement
How do I enlarge a shape?
STEP 1
Pick a vertex of the shape and count the horizontal and vertical distances from the centre of enlargement
STEP 2
Multiply both the horizontal and vertical distances by the given scale factorSTEP 3
Start at the centre of enlargement and measure the new distances to find the enlarged vertexSTEP 4
Repeat the steps for the other verticesYou might be able to draw the enlarged shape from the first vertex by multiplying the original lengths by the scale factor
This can be done quickly if the shape is made up of vertical and horizontal lines
STEP 5
Connect the vertices on the enlarged image and label it
How do I describe an enlargement?
To describe an enlargement, you must:
State that the transformation is an enlargement
State the scale factor
This may be an integer or a fraction
Give the coordinates of the centre of enlargement
To find the scale factor:
Pick a side of the original shape
Identify the corresponding side on the enlarged image
For a fractional enlargement, the side on the enlarged image will be smaller than the corresponding side on the original image
Divide the length of the enlarged side by the length of the original side
To find the centre of enlargement:
Pick a vertex of the original shape
Identify the corresponding vertex on the enlarged image
Draw a line going through these two vertices
Repeat this for the other vertices of the original shape
These lines will intersect at the centre of enlargement
How do I reverse an enlargement?
If a shape has been enlarged, you can perform a single transformation to return the shape to its original size and position
An enlargement can be reversed by multiplying the enlarged shape by the reciprocal of the original scale factor
The centre of enlargement is the same
For a shape enlarged by a scale factor of 3 with centre of enlargement (-1, 6)
The reverse transformation is
an enlargement of scale factor
with centre of enlargement (-1, 6)
Examiner Tips and Tricks
To check that you have enlarged a shape correctly:
Draw lines going from the centre of enlargement to each of the vertices of the original shape
Extend these lines
The lines should go through the corresponding vertices of the enlarged image
Worked Example
(a) On the grid below enlarge shape C using scale factor 2 and centre of enlargement (2, 1).
Label your enlarged shape C'.
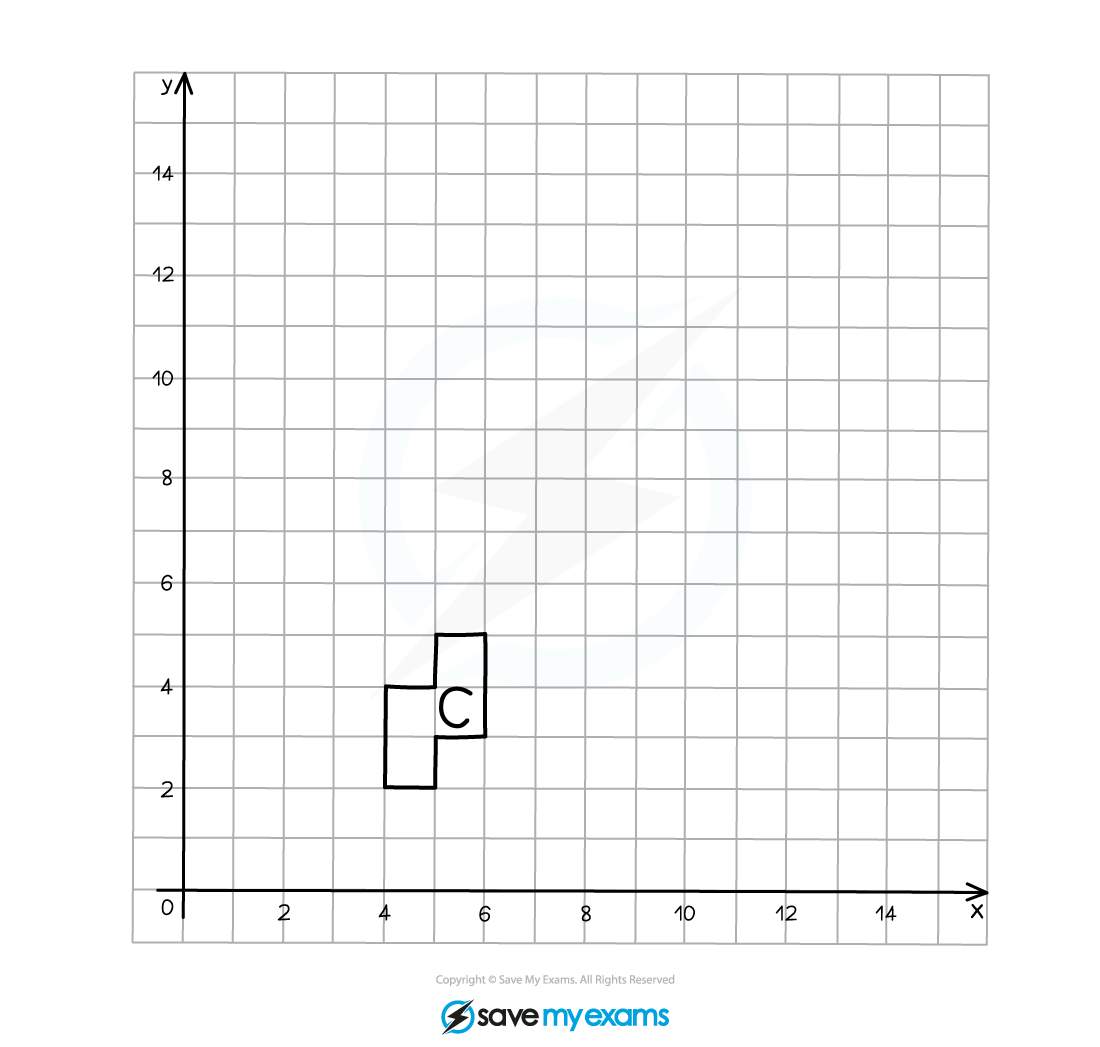
Start by marking on the centre of enlargement (CoE)
Count the number of squares in both a horizontal and vertical direction to go from the CoE to one of the vertices on the original object, this is 2 to the right and 3 up in this example
As the scale factor is 2, multiply these distances by 2, so they become 4 to the right and 6 up
Count these new distances from the CoE to the corresponding point on the enlarged image and mark it on
Draw a line through the CoE and the pair of corresponding points, they should line up in a straight line
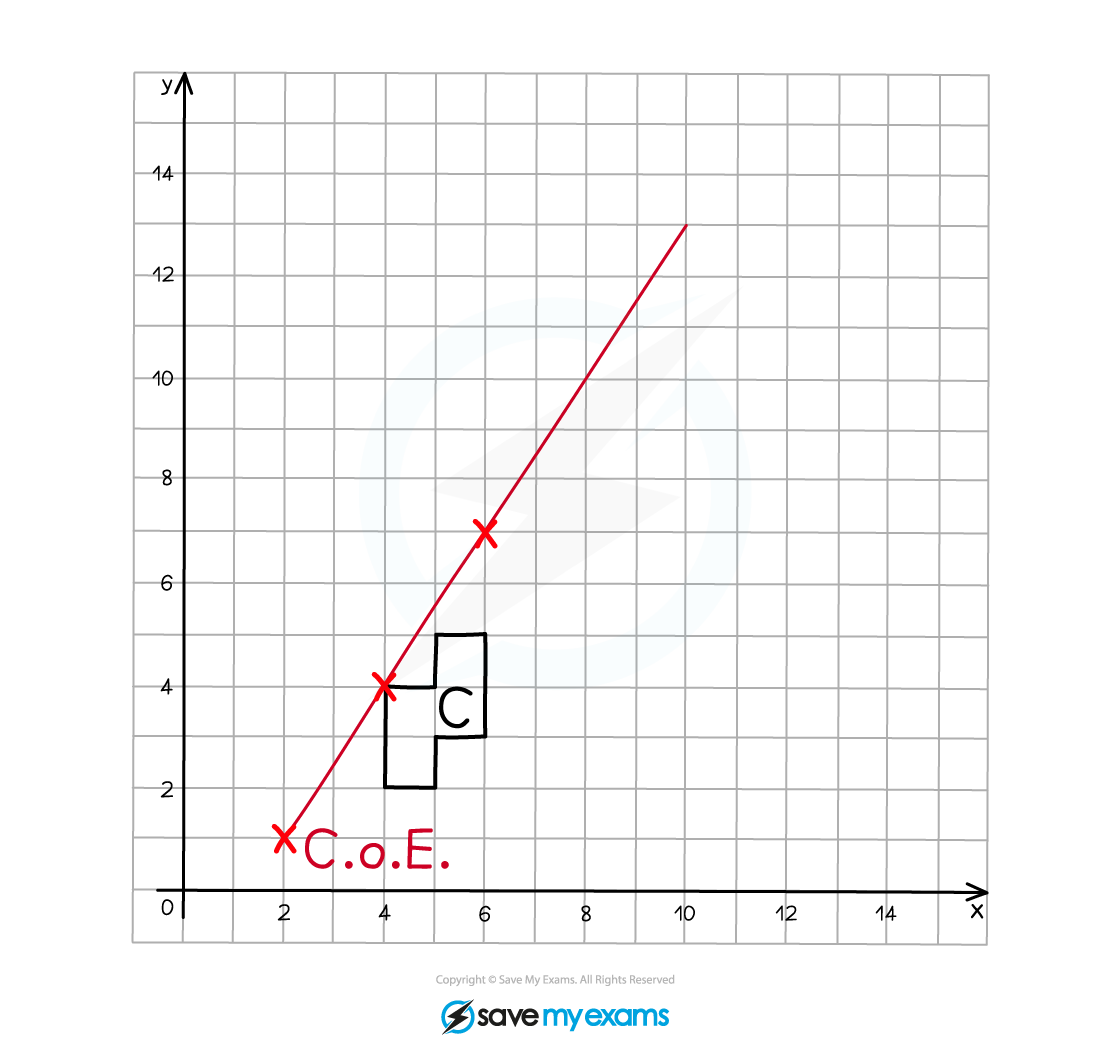
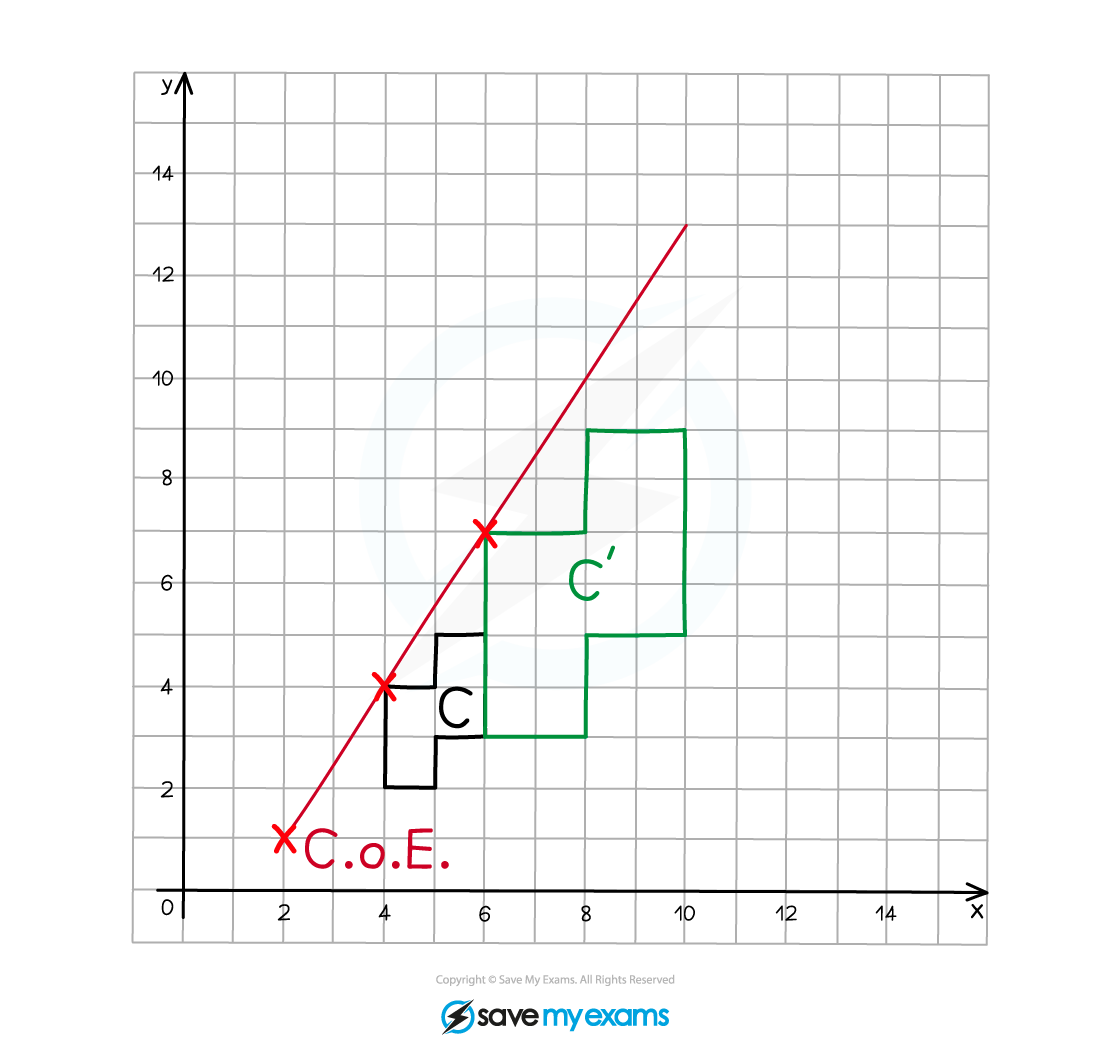
Repeat this process for each of the vertices on the original object (or at least 2)
Join adjacent vertices on the enlarged image as you go
Label the enlarged image C'
(b) Describe fully the single transformation that creates shape B from shape A.
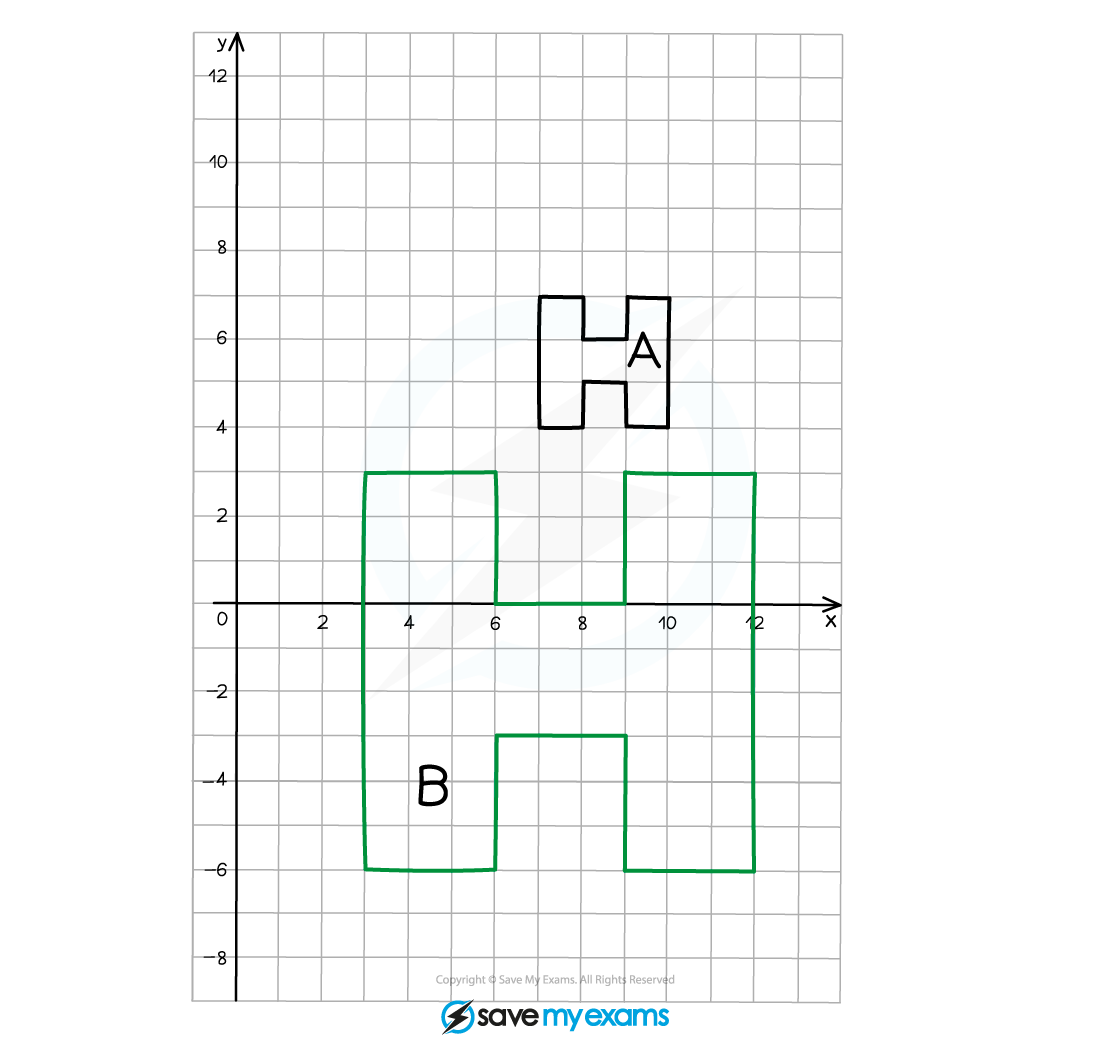
We can see that the image is larger than the original object, therefore it must be an enlargement
As the enlarged image is bigger than the original object, the scale factor must be greater than 1
Compare two corresponding edges on the object and the image to find the scale factor
The height of the original "H" is 3 squares
The height of the enlarged "H" is 9 squares
Draw a straight line through the CoE and a pair of corresponding points on the original object and the enlarged image
Repeat this step for as many vertices as you feel you need to so you can confidently locate the CoE
Do this for all pairs of vertices to be sure!
The point of intersection of the lines is the CoE
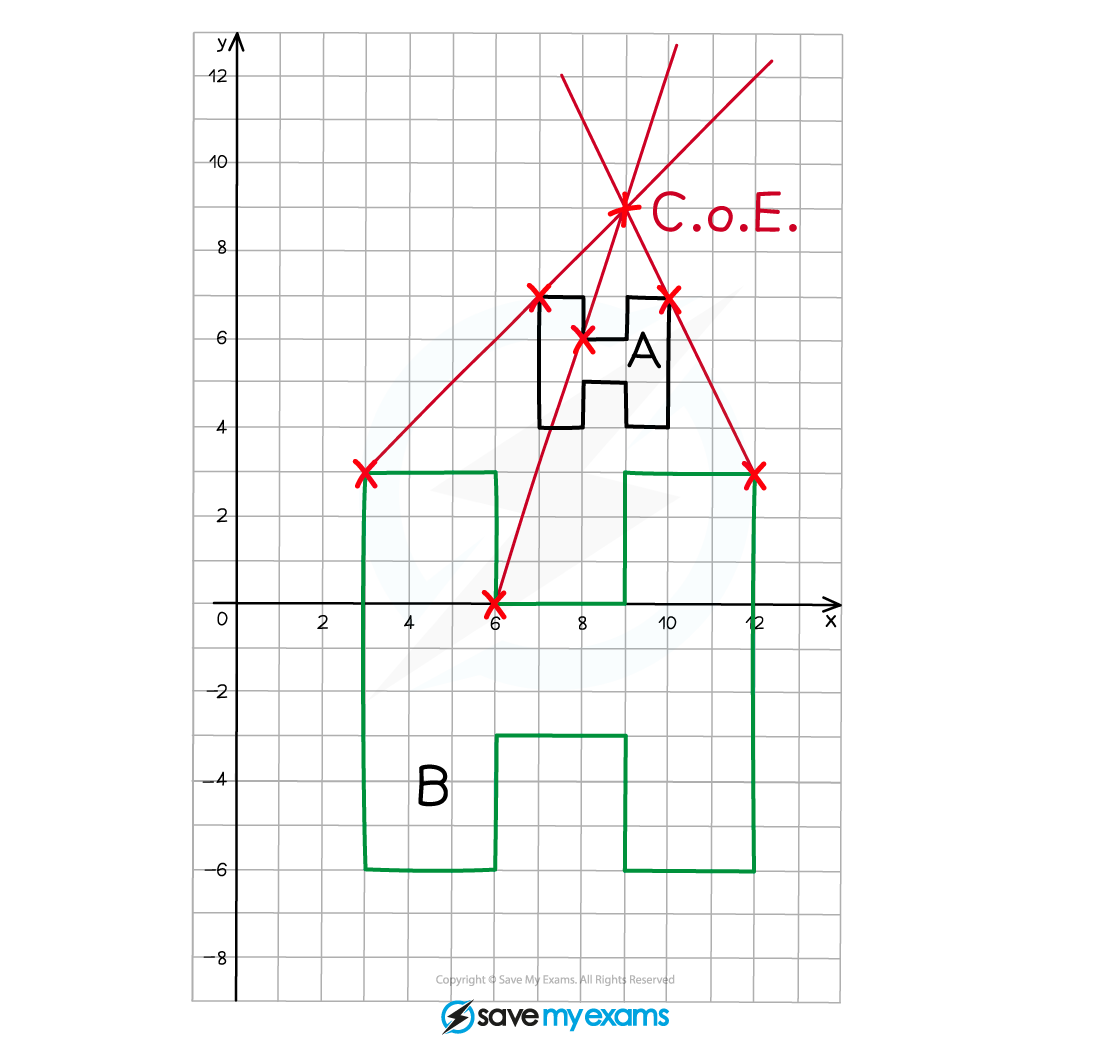
Shape A has been enlarged using a scale factor of 3 and a centre of enlargement (9, 9) to create shape B
Worked Example
(a) On the grid below enlarge shape C using scale factor and centre of enlargement (4, 2).
Write down the four vertices of your enlarged shape.
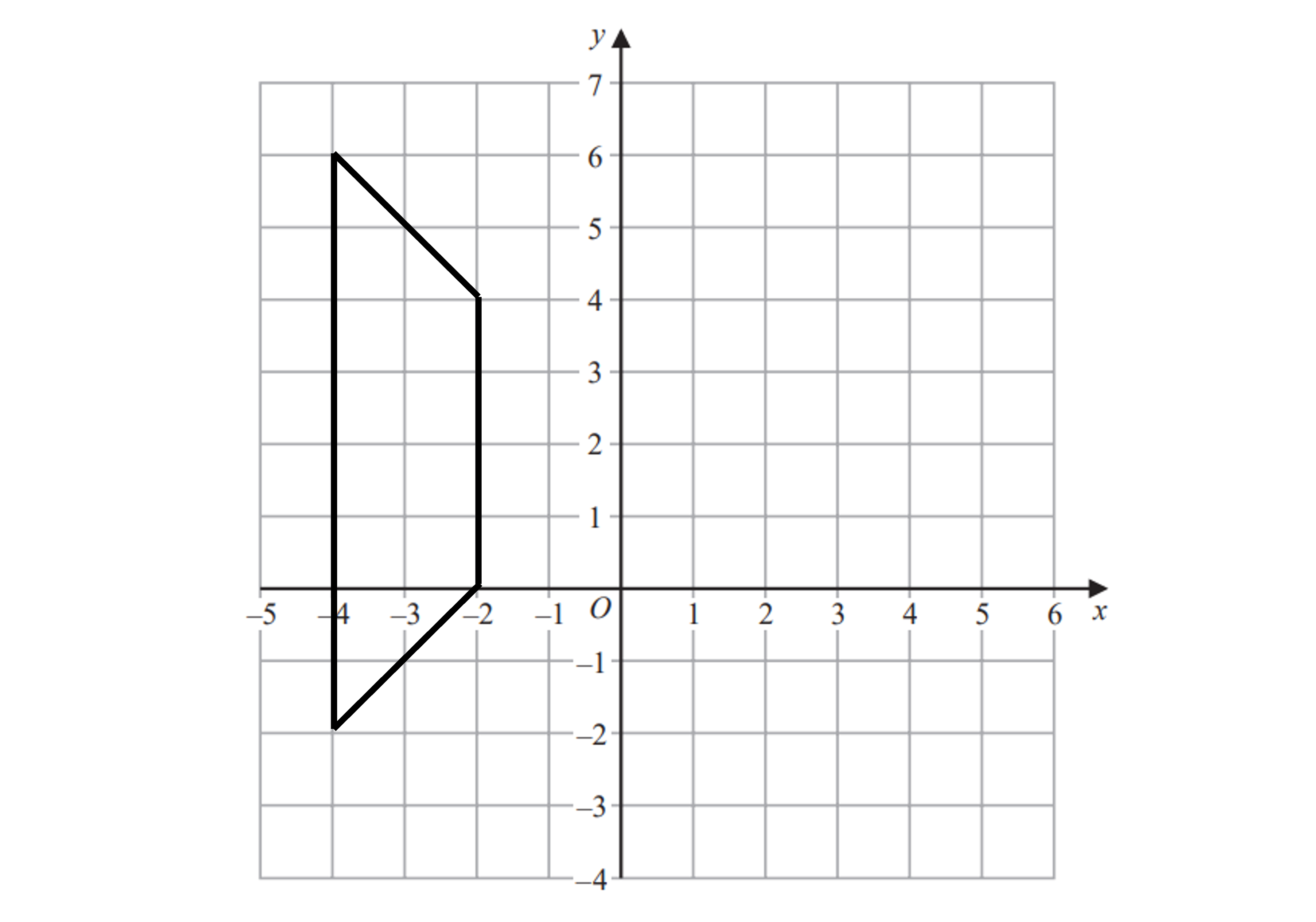
Mark the centre of enlargement at (4, 2)
Count the number of squares horizontally and vertically to any vertex - we've chosen the vertex at (-2, 4)
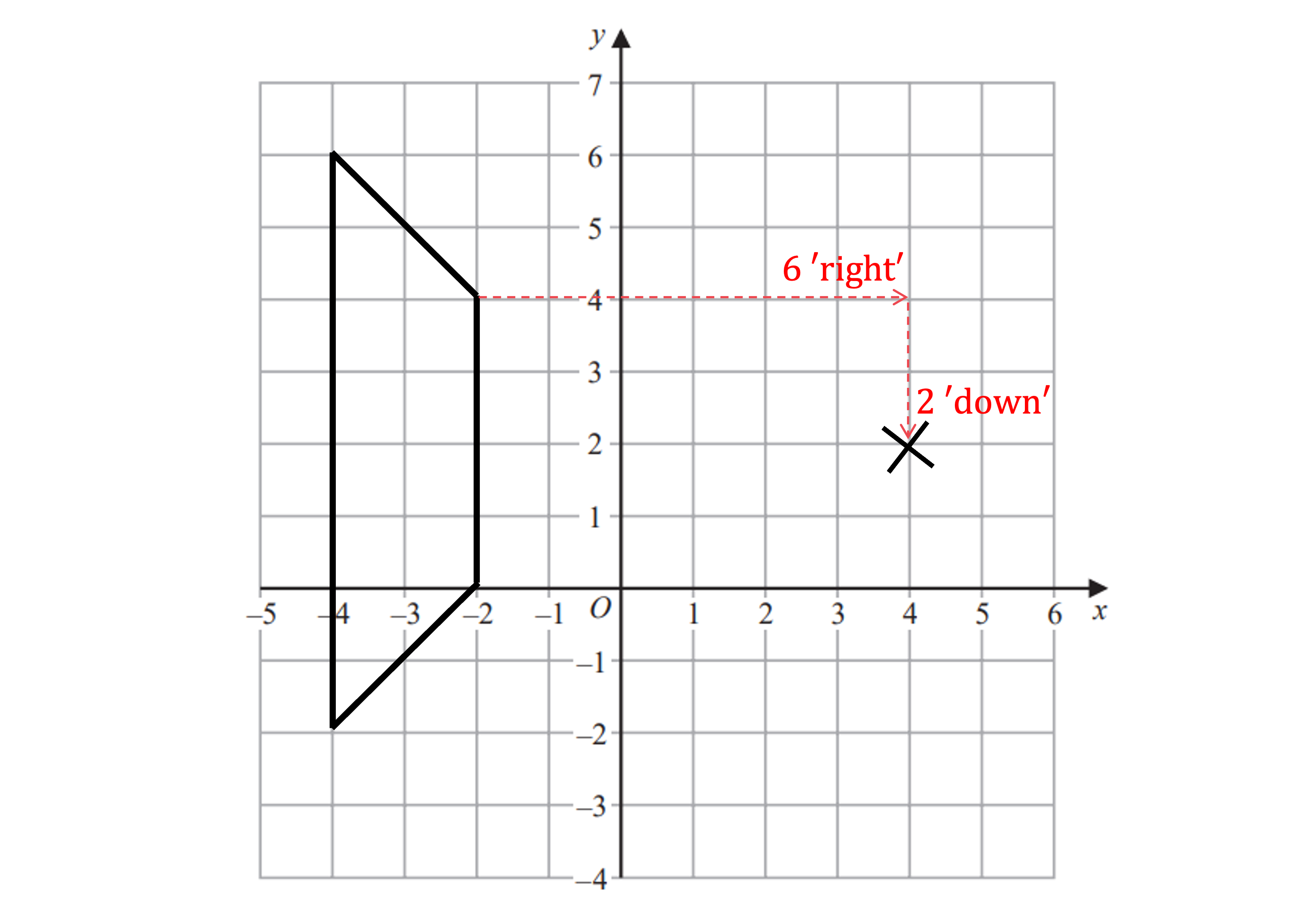
Multiply these distance by the scale factor,
6 'right 'right'
2 'down' 'down'
Count these new distances (which should be smaller than the originals) from the CoE to find the corresponding point on the new image and mark it on
Repeat as required and draw lines through corresponding vertices and the CoE as a check
Use a logical order, working your way round the shape slowly, to ensure you do miss any vertices out
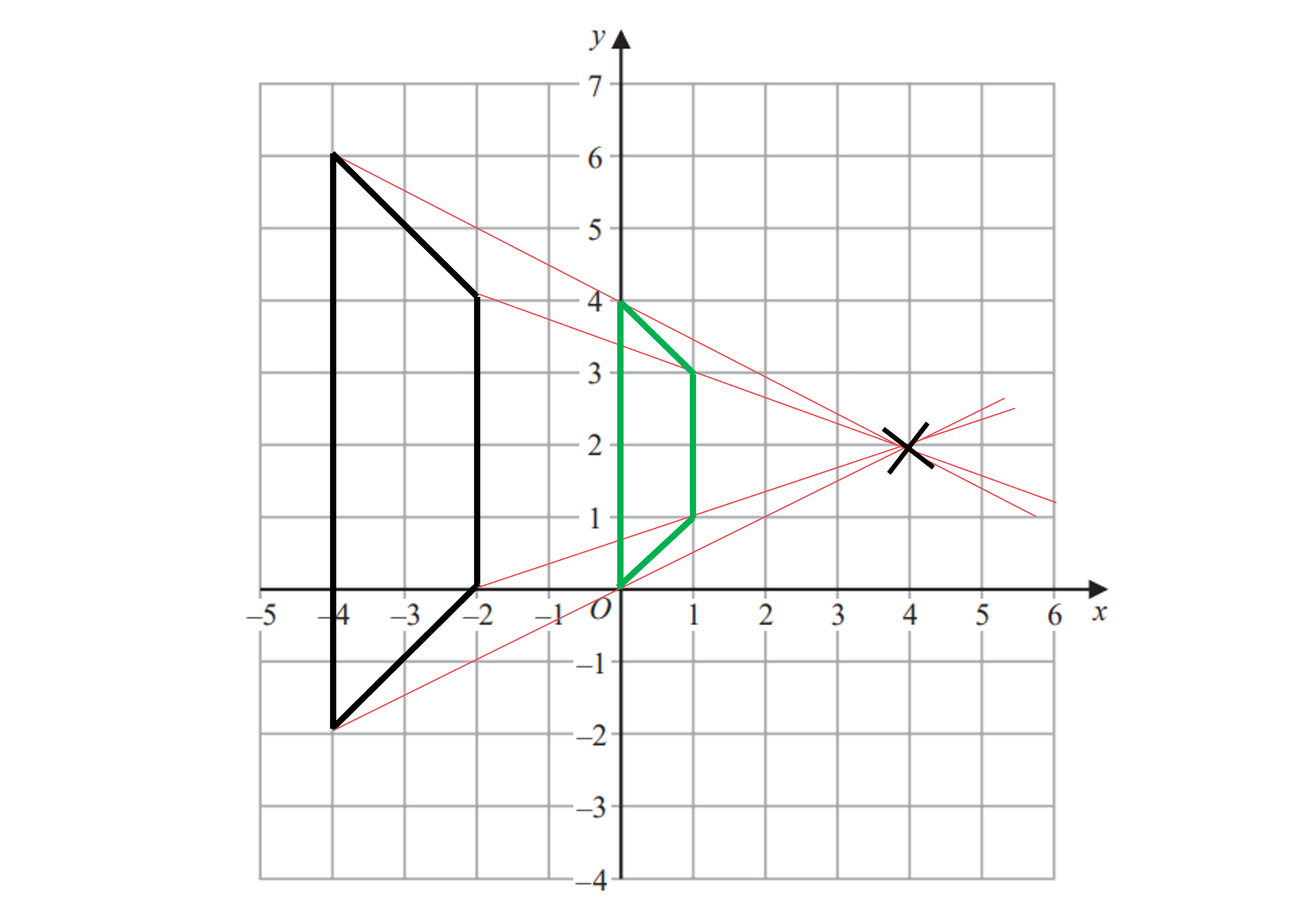
The four vertices of the enlarged shape are (0, 0), (0, 4), (1, 3) and (1, 1)
(b) Describe fully the single transformation that creates shape B from shape A.
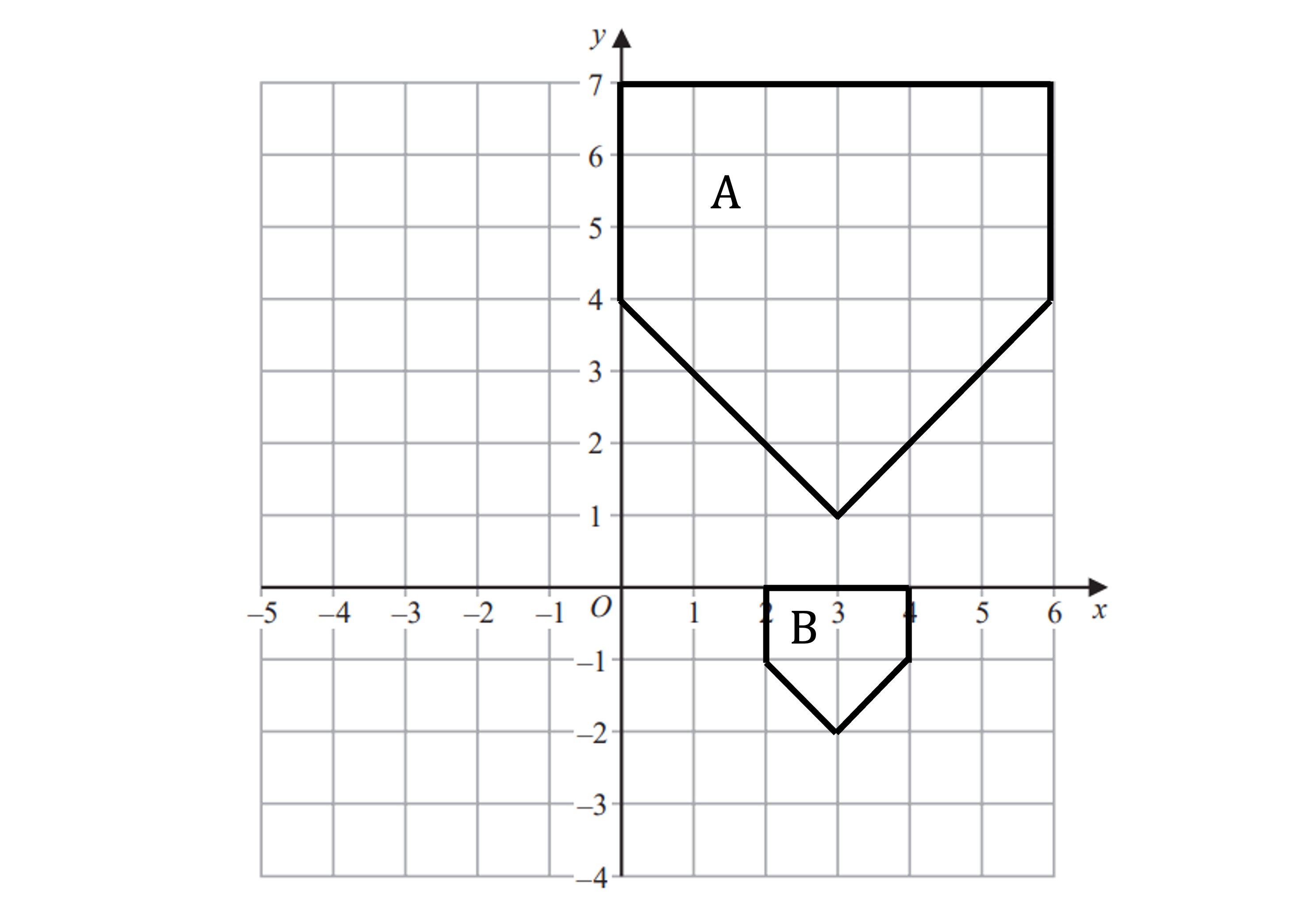
We can see the image is smaller than the original so it is a fractional enlargement
Compare two corresponding edges to find the scale factor - we've used the top edge
scale factor =
Draw straight lines through corresponding vertices on the original shape
Repeat this 3-4 times and you should find the lines intersect at the same point
This point will be the CoE
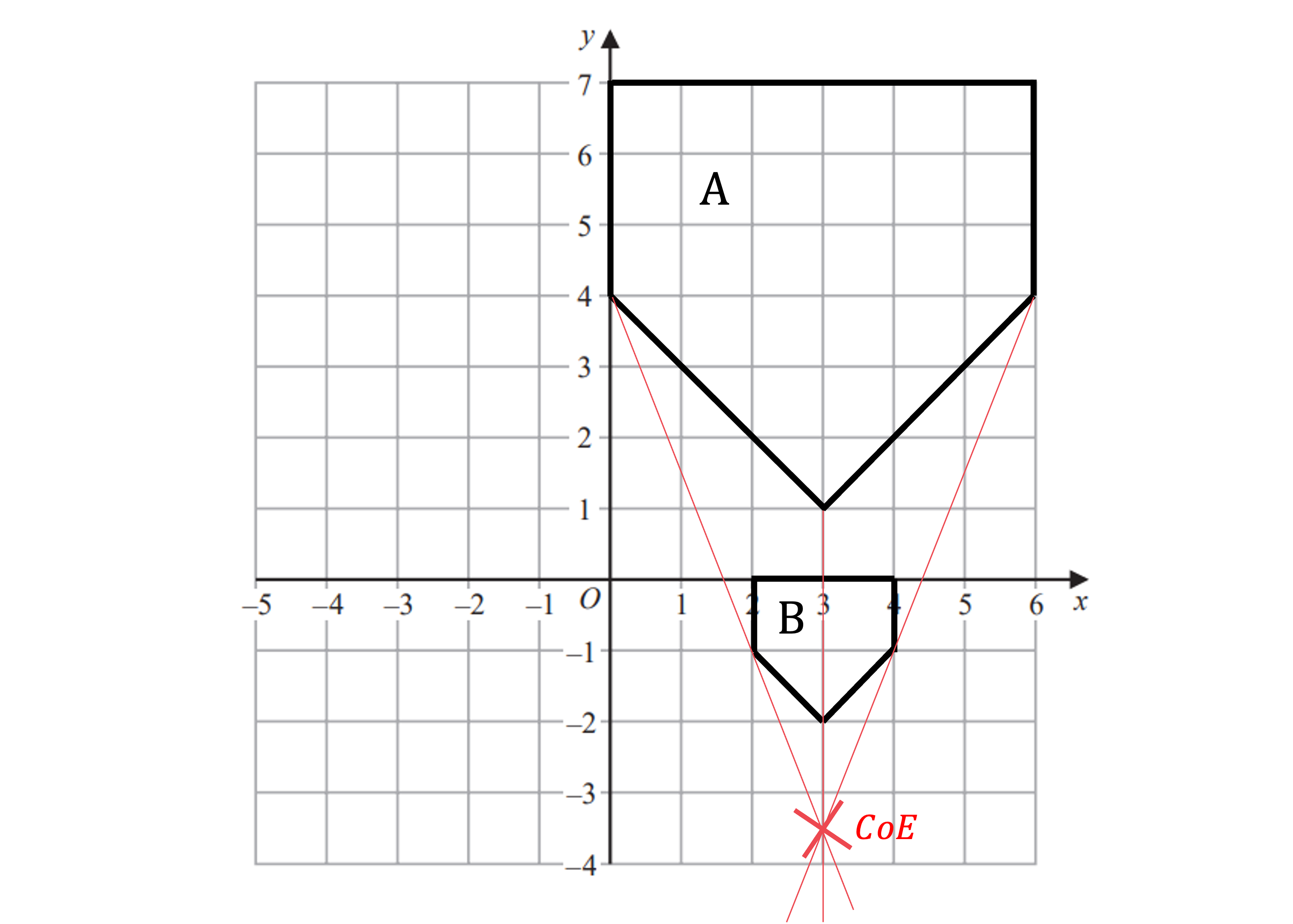
Shape A has been enlarged using a scale factor of and a centre of enlargement (3, -3.5) to create shape B

Unlock more, it's free!
Did this page help you?