3D Shapes (Edexcel IGCSE Maths A)
Revision Note
Properties of 3D Shapes
What common 3D shapes do I need to know about?
There are a number of common 3D shapes
You should know their names
and their key properties
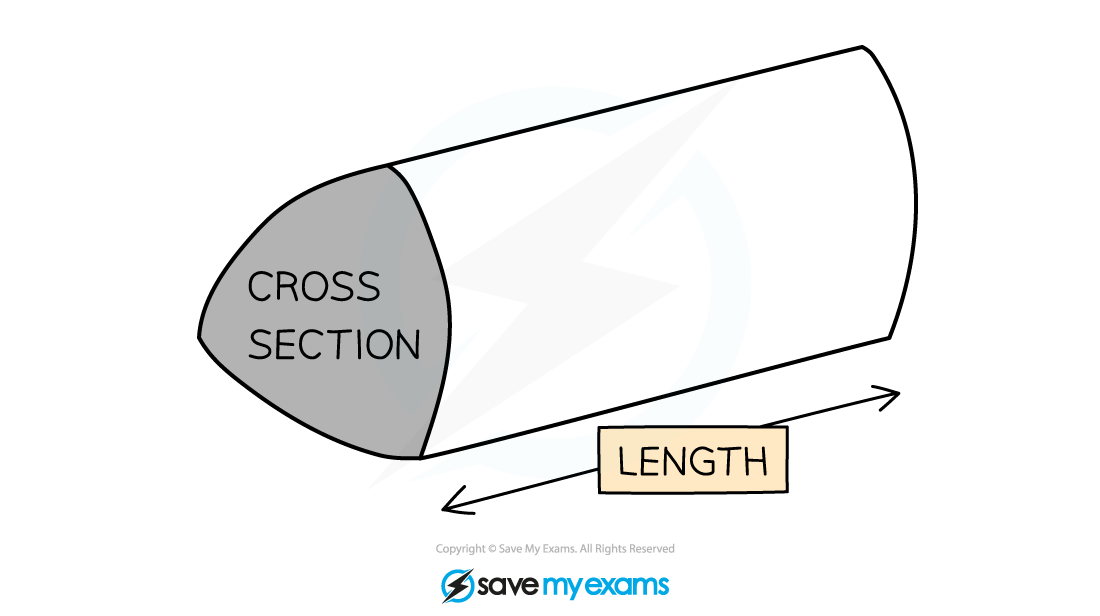
A prism is a 3D shape with the same cross-section throughout
The cross-section of a cube is a square
The cross-section of a cuboid is a rectangle
There are other prisms, such as triangular prisms or hexagonal prisms
In these cases the exam question will make sure the shape of the cross-section is clear
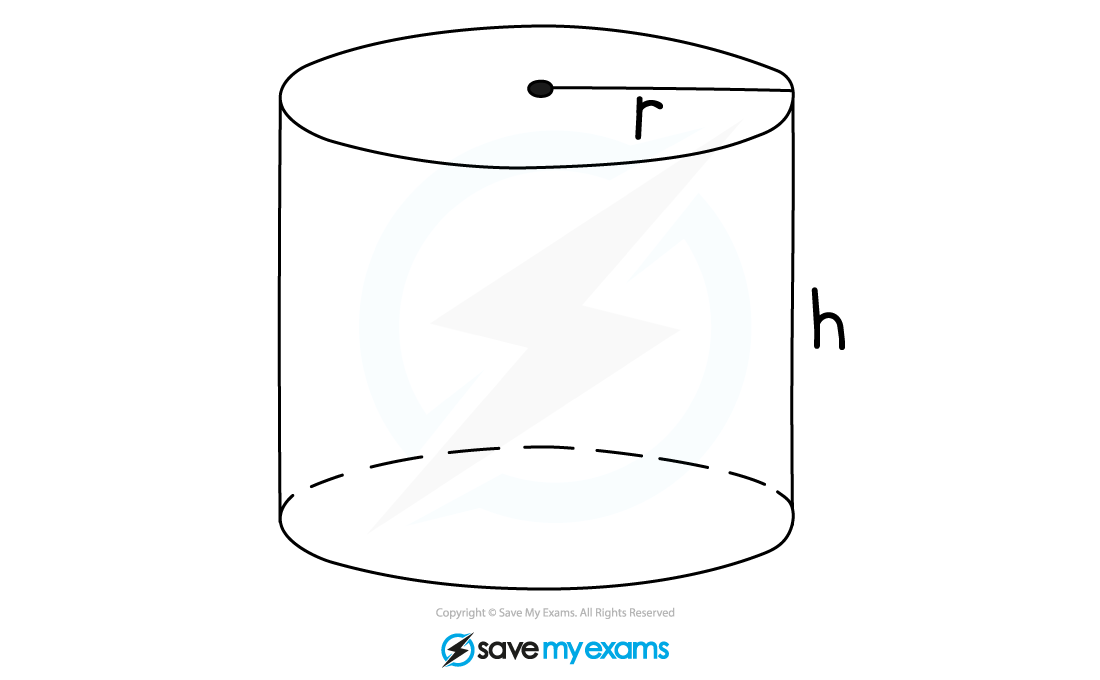
A cylinder is similar to a prism
The cross-section of a cylinder is a circle
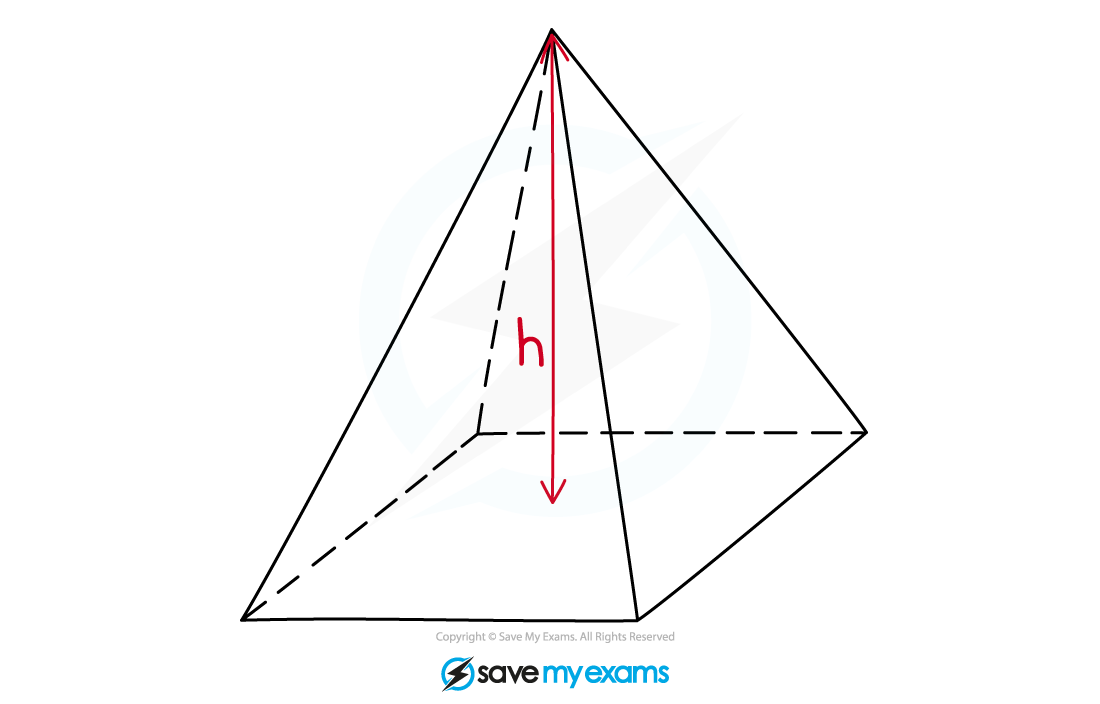
There are a number of different types of pyramid
A pyramid has a flat base and sloping sides that meet at a point
The pyramid may have a square, rectangular or triangular base
Some pyramids have special names you should know
A triangular-based pyramid is called a tetrahedron
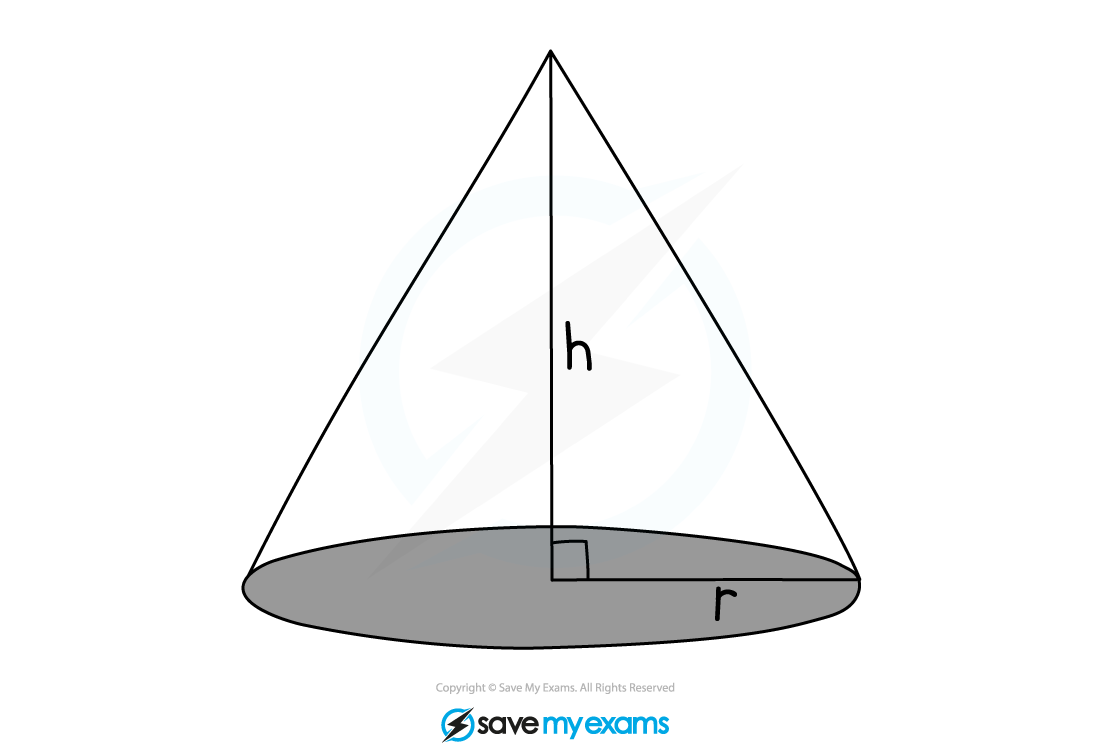
A cone is similar to a pyramid
A cone has a circular base
A sphere is a round solid ball shape
What are the properties of 3D shapes?
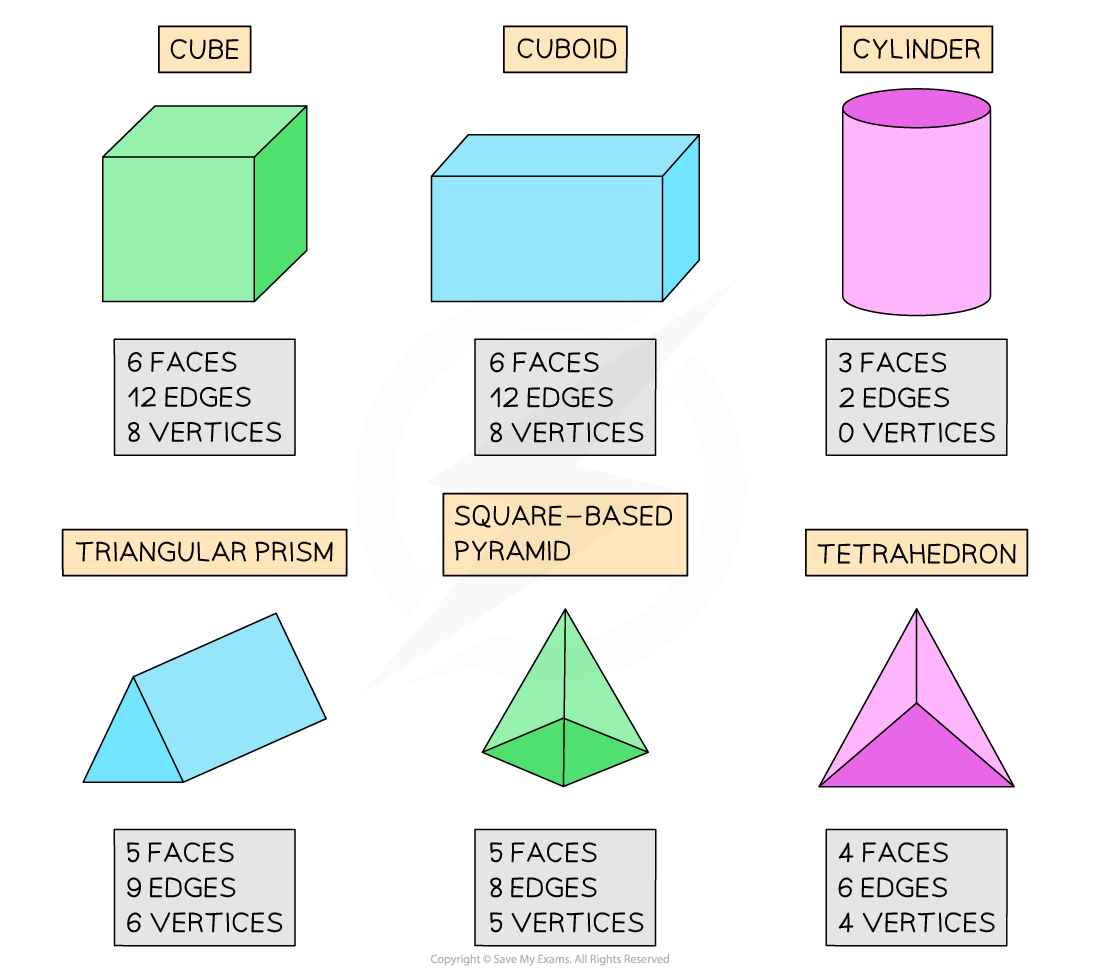
3D shapes have a number of faces, vertices and edges
A face is a single surface of the 3D shape
A vertex (plural, vertices) is a corner of the 3D shape
An edge joins one vertex to another
You should know the number and shape of the faces for the common 3D shapes
A cube has 6 equal, square faces
A cuboid has 3 pairs of rectangular faces
A cylinder has 2 equal circular faces and 1 rectangular face (its curved surface)
A triangular prism has 2 equal triangular faces and 3 rectangular faces
If the triangular faces are equilateral then all of the rectangles will be equal
If the triangular faces are isosceles then two of the rectangles will be equal
A square-based pyramid has 1 square face and 4 equal triangular faces
A tetrahedron has 4 triangular faces
A sphere has 1 face; it is a ball-shape
Examiner Tips and Tricks
Remembering the properties of 3D shapes will help in particular with questions involving surface area

You've read 0 of your 5 free revision notes this week
Sign up now. It’s free!
Did this page help you?

