Magnitude of a Vector (Cambridge (CIE) IGCSE International Maths)

Revision Note

Written by: Jamie Wood

Reviewed by: Dan Finlay

Did this video help you?

Magnitude of a Vector

How do I find the magnitude of a vector?

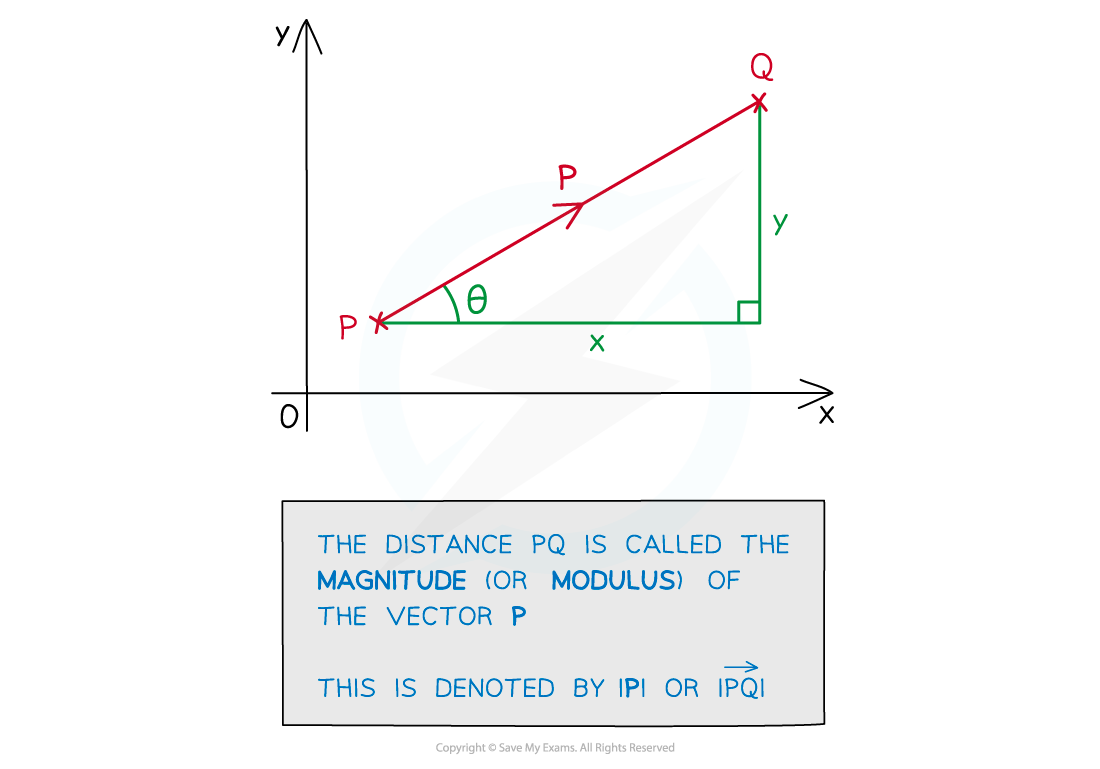
The magnitude of a vector is its length (distance)
- It is also called the modulus
- This is always a positive value
- The direction of the vector is irrelevant
The magnitude of $\vec{A B}$ is written $|\vec{A B}|$
- The magnitude of a is written |a|

Depending on the use of the vector, the magnitude of a vector represents different quantities
- For velocity, magnitude would be speed
- For a force, magnitude would be the strength of the force (in Newtons)

In component form, the magnitude is the hypotenuse of a right-angled triangle
- Use Pythagoras' theorem to find the magnitude
- The magnitude of $a = (\begin{matrix} x \\ y \end{matrix})$
  - $|a| = \sqrt{x^{2} + y^{2}}$

Diagram showing a vector P with horizontal component x and vertical component y. The magnitude of the vector is |P|.

Examiner Tips and Tricks

If there is no diagram, sketch one!
- You can sketch a vector and use it to form a right-angled triangle

Worked Example

Consider two points $A (- 3, 5)$ and $B (7, 1)$ .

(a) Write down the column vector $\vec{A B}$ .

Find the horizontal and vertical distances between the two points
Subtract the x and y components of A from B

$\vec{A B} = (\begin{matrix} 7 - - 3 \\ 1 - 5 \end{matrix})$

$\vec{A B} = (\begin{matrix} 10 \\ - 4 \end{matrix})$

(b) Find the modulus of vector $\vec{A B}$ .

Sketching a diagram of the vector $\vec{A B}$ can help

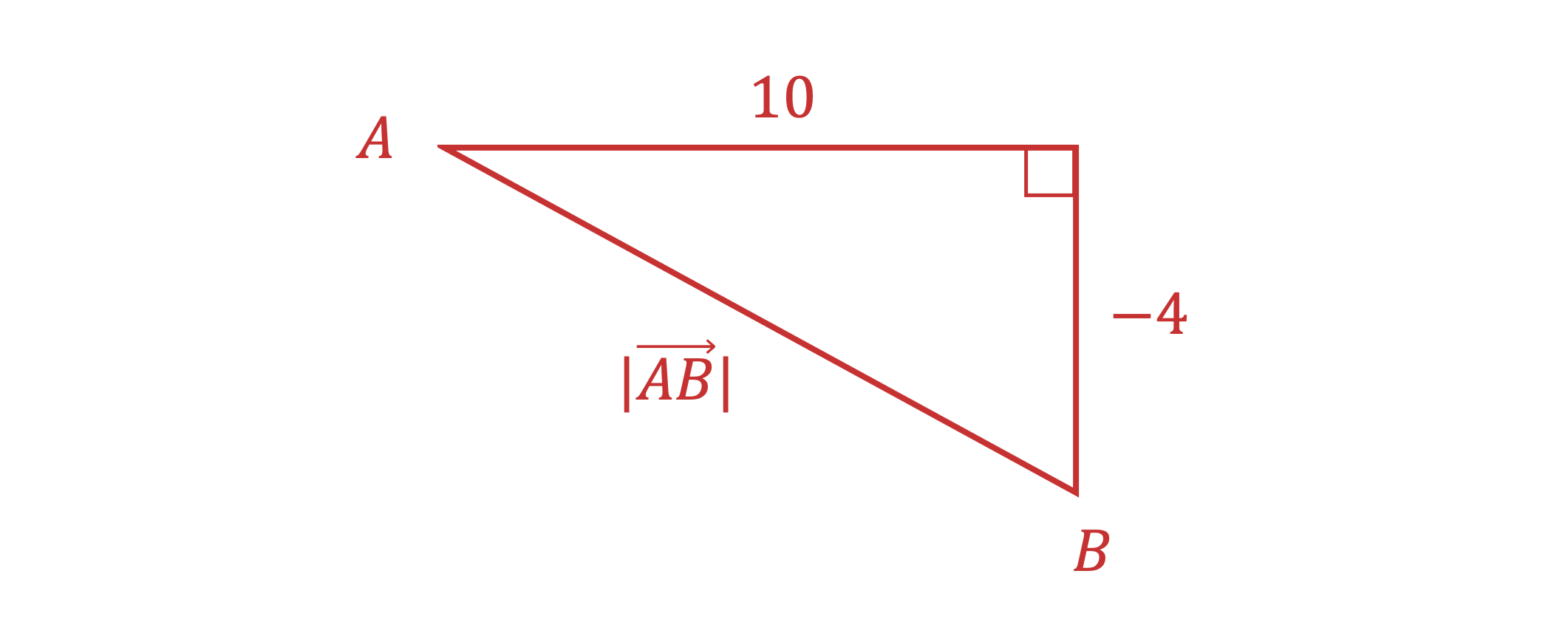
Right-angled triangle formed from points A and B. The horizontal distance is 10, the vertical distance is 4 and the hypotenuse is the magnitude of the vector from A to B.

Apply Pythagoras' theorem to the x and y components of $\vec{A B}$

$\begin{array}{rcl} |\vec{A B}| & = & \sqrt{10^{2} + {(- 4)}^{2}} \\ = & \sqrt{100 + 16} \\ = & \sqrt{116} \end{array}$

$\begin{array}{rcl} | \vec{A B} | & = & 2 \sqrt{29} \end{array}$

The magnitude of a vector is it's 'size'

Direction of the vector is ignored

$|\vec{B A}| = |\vec{A B}|$ since both vectors have the same distance

Another vector, $\vec{C D}$ , has three times the magnitude of vector $\vec{A B}$ .

(d) Write down a possible column vector for $\vec{C D}$ .

Being three times $|\vec{A B}|$ means the vector $\vec{A B}$ is three times longer

One way to find a vector is to multiply each component of the vector $\vec{A B}$ by 3 or -3

$\begin{array}{rcl} \vec{C D} & = & 3 \vec{A B} = 3 (\begin{matrix} 10 \\ - 4 \end{matrix}) \end{array}$

$\vec{C D} = (\begin{matrix} 30 \\ - 12 \end{matrix})$

Another possible answer is $\vec{C D} = (\begin{matrix} - 30 \\ 12 \end{matrix})$

Last updated: 18 October 2024

You've read 0 of your 10 free revision notes

Unlock more, it's free!

Join the 100,000+ Students that ❤️ Save My Exams

the (exam) results speak for themselves:

Did this page help you?

Previous:Representing Vectors as DiagramsNext:Translations