Similar Areas & Volumes (Cambridge (CIE) IGCSE International Maths)

Revision Note

Written by: Amber

Reviewed by: Dan Finlay

Did this video help you?

Two shapes are mathematically similar if one is an enlargement of the other
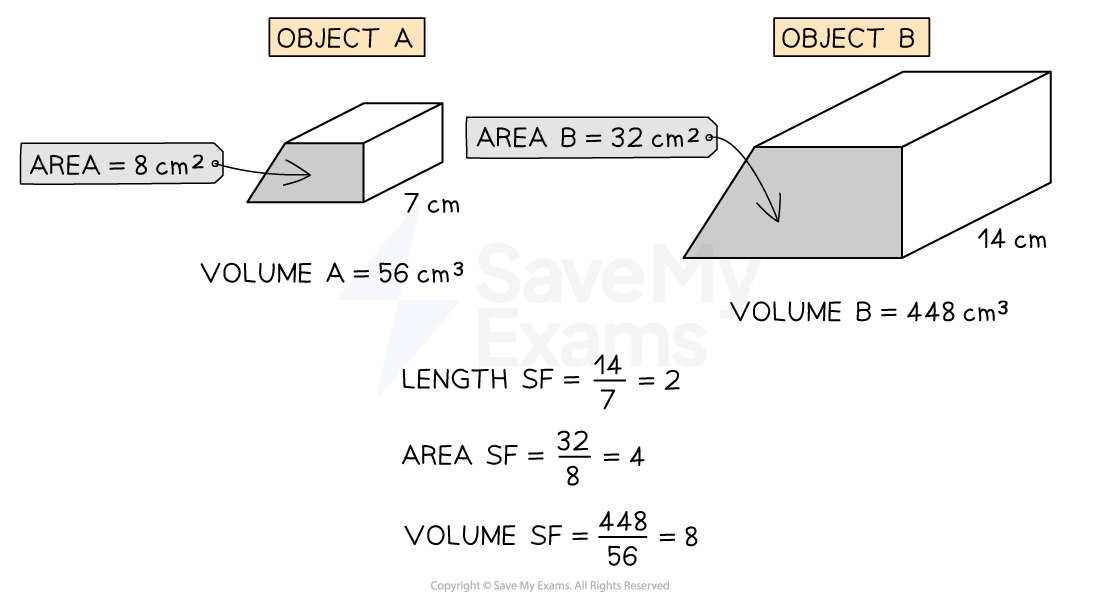
If the lengths of two similar shapes are linked by the scale factor, k
- Equivalent areas are linked by an area factor, k²
- Equivalent volumes are linked by a volume factor, k³
The scale factor (SF) for a given quantity (length, area or volume), can be found using the formula: $scale factor = \frac{second quantity}{first quantity}$

An object could be made either bigger or smaller by a scale factor
- When k > 1, the object is getting bigger
  - This is also true for k² > 1 and k³ > 1
- When 0 < k < 1, the object is getting smaller
  - This is also true for 0< k² < 1 and 0 < k³ < 1

STEP 1
Identify the equivalent known quantities
- Recognise if the quantities are lengths, areas or volumes
STEP 2
Find the scale factor from two known lengths, areas or volumes
- $scale factor = \frac{second quantity}{first quantity}$
- For two lengths, k = length SF
- For two areas, k² = area SF
- For two volumes, k³ = volume SF
STEP 3
Check the scale factor
- SF > 1 if getting bigger
- 0 < SF < 1 if getting smaller

STEP 4
If necessary, use the scale factor you have found to find other scale factors
- If you have the length scale factor
  - $Area scale factor = {(Length scale factor)}^{2}$
  - $Volume scale factor = {(Length scale factor)}^{3}$
- If you have the area scale factor
  - $Length scale factor = \sqrt{(Area scale factor)}$
  - Find the volume scale factor by finding the length scale factor first
- If you have the volume scale factor
  - $Length scale factor = \sqrt[3]{(Volume scale factor)}$
  - Find the area scale factor by finding the length scale factor first
STEP 5
Multiply or divide by relevant scale factor to find a new quantity

Take extra care not to mix up which shape is which when you have started carrying out the calculations
- It can help to label the shapes and write an equation

Solid A and solid B are mathematically similar.

The volume of solid A is 32 cm³.
The volume of solid B is 108 cm³.
The height of solid A is 10 cm.

Find the height of solid B.

Calculate $k^{3}$ , the scale factor of enlargement for the volumes, using: $volume B = k^{3} (volume A)$

Or $k^{3} = \frac{larger volume}{smaller volume}$

$\begin{array}{rcl} 108 & = & 32 k^{3} \\ k^{3} & = & \frac{108}{32} = \frac{27}{8} \end{array}$

Find the length scale factor $k$ by taking the cube root of the volume scale factor $k^{3}$

$k = \sqrt[3]{\frac{27}{8}} = \frac{3}{2}$

Substitute the value for $k$ into formula for the heights of the similar shapes:

$Height B = k (height A)$

$\begin{array}{rcl} h & = & 10 k \\ h & = & 10 (\frac{3}{2}) = \frac{30}{2} = 15 \end{array}$

Height of B = 15 cm

Last updated: 18 October 2024