Logarithms (Cambridge (CIE) IGCSE International Maths)

Revision Note

Author

Mark Curtis

Expertise

Maths

Logarithms

What are logarithms?

A logarithm is the inverse function of an exponential function
- Making $x$ the subject of $a^{x} = b$ gives $x = \log_{a} (b)$
  - This is pronounced "log base a of b"
  - This is the definition of a logarithm
  - The number $a$ is called the base of the logarithm
If no base is given, it means the base number is 10
- $\log (. . .)$ means $\log_{10} (. . .)$
  - In the past, this was a common base to use
  - The log button on a calculator is always base 10

How do I interpret logarithms?

log base a of b, $\log_{a} (b)$ , is the power that you raise $a$ to, to get $b$
- This is the power required to take the small number, $a$ , to the big number, $b$
- For example, $\log_{5} 125$ is the power needed to get 5 to 125
  - The power needed is 3 (because 5³ = 125)
  - So $\log_{5} 125 = 3$
- Similarly
  - $\log_{5} 25 = 2$
  - $\log_{5} 5 = 1$
  - $\log_{5} 1 = 0$ (as $5^{0} = 1$ )
- It works for negative powers (reciprocals)
  - $\log_{5} (\frac{1}{5}) = - 1$ (as $\frac{1}{5} = 5^{- 1}$ )
  - $\log_{5} (\frac{1}{25}) = \log_{5} (\frac{1}{5^{2}}) = - 2$
- It works for fractional powers (roots)
  - $\log_{5} (\sqrt{5}) = \frac{1}{2}$ (as $\sqrt{5} = 5^{\frac{1}{2}}$ )
  - $\log_{5} (\sqrt[3]{5}) = \frac{1}{3}$
Where possible, use a calculator to check
- Use the $\log_{. . .} (. . .)$ button which allows you to change the base number
  - This is a different button to the log button (base 10)

How do I convert between logarithms and exponentials?

$\log_{a} (b) = x$ and $a^{x} = b$ are mathematically identical statements
- You can convert between the two
Learn to read $\log_{a} (b) = x$ as " $x$ is the power that you raise $a$ to, to get $b$ "
- This allows you to convert logs to exponentials
  - $a^{x} = b$
This also works the other way
- $a^{x} = b$ means $x = \log_{a} (b)$
  - Though it is often easier to think of making $x$ the subject
  - To make $x$ the subject, take log base a of both sides, $\log_{a}$
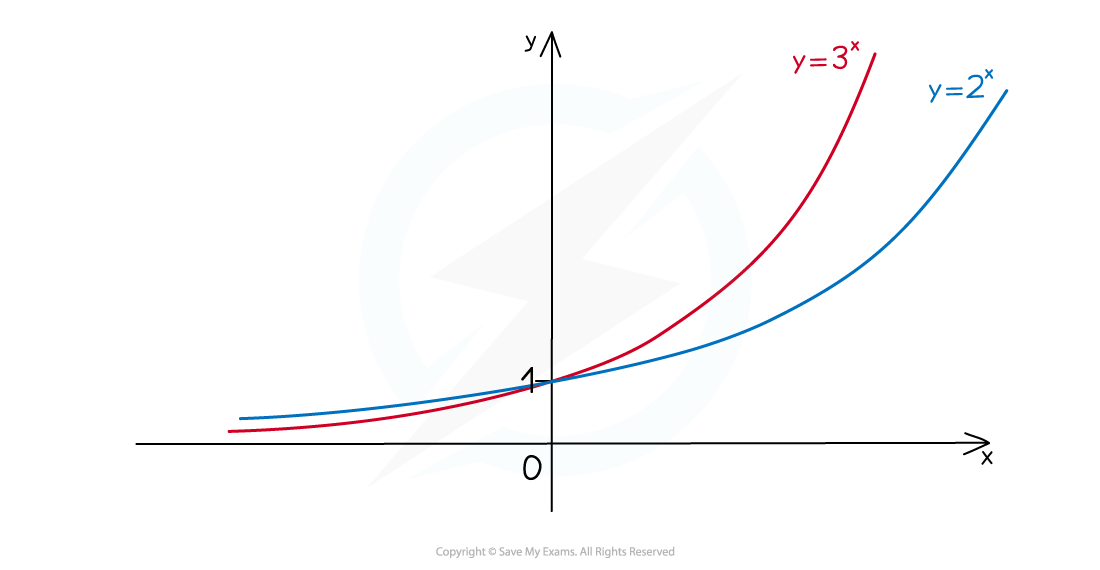
This means you can relate logarithms to exponential graphs
- e.g. the graph of $y = 3^{x}$ passes through the point $(x, 81)$
  - $3^{x} = 81$ so $x = \log_{3} 81 = 4$

Exam Tip

In the exam, you can rewrite a logarithm question as an exponential question if you prefer.

How do I solve equations with logarithms?

Equations where $x$ is in the power are called exponential equations
- Some can be solved easily by inspection (seeing the answer)
  - For example, $2^{x} = 8$ is solved by $x = 3$
- Others cannot
  - For example, $2^{x} = 10$
- These must be solved using logarithms by making $x$ the subject
  - $2^{x} = 10$ means $x = \log_{2} 10$
  - Type this into your calculator to get $x = 3.321928 . . .$
Alternatively, you can also solve equations using logs of base 10 only
- The rule for base 10 is if $a^{x} = b$ then $x = \frac{\log b}{\log a}$ where $\log$ means base 10
- For example, $2^{x} = 10$ means $x = \frac{\log 10}{\log 2} = 3.321928 . . .$

Exam Tip

Whilst you still need to know both methods, mark schemes will accept different correct answers with logarithms, depending on whether you have used logs of base 10 or logs of a different base.

How do I use logarithms in compound interest questions?

You can use logarithms to work out the power, $n$ , in compound interest questions
- e.g.you put £500 into a bank account with 5% interest per year; after how many whole years does it exceed £900?
  - $500 \times 1 . 05^{n} = 900$
  - Make $n$ the subject: $1 . 05^{n} = \frac{900}{500} = 1.8$ so $n = \log_{1.05} (1.8)$ (or $n = \frac{\log 1.8}{\log 1.05}$ )
  - This gives $n = 12.047236 . . .$ so $n = 13$ years
  - (it has not exceeded 900 after 12 years)

How do I find inverse functions using logarithms?

For exponential functions, you can use logarithms to find their inverse functions
- Use the standard process for finding inverse functions
- e.g. $f (x) = 2^{4 x}$
  - $y = 2^{4 x}$ then swap $x$ and $y$ to get $x = 2^{4 y}$
  - Make $4 y$ the subject using logarithms: $4 y = \log_{2} x$
  - Finally make $y$ the subject to give $f^{- 1} (x) = \frac{1}{4} \log_{2} x$

Worked Example

(a) Solve the equation $4^{x} = 20$ , giving your answer correct to 3 significant figures.

Method 1

To make $x$ the subject, take log base 4 of both sides

$x = \log_{4} (20)$

Use the $\log_{. . .} (. . .)$ button on your calculator to find $x$

$x = 2.1609640 . . .$

Round to 3 significant figures

$x = 2.16$ to 3 s.f.

Method 2

Using logs of base 10, solve $a^{x} = b$ by using $x = \frac{\log b}{\log a}$

$x = \frac{\log 20}{\log 4}$

Use the log button on your calculator to find $x$

$x = 2.1609640 . . .$

Round to 3 significant figures

$x = 2.16$ to 3 s.f.

(b) Without using a calculator, solve the equation $\log (8 x) = 4$

log with no base means log base 10

$\log_{10} (8 x) = 4$

Rewrite this statement as a exponential relationship
4 is the power that you raise 10 to, to get $8 x$

$10^{4} = 8 x$

Simplify and make $x$ the subject

$10 000 = 8 x \frac{10 000}{8} = x$

$x = 1250$

Take log base 7 of both sides

$p (t - 1) = \log_{7} (2 q)$

Rearrange to make $t$ the subject
Start by dividing both sides by $p$

$t - 1 = \frac{1}{p} \log_{7} (2 q)$

Then add 1 to both sides

$t = 1 + \frac{1}{p} \log_{7} (2 q)$

$t = 1 + \frac{\log (2 q)}{p \log 7}$ in base 10 is also accepted

You've read 0 of your 10 free revision notes

Unlock more, it's free!

Join the 100,000+ Students that ❤️ Save My Exams

the (exam) results speak for themselves:

Next topic

Did this page help you?

Logarithms (Cambridge (CIE) IGCSE International Maths)

Revision Note

Logarithms

What are logarithms?

How do I interpret logarithms?

How do I convert between logarithms and exponentials?

Exam Tip

How do I solve equations with logarithms?

Exam Tip

How do I use logarithms in compound interest questions?

How do I find inverse functions using logarithms?

Worked Example

You've read 0 of your 10 free revision notes

Unlock more, it's free!

Join the 100,000+ Students that ❤️ Save My Exams

Author: Mark Curtis