Exponential Growth & Decay (Cambridge (CIE) IGCSE International Maths)

Revision Note

Author

Expertise

Maths

Exponential Growth & Decay

The ideas of compound interest and depreciation can be applied to other (non-money) situations, such as increasing or decreasing populations.

What is exponential growth?

When a quantity grows exponentially it is increasing from an original amount by a scale factor (or percentage) each year for $x$ years
- Some questions use a different timescale, such as each day, or each minute
Real-life examples of exponential growth include:
- Population increases
- Bacterial growth
- The number of people infected by a virus

What is exponential decay?

When a quantity exponentially decays it is decreasing from an original amount by a scale factor (or percentage) each year for $x$ years
- Some questions use a different timescale, such as each day, or each minute
Real-life examples of exponential decay include:
- The temperature of hot water cooling down
- The value of a car decreasing over time
- Radioactive decay (the mass of a radioactive a substance over time)

How do I use models for exponential growth or decay?

Scenarios which exponentially grow or decay can be modelled with an equation
A useful format for this equation is
- $y = a^{x}$
  - E.g. $y = 4^{x}$ or $y = 0 . 8^{x}$
- If $a > 1$ then it is exponential growth
- If $0 < a < 1$ then it is exponential decay
- $a$ cannot be negative
$y = 3^{x}$ could model the number of flies, $y$ , in a population of flies, against the number of days, $x$
- The initial number of flies, when $x = 0$ , is $3^{0} = 1$
- After 2 days, the number would be $3^{2} = 9$
- After 4 days, the number would be $3^{4} = 81$
- From the values above, and the fact that $a > 1$ , this is exponential growth
$y = 0 . 5^{x}$ could model the radius of a water droplet, $y cm$ , as it evaporates where $x$ is measured in minutes
- The initial radius of the droplet, when $x = 0$ , is $0 . 5^{0} = 1 cm$
- After 2 minutes, the radius would be $0 . 5^{2} = 0.25 cm$
- After 4 minutes, the radius would be $0 . 5^{4} = 0.0625 cm$
- From the values above, and the fact that $0 < a < 1$ , this is exponential decay

Plotting an exponential model, $y = a^{x}$ , on a set of axes gives an exponential graph with the following shape

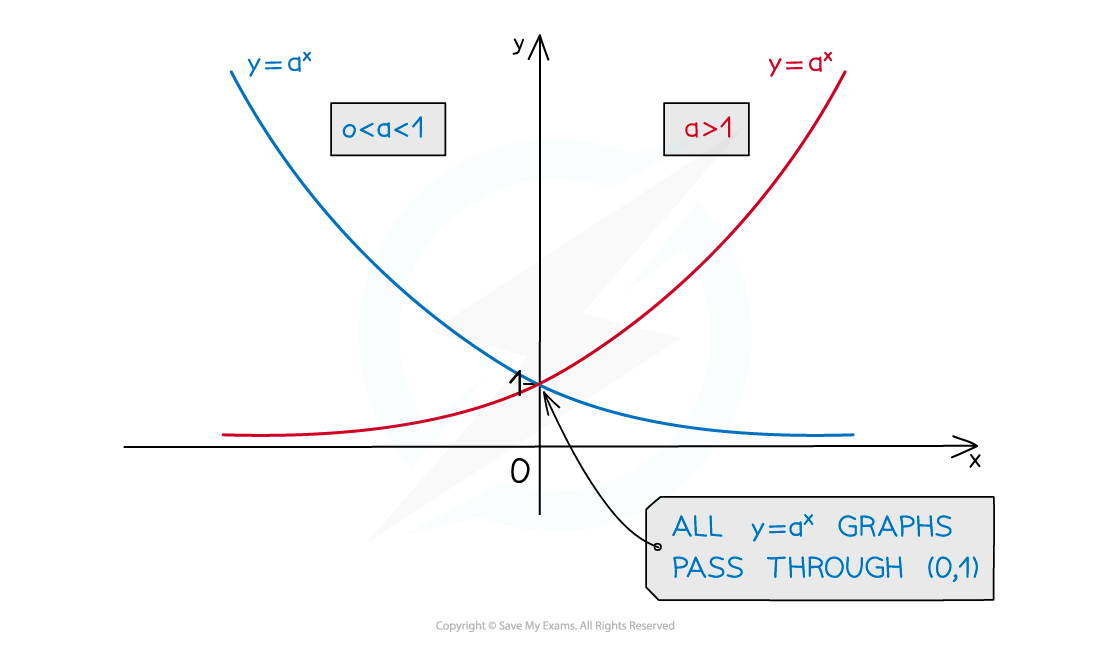
downward exponential curve for y=a^x where 0<a<1 and upward exponential curve for when a>1. The y intercept is always (0,1)

The $y$ -intercept is always 1
- This is because the $y$ -intercept is where $x = 0$
- $y = a^{0} = 1$
There is an asymptote at $y = 0$ (the curve gets closer and closer to the x-axis without crossing it)
- This is because there are no solutions to $0 = a^{x}$

Are there any other exponential models I may encounter?

You may see exponential models in the form $y = b a^{x}$
- The coefficient, $b$ , scales (stretches) the graph vertically
- The $y$ -intercept will be $(0, b)$
  - This is because when $x = 0$ , $y = b a^{0} = b (1) = b$
- $b$ is the initial (starting) amount of the quantity being modelled

Exam Tip

Look out for how the question wants you to give your final answer
- It may want the final amount to the nearest thousand or to the nearest integer

Worked Example

A large population of birds increases exponentially according to the model

$N = 1 . 04^{t}$

where $N$ is the number of birds, measured in thousands, and $t$ is the number of years.

Find the population after 13 years, giving your answer to the nearest hundred.

Substitute in $t = 13$

$N = 1 . 04^{13} N = 1.665073507 . . .$

The question asks for the answer to the nearest hundred
The question also states that $N$ is measured in thousands

1.665073507... × 1000 = 1665.073507... birds

Round to nearest hundred

1700

Worked Example

The percentage of tickets still available for a concert is modelled approximately by the equation

$y = a^{x}$

Where $y$ is the percentage of tickets still available, as a decimal, and $x$ is the number of days since the tickets have been released.

(a) After 3 days, 51.2% of the tickets are still available. Find the value of $a$ .

$x$ will be 3 (from 3 days)
$y$ will be 0.512 (as the percentage is written as a decimal in this model)
Substitute these values into the model

$0.512 = a^{3}$

Find $a$ by taking the cube root of both sides

$\begin{array}{rcl} \sqrt[3]{0.512} & = & a \\ 0.8 & = & a \end{array}$

$a = 0.8$

(b) What percentage of tickets remain after 2 weeks? State your answer to three significant figures.

Now that $a$ is known, the full equation of the model is

$y = 0 . 8^{x}$

$x$ is measured in days

2 weeks = 2 × 7 = 14 days

Substitute in $x = 14$

$y = 0 . 8^{14} = 0.04398046511 . . .$

Turn this into a percentage by multiplying by 100

4.398046511... %

Round to three significant figures

4.40 %

The value of y can never reach zero (there is an asymptote at $y = 0$ ).
The model suggests that the tickets never truly run out (which is unrealistic).

You've read 0 of your 10 free revision notes

Unlock more, it's free!

Join the 100,000+ Students that ❤️ Save My Exams

the (exam) results speak for themselves:

Next topic

Did this page help you?

Exponential Growth & Decay (Cambridge (CIE) IGCSE International Maths)

Revision Note

Exponential Growth & Decay

What is exponential growth?

What is exponential decay?

How do I use models for exponential growth or decay?

How are exponential models related to exponential graphs?

Are there any other exponential models I may encounter?

Exam Tip

Worked Example

Worked Example

You've read 0 of your 10 free revision notes

Unlock more, it's free!

Join the 100,000+ Students that ❤️ Save My Exams

Author: Jamie Wood