IGCSEMathsCambridge (CIE)International MathsCoreRevision NotesGeometryCircle TheoremsTwo Tangents from a Point

Two Tangents from a Point (Cambridge (CIE) IGCSE International Maths): Revision Note

Exam code: 0607

Naomi C

Written by: Naomi C

Reviewed by: Dan Finlay

Updated on

Two Tangents from a Point

Circle Theorem: Tangents from an external point are equal in length

  • Two tangents from the same external point are equal in length

  • This means that a kite can be formed by two tangents meeting a circle

    • The kite below has a vertical line of symmetry

      • It is formed from two congruent triangles back-to-back

    • The kite will have two right angles where the tangents meet the radii

      • You can use Pythagoras and SOHCAHTOA on each of these triangles

A circle with centre, O, and two points on the circumference, R and S. Tangents to the circle at these two points, intersect at a point outside the circle, T. OR and OS are radii. ROST forms a kite.

Examiner Tips and Tricks

  • Look for tangents in the exam and draw on the radius at right angles to see if it helps

Worked Example

Consider the diagram below.

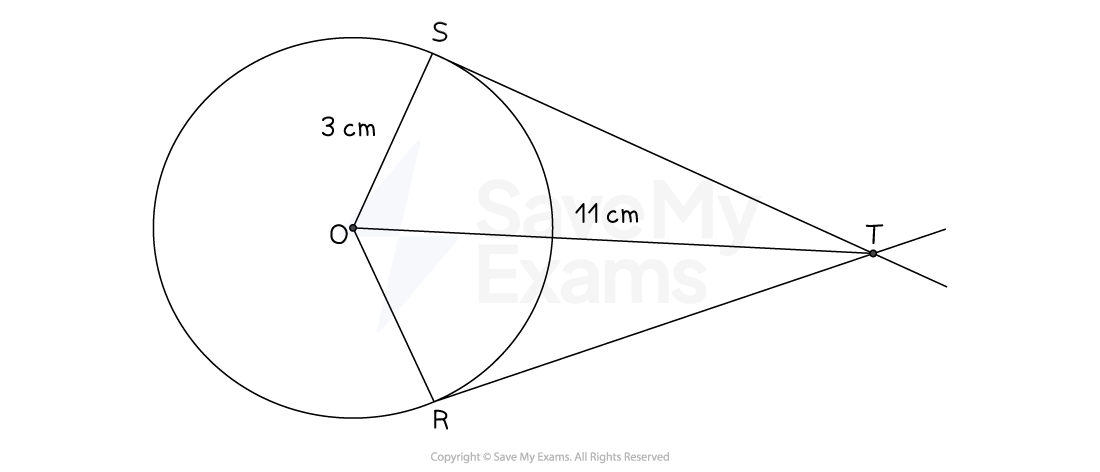
A circle with centre, O, and two points on the circumference, S and R. Tangents to the circle, at S and R, meet at a point outside the circle, T. OR = 3 cm, OT = 11 cm.

(a) Find the length ST.

The lines ST and RT are both tangents to the circle
They meet the two radii on the circumference at the points S and T

Angle TSO = 90° because a radius and a tangent meet at right angles

Use Pythagoras' theorem on the right-angled triangle OST to calculate the length ST

table row cell 3 squared plus open parentheses S T close parentheses squared end cell equals cell 11 squared end cell row cell 9 plus open parentheses S T close parentheses squared end cell equals 121 row cell open parentheses S T close parentheses squared end cell equals 112 row cell S T end cell equals cell square root of 112 equals 10.58300... end cell end table

ST = 10.6 cm (3 s.f.)

(b) Find the length RT.

Two tangents from the same external point are equal in length

ST = RT

RT = 10.6 cm (3 s.f.)

👀 You've read 1 of your 5 free revision notes this week
An illustration of students holding their exam resultsUnlock more revision notes. It's free!

By signing up you agree to our Terms and Privacy Policy.

Already have an account? Log in

Test yourself

Did this page help you?

Previous:Tangent & RadiusNext:Perimeter
Author
Reviewer
Naomi C

Author: Naomi C

Expertise: Maths Content Creator

Naomi graduated from Durham University in 2007 with a Masters degree in Civil Engineering. She has taught Mathematics in the UK, Malaysia and Switzerland covering GCSE, IGCSE, A-Level and IB. She particularly enjoys applying Mathematics to real life and endeavours to bring creativity to the content she creates.

Dan Finlay

Reviewer: Dan Finlay

Expertise: Maths Subject Lead

Dan graduated from the University of Oxford with a First class degree in mathematics. As well as teaching maths for over 8 years, Dan has marked a range of exams for Edexcel, tutored students and taught A Level Accounting. Dan has a keen interest in statistics and probability and their real-life applications.

Download notes on Two Tangents from a Point