Classifying Stationary Points (Cambridge (CIE) IGCSE Maths): Revision Note
Exam code: 0580 & 0980
Did this video help you?
Classifying stationary points
What are the different types of stationary points?
You need to know two different types of stationary points:
Maximum points (this is where the graph reaches a “peak”)
Minimum points (this is where the graph reaches a “trough”)
These are also called turning points
Deciding which is which is called classifying them (or finding the nature of them)

If a graph has multiple turning points, the one you are interested in can be described as a local maximum or minimum point
Other parts of the graph may still reach higher/lower values elsewhere
How do I classify turning points using graphs?
You can use the shape of common graphs to classify different turning points
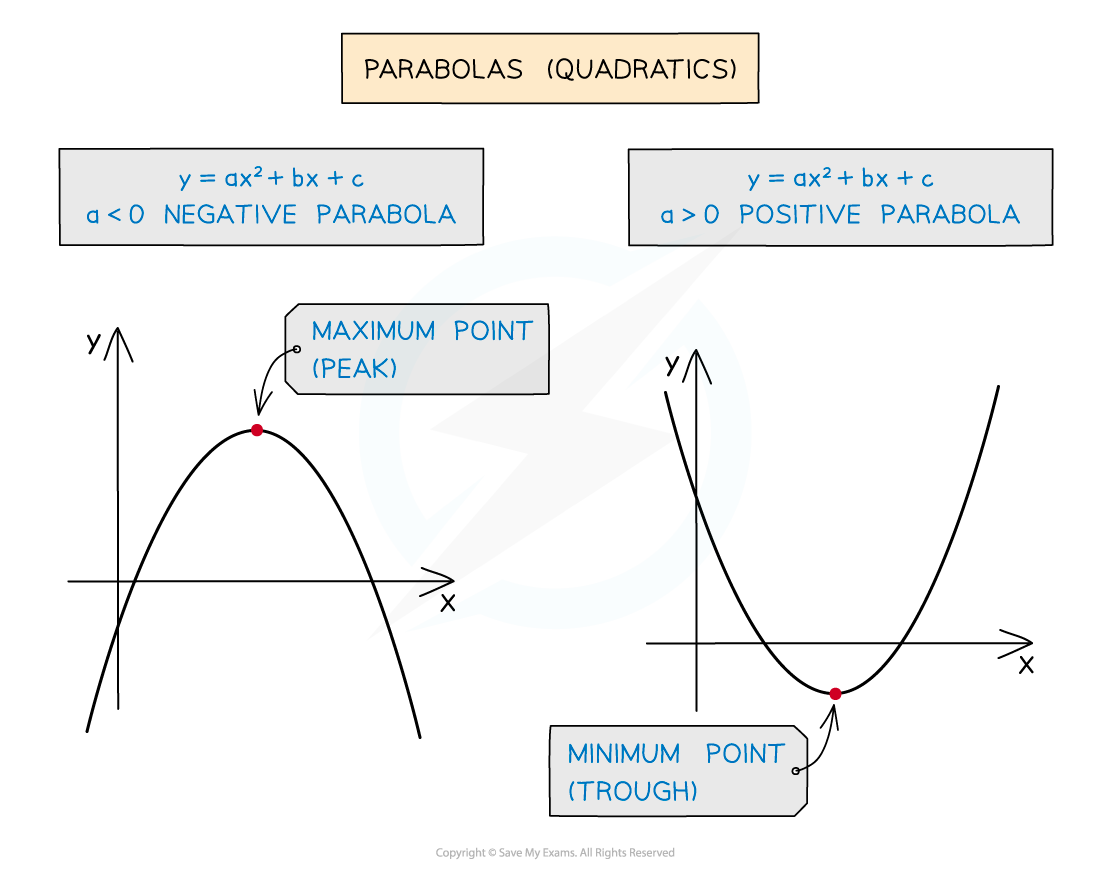
To classify the turning point on a quadratic curve (parabola), remember that:
A positive quadratic (positive x2 term) must have a minimum point
A negative quadratic (negative x2 term) must have a maximum point
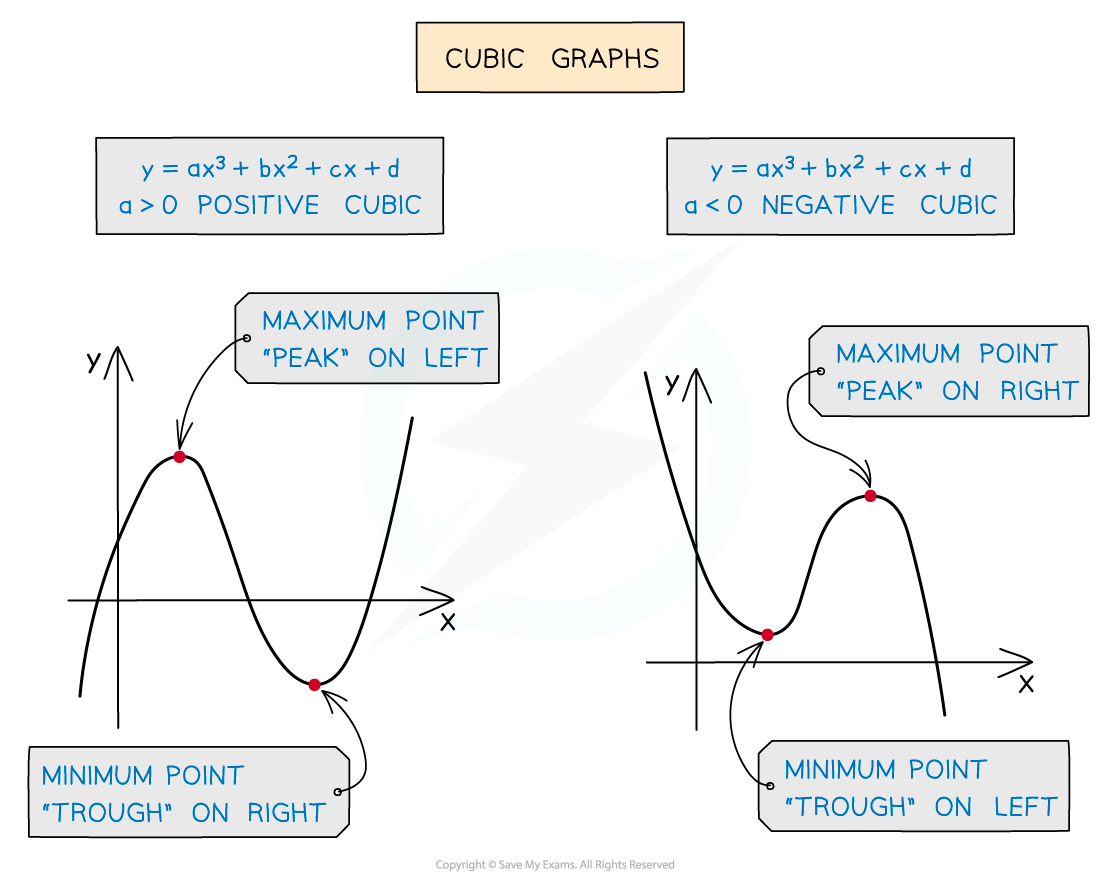
To classify two different turning points on a cubic curve, remember that:
A positive cubic has a maximum point on the left and a minimum on the right
A negative cubic has a minimum point on the left and a maximum on the right
How do I classify turning points using the gradient function (derivative)?
An alternative method if no graph is available is to use the gradient function (derivative)
By looking at the gradient just before and just after a turning point, you can determine if it is a maximum or a minimum
Find the gradient just before and just after a turning point by substituting values near to the value of x into the gradient function
It helps to imagine what the tangents to the curve would look like
Gradient before turning point | Gradient at turning point | Gradient after turning point | Type of turning point |
|---|---|---|---|
Positive / | Zero ⁻ | Negative \ | Maximum /—\ |
Negative \ | Zero _ | Positive / | Minimum \_/ |
How do I classify turning points using the second derivative?
Another method is to use the second derivative,
, which is the derivative-of-the-derivative
is pronounced "d-two-y by dx-squared"
Differentiate the equation once to get
then again to get
This is the same as differentiating the original equation for
twice
If the stationary point is at
, substitute
into the expression for
to get a numerical value
If the result is negative,
, then the stationary point is a maximum point
This is often the opposite of what people expect!
If the result is positive,
, then the stationary point is a minimum point
If the value is zero,
, then the test has failed
You will need to try another method to classify the turning point
Worked Example
The turning points on the curve with equation are (-2, 21) and (1, -6).
Determine the nature of these turning points.
Method 1: Using the graph
Make a quick sketch of the curve
It is a positive cubic so you know that it will start at the bottom left, have two turning points and go towards the top right
You know the coordinates of the turning points and the y-intercept: (0, 1)
(the sketch does not have to be perfect!)
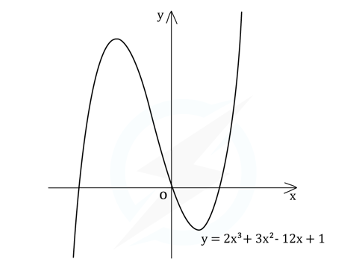
From the graph, which is a positive cubic,
(-2, 21) is to the left of (1, -6)
Therefore, (-2, 21) is a maximum point and (1, -6) is a minimum point
Method 2: Differentiation (1st derivative)
Differentiate the original equation to find the 1st derivative, the gradient function,
Find the gradient just before and just after each turning point by substituting a suitable x-value into the gradient function
Turning point | Gradient before | Gradient after | |||||
(-2, 21) | sub x = -3 6(-3)2 + 6(-3) - 12 = 24 | sub x = -1 6(-1)2 + 6(-1) - 12 = -12 | |||||
(1, -6) | sub x = 0 6(0)2 + 6(0) - 12 = -12 | sub x = 2 6(2)2 + 6(2) - 12 = 24 | |||||
The gradient just before (-2, 21) is positive and the gradient just after is negative, therefore the turning point here is a maximum point
The gradient just before (1, -6) is negative and the gradient just after is positive, therefore the turning point here is a minimum point
Method 3: Differentiation (2nd derivative)
Differentiate the original equation to find the 1st derivative
Differentiate again to find the 2nd derivative
Find the nature of each turning point by substituting the value of its x-coordinate into the 2nd derivative
A negative result indicates a maximum point (or positive for a minimum point)
For (-2, 21):
The second derivative is negative, therefore the turning point at (-2, 21) is a maximum point
For (1, -6):
The second derivative is positive, therefore the turning point at (1, -6) is a minimum point

Unlock more, it's free!
Did this page help you?