IGCSEMathsCambridge (CIE)ExtendedRevision NotesCoordinate Geometry & GraphsCoordinate GeometryLength of a Line

Length of a Line (Cambridge (CIE) IGCSE Maths) : Revision Note

Jamie Wood

Written by: Jamie Wood

Reviewed by: Dan Finlay

Updated on

Cambridge (CIE) Maths: ExtendedEdexcel Maths A (Modular): Higher Unit 1Edexcel Maths A: HigherCambridge (CIE) International Maths: Extended

Length of a Line

How do I calculate the length of a line?

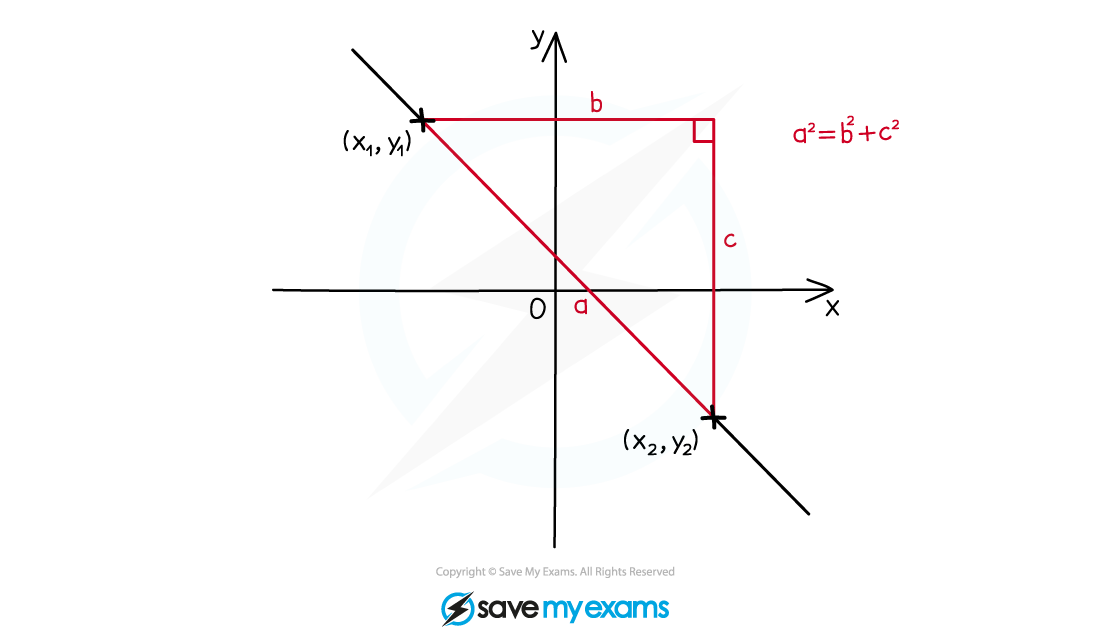
  • The distance between two points with coordinates open parentheses x subscript 1 space comma space y subscript 1 close parentheses and open parentheses x subscript 2 space comma space y subscript 2 close parentheses can be found using the formula

d equals square root of open parentheses x subscript 1 minus x subscript 2 close parentheses squared plus open parentheses y subscript 1 minus y subscript 2 close parentheses squared end root

  • This formula uses Pythagoras’ theorem  a squared plus b squared equals c squared

    • It is applied to the difference in the x-coordinates and the difference in the y-coordinates

A right-angled triangle drawn between two point on a straight line, to show how Pythagoras is used to find the length of a line

Examiner Tips and Tricks

  • Be extra careful when negative coordinates are involved

    • It can help to put negative numbers in brackets to make your working clearer

    • E.g. open parentheses negative 6 close parentheses minus open parentheses negative 8 close parentheses equals 2

Worked Example

Point A has coordinates (3, -4) and point B has coordinates (-5, 2).

Calculate the distance of the line segment AB.

Using the formula for the distance between two points, d equals square root of open parentheses x subscript 1 minus x subscript 2 close parentheses squared plus open parentheses y subscript 1 minus y subscript 2 close parentheses squared end root 

Substitute in the two given coordinates

d equals square root of open parentheses 3 minus open parentheses negative 5 close parentheses close parentheses squared plus open parentheses open parentheses negative 4 close parentheses minus 2 close parentheses squared end root

Be careful with the negative numbers
3 minus open parentheses negative 5 close parentheses equals 8 and open parentheses negative 4 close parentheses minus 2 equals negative 6

Simplify

d equals square root of open parentheses 8 close parentheses squared plus open parentheses negative 6 close parentheses squared end root space equals space square root of 64 plus 36 end root equals square root of 100 equals 10

10 units

👀 You've read 1 of your 5 free revision notes this week
An illustration of students holding their exam resultsUnlock more revision notes. It's free!

By signing up you agree to our Terms and Privacy Policy.

Already have an account? Log in

Test yourselfFlashcards

Did this page help you?

Previous:Gradient of a LineNext:Equations of Straight Lines (y = mx + c)
Author
Reviewer
Jamie Wood

Author: Jamie Wood

Expertise: Maths Content Creator

Jamie graduated in 2014 from the University of Bristol with a degree in Electronic and Communications Engineering. He has worked as a teacher for 8 years, in secondary schools and in further education; teaching GCSE and A Level. He is passionate about helping students fulfil their potential through easy-to-use resources and high-quality questions and solutions.

Dan Finlay

Reviewer: Dan Finlay

Expertise: Maths Lead

Dan graduated from the University of Oxford with a First class degree in mathematics. As well as teaching maths for over 8 years, Dan has marked a range of exams for Edexcel, tutored students and taught A Level Accounting. Dan has a keen interest in statistics and probability and their real-life applications.

Download notes on Length of a Line