Revision NotesPast Papers
IGCSEFurther MathsEdexcelRevision NotesSeriesArithmetic & Geometric SeriesSigma Notation

Sigma Notation (Edexcel IGCSE Further Pure Maths)

Revision Note

Roger B

Written by: Roger B

Reviewed by: Dan Finlay

Sigma Notation

What is sigma notation?

  • Sigma notation is used to show the sum of a certain number of terms in a sequence

    • The symbol Σ is the capital Greek letter sigma

  • Σ stands for ‘sum’

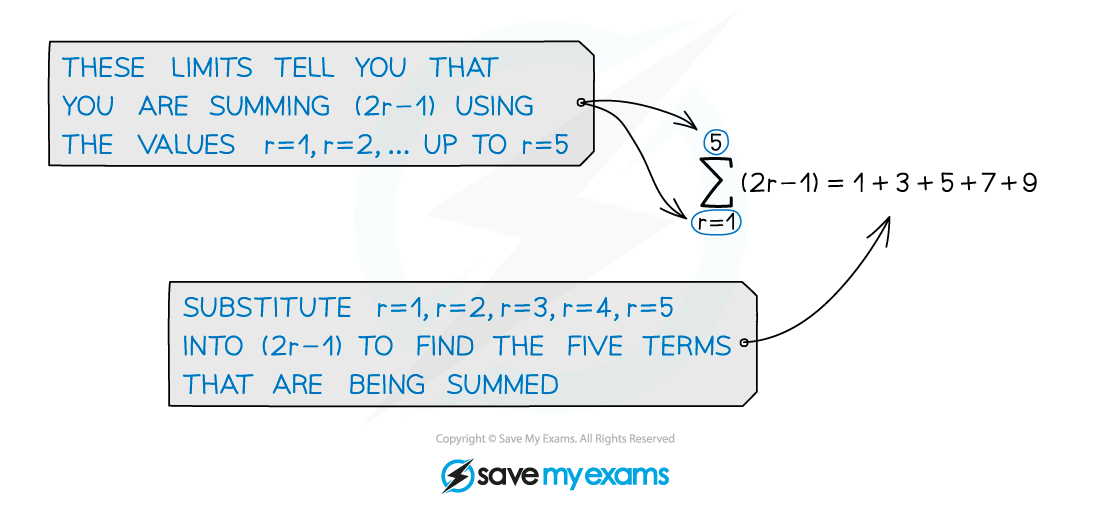
    • The expression to the right of the Σ tells you what is being summed

    • The limits above and below tell you which terms you are summing

    • r and k are both common variables to use when writing sigma sums

Sigma notation

  • Be careful, the limits don’t have to start with 1

    • For example sum from k space equals space 0 to 4 of left parenthesis 2 k plus 1 right parenthesis  or  sum from k space equals space 7 to 14 of left parenthesis 2 k minus 13 right parenthesis

  • You need to be able to read and use sigma notation when answering questions about series

    • For example the sum of the fifth through ninth terms of an arithmetic series

      • This could be written as  sum from k equals 5 to 9 of open parentheses a plus open parentheses k minus 1 close parentheses d close parentheses

    • Or the sum of the first n terms of a geometric series

      • This could be written as  sum from k equals 1 to n of a r to the power of k minus 1 end exponent

Examiner Tips and Tricks

  • Your calculator may be able to use sigma notation

    • If so make sure you know how it works 

    • You can use this to check your work

Worked Example

The terms of a series are defined by  u subscript n equals space 2 space cross times space 3 to the power of n minus 1 end exponent,  for  n equals 1 comma space 2 comma space 3 comma space... . 

(a) Write an expression for the sum of the first six terms of the series using sigma notation.

Use sum limits from 1 to 6

sum from k equals 1 to 6 of u subscript k space

Note that 'n' is replaced by 'k' to match the variable used for the sigma sum


Now replace u subscript k with the actual formula, being sure to use k instead of n again

bold sum from bold k bold equals bold 1 to bold 6 of stretchy left parenthesis 2 cross times 3 to the power of k minus 1 end exponent stretchy right parenthesis

(b) Write an expression for the sum of the seventh through twelfth terms of the series using sigma notation.

This will be the same as in part (a), except that the limits will be 7 on the bottom and 12 on the top

bold sum from bold k bold equals bold 7 to bold 12 of begin bold style stretchy left parenthesis 2 cross times 3 to the power of k minus 1 end exponent stretchy right parenthesis end style

(c) Write an expression for the sum of the first n terms of the series using sigma notation.

This is very similar to the above, but the sum limits will start at 1 and go to n

sum from k equals 1 to n of u subscript k

Be careful here  –  k is the variable for the sigma sum, and n means we're going up to the nth term

bold sum from bold k bold equals bold 1 to bold n of begin bold style stretchy left parenthesis 2 cross times 3 to the power of k minus 1 end exponent stretchy right parenthesis end style

Last updated:

You've read 0 of your 5 free revision notes this week

Sign up now. It’s free!

Join the 100,000+ Students that ❤️ Save My Exams

the (exam) results speak for themselves:

    Did this page help you?

    Previous:Solving Equations GraphicallyNext:Arithmetic Sequences & Series
    Author
    Reviewer
    Roger B

    Author: Roger B

    Expertise: Maths

    Roger's teaching experience stretches all the way back to 1992, and in that time he has taught students at all levels between Year 7 and university undergraduate. Having conducted and published postgraduate research into the mathematical theory behind quantum computing, he is more than confident in dealing with mathematics at any level the exam boards might throw at you.

    Dan Finlay

    Author: Dan Finlay

    Expertise: Maths Lead

    Dan graduated from the University of Oxford with a First class degree in mathematics. As well as teaching maths for over 8 years, Dan has marked a range of exams for Edexcel, tutored students and taught A Level Accounting. Dan has a keen interest in statistics and probability and their real-life applications.