Exponential Functions (Edexcel IGCSE Further Pure Maths): Revision Note

Exponential Functions & Graphs

What is an exponential function?

• An exponential function is of the form

• Its domain is the set of all real numbers

• Its range is the set of all positive real numbers

• An important exponential function is 

  • e is the mathematical constant 

  • See the following note on "" for more details

What are the key features of exponential graphs?

• The graphs have a -intercept at

  • Because  

• The graph will always pass through the point

  • Because  

• The graphs do not intersect the -axis

  • The graphs have a horizontal asymptote at the x-axis

• The graphs do not have any minimum or maximum points

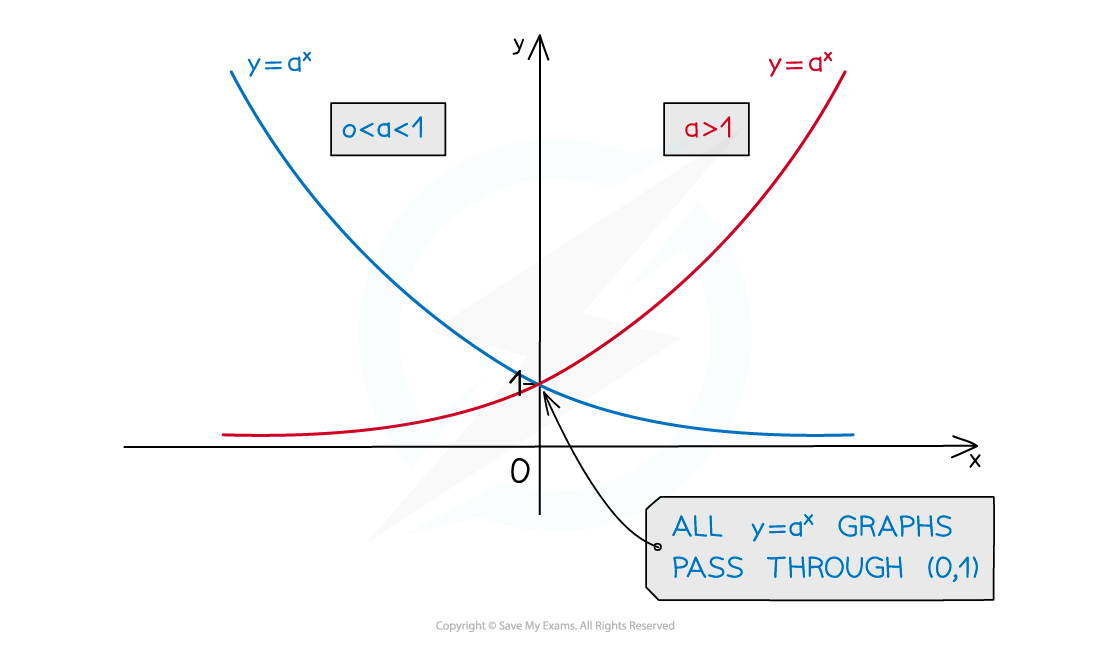
• When

  • For the higher value of is the “lower” graph

  • Where the higher value of is the “higher” graph
    

    

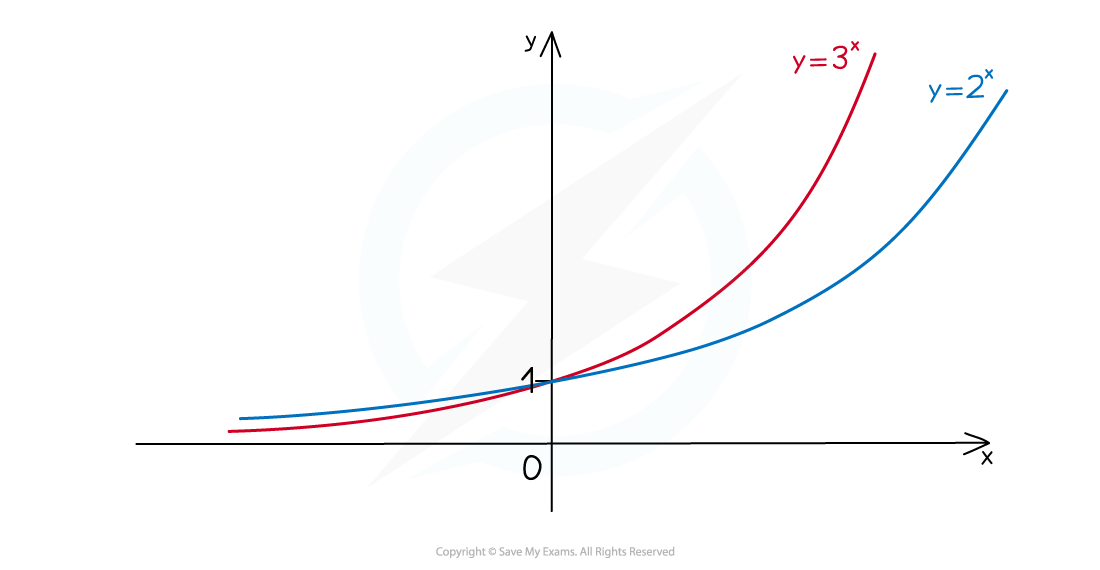
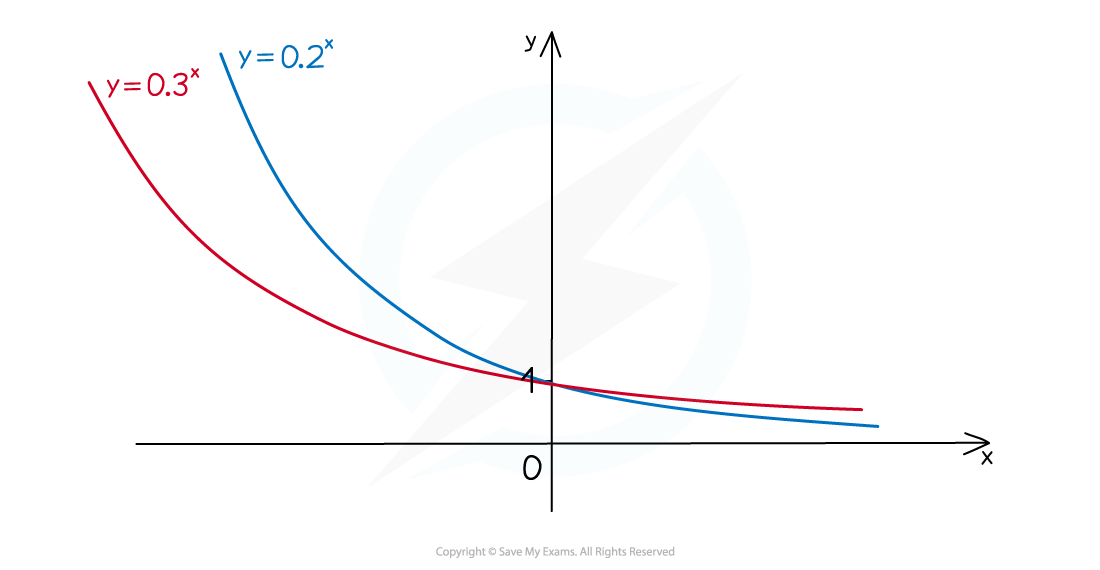
• When

  • Where the higher value of is the “lower” graph

  • Where the higher value of is the “higher” graph
    

    

• When 

  • The graph is a horizontal line through 

  • Because    for all values of 

"e"

What is e, the exponential constant?

•   is one of the most important mathematical constants

  • 

  • is an irrational number

  •   is often referred to as 'the exponential function'

• As with other exponential graphs,

  • passes through

  • has the -axis as an asymptote

  

Why is e so important?

• has the particular property that  

  • i.e. if  , then  is also equal to 

• This means that for every real number , the gradient of  is also equal to 

The negative exponential graph

•  is a reflection in the -axis of 

  • Note by laws of indices that 

• They are of the form  and