Tangents & Normals (Edexcel IGCSE Further Pure Maths)

Revision Note

Written by: Paul

Reviewed by: Dan Finlay

Tangents & Normals

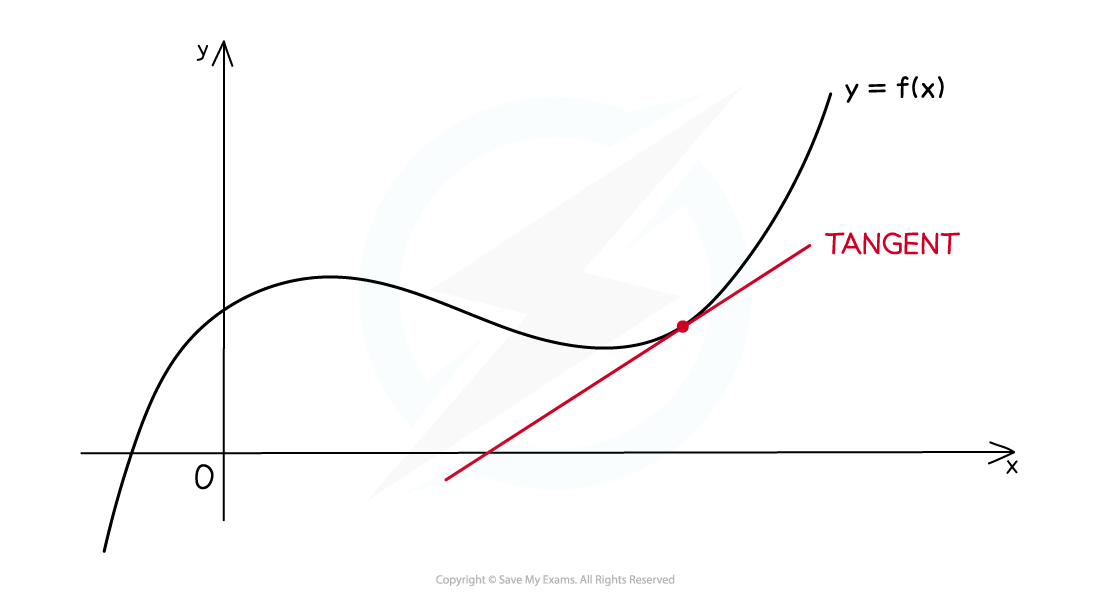
What is a tangent?

At any point on the graph of a (non-linear) function
- the tangent is the straight line that touches the graph at the point without cutting through it
- Its gradient is given by the derivative of the function

How do I find the equation of a tangent?

You need a point and the gradient to find the equation of a straight line
- The gradient of the tangent to the function $y = f (x)$ at the point ${(x}_{1}, y_{1})$ is $f^{'} (x_{1})$
Therefore to find the equation of the tangent to the function $y = f (x)$ at the point ${(x}_{1}, y_{1})$
- Find $f^{'} (x)$
- Substitute $x_{1}$ into $f' (x)$ to find the gradient $f^{'} (x_{1})$ ,
- Use the $y - y_{1} = m (x - x_{1})$ form of the line equation
  - $y - y_{1} = f^{'} (x_{1}) (x - x_{1})$
- Rearrange the equation into whatever form the question requires

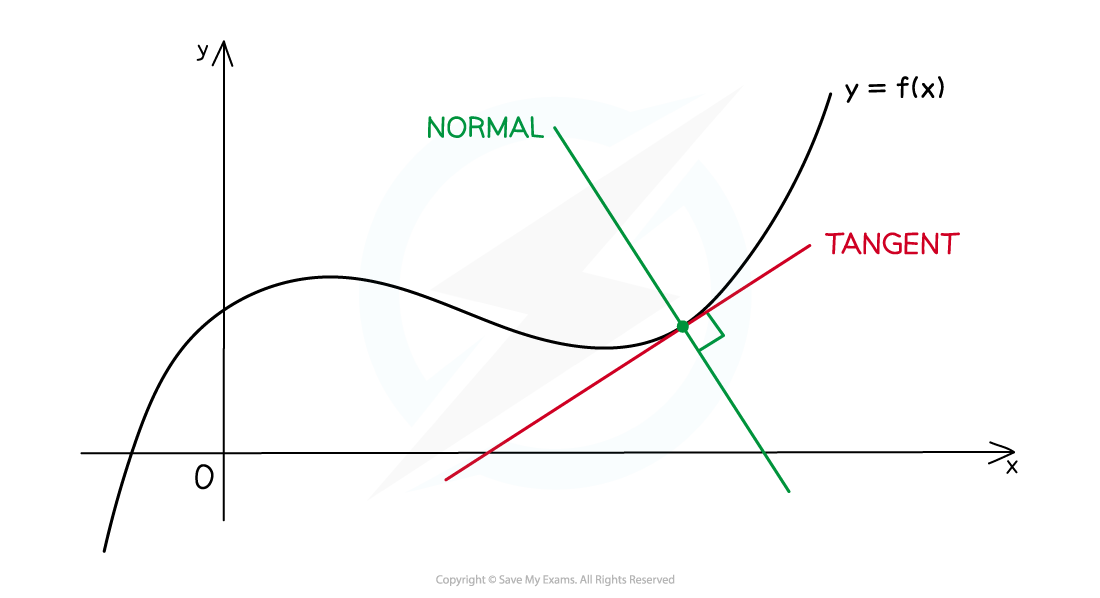
What is a normal?

At any point on the graph of a (non-linear) function
- the normal is the straight line that passes through that point
- and is perpendicular to the tangent

How do I find the equation of a normal?

You need a point and the gradient to find the equation of a straight line
- The tangent and the normal are perpendicular
- Therefore the gradient of the normal to the function $y = f (x)$ at the point ${(x}_{1}, y_{1})$ is $- \frac{1}{f^{'} (x_{1})}$
To find the equation of the normal to the function $y = f (x)$ at the point ${(x}_{1}, y_{1})$
- Find $f^{'} (x)$
- Substitute $x_{1}$ into $f' (x)$ to find the gradient of the tangent $f^{'} (x_{1})$
- Use that to find the gradient of the normal $- \frac{1}{f^{'} (x_{1})}$
- Use the $y - y_{1} = m (x - x_{1})$ form of the line equation
  - $y - y_{1} = - \frac{1}{f^{'} (x_{1})} (x - x_{1})$
- Rearrange the equation into whatever form the question requires

Examiner Tips and Tricks

Make sure you are confident with finding the equation of a straight line
- In particular when you know the gradient and one point on the line
- This is an essential skill for finding tangents and normals

Worked Example

The function $f (x)$ is defined by

$f (x) = 2 x^{4} + \frac{3}{x^{2}} x \neq 0$

a) Find an equation for the tangent to the curve $y = f (x)$ at the point where $x = 1$ , giving your answer in the form $y = m x + c$ .

Substitute $x = 1$ into $f (x)$ to find the $y$ -coordinate of the point

$f (1) = 2 {(1)}^{4} + \frac{3}{{(1)}^{2}} = 2 + 3 = 5$

So the point in question is $(1, 5)$

Now differentiate to find $f^{'} (x)$ , first using laws of indices to rewrite $\frac{3}{x^{2}}$ as $3 x^{- 2}$

$f (x) = 2 x^{4} + 3 x^{- 2}$

$\begin{array}{rcl} f^{'} (x) & = & 2 (4 x^{4 - 3}) + 3 (- 2 x^{- 2 - 1}) \\ = & 8 x^{3} - 6 x^{- 3} \\ = & 8 x^{3} - \frac{6}{x^{3}} \end{array}$

Substitute $x = 1$ into $f^{'} (x)$ to find the gradient of the tangent at the point

$f^{'} (1) = 8 {(1)}^{3} - \frac{6}{{(1)}^{3}} = 8 - 6 = 2$

Now use $y - y_{1} = m (x - x_{1})$ to find the equation of the line

Here $m = 2$ and $(x_{1}, y_{1}) = (1, 5)$

$\begin{array}{rcl} y - 5 & = & 2 (x - 1) \\ y - 5 & = & 2 x - 2 \\ y & = & 2 x + 3 \end{array}$

$y = 2 x + 3$

b) Find an equation for the normal to the curve $y = f (x)$ at the point where $x = 1$ , giving your answer in the form $a x + b y = c$ , where $a$ , $b$ and $c$ are integers.

The normal and tangent are perpendicular

So the gradient of the normal will be $- \frac{1}{f^{'} (1)}$

$- \frac{1}{f^{'} (1)} = - \frac{1}{2}$

Now use $y - y_{1} = m (x - x_{1})$ to find the equation of the line

Here $m = - \frac{1}{2}$ and $(x_{1}, y_{1}) = (1, 5)$

$y - 5 = - \frac{1}{2} (x - 1) y - 5 = - \frac{1}{2} x + \frac{1}{2}$

Multiply both sides by 2 to get rid of the fractions

Then rearrange into the required form

$\begin{array}{rcl} 2 y - 10 & = & - x + 1 \\ x + 2 y & = & 11 \end{array}$

$x + 2 y = 11$

Last updated: 20 October 2024

You've read 0 of your 10 free revision notes

Unlock more, it's free!

Join the 100,000+ Students that ❤️ Save My Exams

the (exam) results speak for themselves:

Did this page help you?

Previous:Stationary Points & Turning PointsNext:Rates of Change